By multiplying the number of days in a sothic, or julian year (365.25) by the number of days in a draconic year (346.62), and 3√3, then dividing by 20π and the number of days in a lunation (29.53059), we can obtain approximately the number of days in a lunar year. This approximate equation would also work with the tropical year of 365.242199 days instead of the Sothic year. Perhaps this is why we find the square root of 3 and pi in various measures at Giza. It may also be linked to the curious number 440, which is the number of royal Egyptian cubits generally assigned to the base side of the Great Pyramid. This 440 is often associated with the approximation of pi as 22/7, and indeed there is a pi ratio between the height and base of the Great Pyramid. But perhaps the number 440 is also linked to ratios between calendar periods, expressed within the dimensions of the pyramid.
A Sothic year is 365.25 days long, which corresponds almost exactly to the motion of Sirius in the 4th millenium BC, so it is the length of time Sirius takes to return to the same position in relation to the sun, as seen from earth. Sirius does not travel across the sky at the same rate that other stars do in terms of precession, so it has a special status, in that it offers a very stable standard by which to monitor other cycles, and was used as a basis for calendars across the ancient world, from Egypt to Mali, Mesopotamia to Mexico, New Zealand to China. The Sothic cycle marries two cycles, the Egyptian civil year of 365 days and the Sothic year, of 365.25 days. the sothic cycle is 1 461 Egyptian civil years of 365 days each, which is equivalent to 1,460 Julian years averaging 365.25 days each.
A draconic year, or eclipse year, is the time taken for the Sun (as seen from the Earth) to complete one revolution with respect to the same lunar node, the point where the Moon's orbit intersects the ecliptic, the path of the sun. Eclipses occur when the sun and moon are near these nodes. The average duration of the eclipse year is 346.6201days.
A lunar year is a period of 12 lunations, or full cycles of the phases of the Moon, as seen from Earth, of 29.53059 days, which gives a total of 354.36708 days. (In the Hebrew calendar it can also mean 13 lunations.)
If we take the period of a Sothic year as 365.25 days , and a draconic year as 346.6201 days, multiply them by 3√3, and divide by 20π and 29.53059, this last number being the average number of days in a lunation, and π being the ratio between a circle's circumference and diameter, we get 354.54703, which is very nearly the average number of days in a lunar year (about 4 hours and 19 minutes short). In the same way, we can obtain the approximate value of the Sothic year as 20π x 29.53059 / 3√3 x the number of days in a lunar year, divided by the number of days in a draconic year. And by the same token, the draconic year is the lunar year divided by the sothic year multiplied by 20π x 29.53059 / 3√3. Also, 28 / 10 000 is a good approximation of 3√3/ 20π x 29.53059. If there are 440 royal Egyptian cubits in the base side of the Great Pyramid (440 x 20.618181818 = 9072, and Flinders Petrie gives 9068.8 inches), there are 280 in its height. 280 x 20.618181818 = 5773.090909. Flinders Petrie's estimate for the height is 5776 inches.
Could any of these calculations have been used in the dimensions of ancient sites, such as Giza? If we take 20π / 3√3, omitting the 29.53059, this does seem to be compatible with several measures at Giza. For example, 20π / 3√3 multiplied by 3000 gives the perimeter of the Great Pyramid in inches. Or multiplied by 1500/2 gives the base side of the Great Pyramid, which can also be approximated by 9! / 40 inches.
20π / 3√3 multiplied by 29.53059 x 100 and by 9/11 gives the Giza rectangle width, that is the east-west dimension of the rectangle created by the top easternmost corner of the Great Pyramid and the bottom westernmost corner of the third pyramid. Or, the width of this rectangle can be understood as 2π / 9 x 29.53059² x 48 inches. And 20π / 3√3 x 29.53059 x 100 gives the length of this Giza rectangle. The Great Pyramid base side can simply be multiplied by 12 / 110 and 29.53059 to obtain the Giza rectangle width, and by 4/30 x 29.53059 or the Giza rectangle length. The Great Pyramid base can be understood as 5000 π / √3 inches. When this is multiplied by 9/11, the ratio between sides of the Giza rectagle, and then multiplied by 1 / 600, the average number of lunations per year is obtained: 12.3668138. And 365.242199 / 29.53059 = 12.368266.
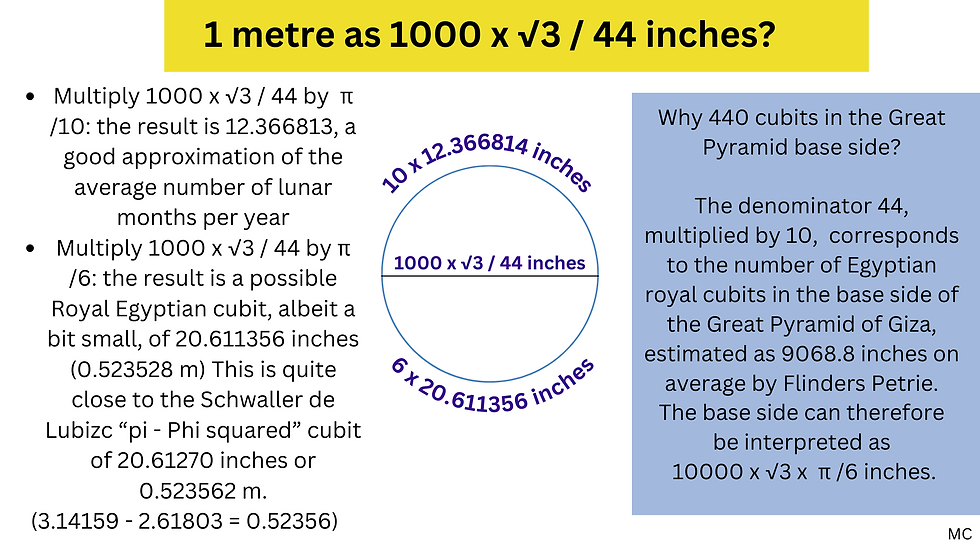
The average value of lunar months per year is also approximately 20π / 3√3 multiplied by 9 / 880, which gives 12.3668138. Or 1 000π x √3 /440. This can be multiplied by 10/π to give something which, in inches, is just under the length of a metre, at 39.36479 inches.
If the Great Pyramid's perimeter can be approximated by 9! / 10 inches (this is 1 x 2 x 3 x 4 x 5 x 6 x 7 x 8 x 9 / 10), the cubit that is often associated with this base is then 9! / 17.6 = 20.618181818 inches. The remen can be described as 9! / 12⁵ = 14.5833333 inches. Very close to the number produced by 9! / 10 inches or the perimeter is 20 000 π / √3 inches. Indeed, 9! x √3 / 10π is 20 006.6, very close to 20 000.
Dennis Payne has found that the line running from the centre of the Queen's pyramid, beside the Great Pyramid (the top one), to the Queen's pyramid beside the third pyramid (the bottom one) is 3850.34 feet, which is equivalent to 12096.1999 / π feet (see here). This means that a circle with a diameter equal to the diagonal of this rectangle, formed by these two queen's pyramids, has a circumference of 12096.1999 feet. This figure is almost exactly 2000 π / 3√3 feet, or 8000 π /√3.
David Kenworthy works with a value of 9216 inches, and this can be equated approximately to 8000 π x 11 / 30 inches. The large rectangle at Giza formed by the outermost corners of the great and third pyramids has a length, N-S, which can be approximately equated to 8000 π / √3 x 29.53059 / 12 = 35708.37 inches. And 8000 π / √3 x 5/2 works as the perimeter of the Great Pyramid in inches. 8000 π / √3 x 3 / (8 x 440) = 12.366814. This is close to the average number of lunar months per year, also equivalent to √3 x 1000 π / 440. The appearance of the number 440 is curious, as there are 440 Egyptian royal cubits in the base of the Great Pyramid.
If we take Flinders Petrie's estimate for the mean base side of the Great Pyramid, which is 9068.8 inches, and divide that into 440 parts, the result is 20.61090909. If we round the value up slightly to 9069 inches, the result is 20.611363636 inches. And if we take the base side as 9072 inches, the resulting cubit is 20.6181818 inches, which is the one most commonly associated with the exterior of the Great Pyramid, equivalent to 0.2 x 39.375 x 144/55 inches.
If we can think of the average number of lunations per year as 1000 π x √3 / 440, can we think of a cubit also as 10 000 π x √3 / (440 x 6) = 20.6113564 inches? Multiplying this value by 440 gives 9068.9968, which is the Great Pyramid base, which fits well with Flinders Petrie's estimate. The height of the Great Pyramid then becomes √3 x10 000 / 3 = 10 000 / √3 = 5773.5026 inches. The 440 links the ratio between the solar and lunar years to the 1000 π x √3 value that the Great Pyramid seems to embody, as its base side can be equated with 10 000 π x √3 / 6 inches. A cubit can also be understood as the solar year divided by the lunar year x 20 inches, but this value is slightly greater.
A cubit of 20.611356 inches is 0.52352844 modern metres, which is very close to the π - ((√5 + 3)/2) = 0.52355866 m (20.6125458 inches) value suggested by Schwaller de Lubicz. The large rectangle formed by the topmost east corner of the Great Pyramid and the bottom west corner of the third pyramid, is, according to Flinders Petrie, 29 227.2 inches wide E-W and 35 713.2 inches long N-S. This cubit of 20.611356 inches doesn't fit quite as well as the 20.6181818 inch cubit, as 48 x 29.53059 x 20.611356 = 29 215.944 inches, and this value multiplied by 11 / 9, the proportions of this rectangle, gives 35 708.376. As a value to link the solar year and lunar month, 1000 π x √3 / 440 is resonably accurate, to within just over an hour. This means that it is possible to use geometry to illustrate the relationship between the sun and the moon.
The geometry of the circle comes into play, to represent the ratio between the lunar month and the solar year, if we equate pi approximately with this ratio (12.36681) multiplied by 44 / (√3 x 100). For those who like to believe that the ancient Egyptians didn't know the ratio between a circle's diameter and circumference, this comes pretty close, being 3.141591676. It's interesting, also, that √3 x 1000 / 44 = 39.36479, which is very close to the value of a metre in inches. And this is why a circle with a diameter of 1 metre gives a circumerence, in inches, with a value close to the average number of lunations in a year. In this case, π x √3 x 100 / 44 = 12.3668. And this number multiplied by 440, the number of cubits in the Great Pyramid base side, divided by 1000 π, brings us back to √3, approximately.
The use of the square root of three together with pi could be a geometric reference to the ratios between the draconic, sothic and lunar years, and the lunar month. Indeed 100 π / (3 √3) x 12 x 29.53059 ² / ( 5 x 346.62) = 365.0647. The N-S length of the large Giza rectangle can be understood as 5 draconic years of 346.62 days, with inches representing days. If we divide this length, 35 713.2 inches, by 1000 √3, we obtain a cubit of 20.6190256 inches, which, multiplied by 48 x 29.53059, gives the width of this rectangle, to within less than an inch, according to Flinders Petries measure of 29 227.2 inches. The W-E width of this rectangle can be made to represent in the same way either 80 solar years of 365.242199 days in inches, or 48 lunar months of 29.53059 in royal cubits. The ratio between the lunar and draconic years is very close to 20 π x 29.53059 / 3√3. And while 1000 π x √3 / (6 x 44) produces a slightly smaller cubit in inches, at 20.611356 inches, this fits perfectly into the Great Pyramid's base side 440 times.
The diagram below is to show how one might begin with10 000 inches and through a series of simple calculations, arrive at the dimensions of many key features at Giza. The place to start is the yellow bow to the top left. In the first algorithm, with the yellow boxes, the number 10 001.4822 has been plugged in. While the number 10 000 works well, this slightly bigger number works even better, in matching the products of the sums in the algorithm with Flinders Petrie's dimensions. This could be because the Giza plans relies on an inch that is very slightly different to ours, so that 1.000148222 imperial inch is 1 Giza inch. This figure was arrived at simply by taking the Giza rectangle width (the width of the rectangle formed by the outermost corners of the Great and third pyramid), as given by Flinders Petrie, and multiplying by 3 / 32 π x 29.53059². It is by no means proven that the inch was used at Giza, perhaps it never can be. But this algorithm is food for thought as it begins with close to 10 000 inches. However, it is the ratios contained in the arrows that are most important, and they work whatever unit is being used. The number 1209.37881 has been found at Giza in several areas, by Dennis Payne.
In this second algorithm, below, the number 10 001.17441833 has been plugged in. This is 365.242199 / (12 x 29.53059) x 440 x √3 x 2 / π, so it is derived from accurate sun and moon cycle values.
In either case, we can think of 10 000 inches being put into the algorithm, but perhaps with a slight variation on our modern inch, as per the two numbers in the algorithms above, 1.000148222 and 1.000117441833 respectively. If we think of the cubit of 1000 π x √3 / (6 x 44) inches, in terms of this possible ancient inch, so multiplying it by 1.00017441833, the result is 20.614951 inches, which is slightly closer to what we find on the ground at Giza, be it the Great Pyramid's exterior, or the King's Chamber.