79. Plato's Perfect Number of Time
- M Campbell
- Sep 25, 2024
- 33 min read
Updated: Nov 15, 2024

In Timaeus, one of his most intriguing dialogues, Plato invites readers to think about the vast and ordered structure of the cosmos. A vivid picture of a universe governed by harmonious celestial movements is painted, part of a grand cosmic order. The reader is introduced to two primary cosmic cycles: the circle of the Same, representing the apparently fixed stars, and the circle of the Different, which maps the complex paths of the seven ancient ‘planets’, these being the Sun, the Moon, Mercury, Venus, Mars, Jupiter and Saturn, as seen from earth. Altogether, there are eight motions: the background of apparently fixed stars, which is the cycle of axial precession, and the heavenly bodies just mentioned. This grand cosmic machinery, Plato suggests, follows a precise rhythm, mirroring the perfection and intention of the universe itself.
Plato introduces the idea of the "perfect number of time" as "τέλειος ἀριθμός τοῦ χρόνου" (teleios arithmos tou chronou), or "the perfect number governing time." This number is part of a belief according to which time itself is governed by numerical order and cycles. The choice of the word "τέλειος" (teleios), meaning "complete" or "perfect," emphasises the philosophical idea that time follows a perfect, preordained structure, a reflection of the divine harmony of the cosmos. Perhaps this perfection or completeness is simply the consequence of being part of a divine structure in nature. It may also refer to a mathematically perfect number, such as 6 or 28, being the sum of its divisors, or perhaps to some other number, important in ancient tradition, such as 60, 216, 1 296, 108 or 4 320. This reflection on numbers, particularly "οἱ ἀριθμοί τελεώτατοι" in Laws and "τέλειος ἀριθμός τοῦ χρόνου" in Timaeus, reveals Plato’s conviction that the universe and time itself are governed by mathematics.
Plato is, however, deliberately obscure about certain aspects of these cosmic workings, and while he refers to a certain number which expresses the great cycle within which the eight lesser cycles operate, he does not say what it is. In the Timaeus, Plato mentions other numbers, indeed the first words of the dialogue are numbers, and numerical progressions such as 1, 2, 4, 8 and intervals such as 4/3 are freely given. The perfect or complete number of time is not, however, given. What could it be?
This article will first examine Plato’s depiction of celestial motion and the intricate system of planetary cycles and alignment, known as the perfect, or great year, before looking at some possible numbers which might fit the bill, based on astronomical cycles. Following this, we will explore possible resonances of this number at Giza.
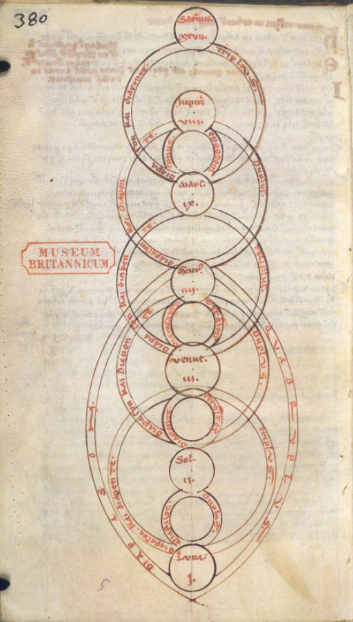
Reason + mathematics = order
In Timaeus, Plato describes the movement of the celestial bodies and the return of the planets to their original positions. There are two great circles: the outer one, called the circle of the Same, which represents the fixed stars, and the inner one, the circle of the Different, which is broken into seven parts, corresponding to the movements of the seven known planets. The circle of the Different is the movement of the stars, which we could equate with the Zodiac or the ecliptic. As the earth's axis is slightly tilted, it creates a wobble, so that over about 25 920 years, a full cycle is completed, known as axial precession, or the precession of the equinoxes. Together, these eight circles represent the celestial order. The outermost circle is the motion of the fixed stars (the Same). The inner circles represent the motions of the seven planets, including the Sun and Moon (the Different). In total, there are eight circles of motion: the fixed stars plus the seven planets known to antiquity. Plato's description in the Timaeus gives a detailed account of the ordered cosmos, with the stars and planets following their harmonious revolutions, reflecting the structure and motion of the universe.
Plato describes how time, heaven, and the seven planets came into being:
“Time, then, and the heaven came into being at the same instant in order that, having been created together, if ever there was to be a dissolution of them, they might be dissolved together. It was framed after the pattern of the eternal nature, that it might resemble this as far as was possible; for the pattern exists from eternity, and the created heaven has been, and is, and will be, in all time. Such was the mind and thought of God in the creation of time. The sun and moon and five other stars, which are called the planets, were created by him in order to distinguish and preserve the numbers of time; and when he had made-their several bodies, he placed them in the orbits in which the circle of the other was revolving-in seven orbits seven stars. First, there was the moon in the orbit nearest the earth, and next the sun, in the second orbit above the earth; then came the morning star and the star sacred to Hermes, moving in orbits which have an equal swiftness with the sun, but in an opposite direction; and this is the reason why the sun and Hermes and Lucifer overtake and are overtaken by each other.”(1)
The seven “stars” are the moon, the sun, Venus (referred to as the “morning star”, and Lucifer), Mercury (referred to as “the star sacred to Hermes”, or simply as Hermes, the Greek counterpart of Hermes being Mercury in the Roman tradition), and, not mentioned by name, Mars, Jupiter and Saturn. These “stars” are described in terms of their motion, within time.
“Now, when all the stars which were necessary to the creation of time had attained a motion suitable to them,-and had become living creatures having bodies fastened by vital chains, and learnt their appointed task, moving in the motion of the diverse, which is diagonal, and passes through and is governed by the motion of the same, they revolved, some in a larger and some in a lesser orbit-those which had the lesser orbit revolving faster, and those which had the larger more slowly. Now by reason of the motion of the same, those which revolved fastest appeared to be overtaken by those which moved slower although they really overtook them; for the motion of the same made them all turn in a spiral, and, because some went one way and some another, that which receded most slowly from the sphere of the same, which was the swiftest, appeared to follow it most nearly.”(2)
The sun is described as giving light to “the whole of heaven”, which enables animals on earth to see all the heavenly activity, learn arithmetic, and “participate in number”. Several cosmic cycles are briefly described: the rotation of earth on its own axis (day and night), the orbit of the moon around the earth (month), and the earth around the sun (year), though it is described as the sun around the earth, just as it is seen from the viewpoint of earth. Rather than describe the other planetary orbits, Plato simply comments that most people don’t know much about them.
“That there might be some visible measure of their relative swiftness and slowness as they proceeded in their eight courses, God lighted a fire, which we now call the sun, in the second from the earth of these orbits, that it might give light to the whole of heaven, and that the animals, as many as nature intended, might participate in number, learning arithmetic from the revolution of the same and the like. Thus then, and for this reason the night and the day were created, being the period of the one most intelligent revolution. And the month is accomplished when the moon has completed her orbit and overtaken the sun, and the year when the sun has completed his own orbit. Mankind, with hardly an exception, have not remarked the periods of the other stars, and they have no name for them, and do not measure them against one another by the help of number, and hence they can scarcely be said to know that their wanderings, being infinite in number and admirable for their variety, make up time.“(3)
The motion of the seven planets is added to the background motion of the stars. In total: eight motions, as seen from earth.
“And yet there is no difficulty in seeing that the perfect number of time fulfils the perfect year when all the eight revolutions, having their relative degrees of swiftness, are accomplished together and attain their completion at the same time, measured by the rotation of the same and equally moving. After this manner, and for these reasons, came into being such of the stars as in their heavenly progress received reversals of motion, to the end that the created heaven might imitate the eternal nature, and be as like as possible to the perfect and intelligible animal.” [Italics added] (4)
Translations differ. What Benjamin Jowett translates as “perfect number of time” can also be interpreted as “complete number of time”, for example, as below, in the translation by W.R.M. Lamb:
"Nevertheless, it is still quite possible to perceive that the complete number of Time fulfils the Complete Year when all the eight circuits, with their relative speeds, finish together and come to a head, when measured by the revolution of the Same and Similarly-moving."(5)
At the end of this "perfect number of Time", all the planets realign, bringing renewal. In this translation, the emphasis is on completion, as opposed to perfection, in the earlier quotation. In Timaeus, Plato also introduces the idea that the universe was created by a creator, called the Demiurge. This being does not create out of nothing, but rather organises pre-existing chaotic matter into an ordered cosmos. The Demiurge, driven by goodness and intelligence, aims to create the most perfect and harmonious universe possible. The Demiurge uses reason and mathematics to impose order on the chaotic material, and the cosmos is constructed as a living being, endowed with soul and intelligence. Plato emphasises that the cosmos is governed by order, proportion, and harmony, reflecting the rational structure imposed by the Demiurge. The cosmic order is deeply rooted in mathematical ratios and geometric forms. In Timaeus, Plato describes how the Demiurge crafts the universe using ideal mathematical forms like the Platonic solids, which are associated with the elements (earth, air, fire, water, and ether).
In the Republic, in the Myth of Er, Plato also refers to eight celestial motions, reflecting a concept of an ordered universe governed by precise mathematical proportions and geometrical harmony.
“The staff turned as a whole in a circle with the same movement, but within the whole as it revolved the seven inner circles revolved gently in the opposite direction to the whole, and of these seven the eighth moved most swiftly, [617b] and next and together with one another the seventh, sixth and fifth; and third in swiftness, as it appeared to them, moved the fourth with returns upon itself, and fourth the third and fifth the second. And the spindle turned on the knees of Necessity, and up above on each of the rims of the circles a Siren stood, borne around in its revolution and uttering one sound, one note, and from all the eight there was the concord of a single harmony. And there were another three [617c] who sat round about at equal intervals, each one on her throne, the Fates, daughters of Necessity, clad in white vestments with filleted heads, Lachesis, and Clotho, and Atropos, who sang in unison with the music of the Sirens, Lachesis singing the things that were, Clotho the things that are, and Atropos the things that are to be. And Clotho with the touch of her right hand helped to turn the outer circumference of the spindle, pausing from time to time. Atropos with her left hand in like manner helped to turn the inner circles, and Lachesis [617d] alternately with either hand lent a hand to each.”(6)
The idea of circles is important within this understanding of the cosmos. The Antikythera mechanism, an analogue computer from ancient Greece, clearly shows that at least one Greek astronomer understood that the paths of some of these heavenly bodies was in fact best described as ellipses, rather than as circles. Plato doesn’t mention ellipses, but instead emphasises the importance of the circle, perhaps on the basis that it is a geometric shape that lends itself well to being described as symmetrical, perfect even.
“The opinion, my friends, that the Sun and Moon and the rest of the stars “wander” is not correct; the truth is precisely the opposite: each of them always travels in a circle one and the same path,—not many paths, although it appears to move along many paths; and the quickest of the stars is wrongly opined to be the slowest, and vice versa.“
Indeed, these circles are key to the whole motion of heaven for Plato, the inner circle corresponding to the planets (as opposed to the background of the stars) being divided into seven parts according to particular intervals, and moving at particular speeds. Various numbers are mentioned in the process of creation, for example mathematical progressions such as a series of powers of 2, and another of powers of 3, intervals of 3:2, 4:3, 9:8, the ratio 246 / 243, and of course these 8 circles,
“Next, He split all this that He had put together into two parts lengthwise; and then He laid the twain one against the other, the middle of one to the middle of the other, like a great cross; [36c] and bent either of them into a circle, and join them, each to itself and also to the other, at a point opposite to where they had first been laid together. And He compassed them about with the motion that revolves in the same spot continually, and He made the one circle outer and the other inner. And the outer motion He ordained to be the Motion of the Same, and the inner motion the Motion of the Other. And He made the Motion of the Same to be toward the right along the side, and the Motion of the Other to be toward the left along the diagonal; and He gave the sovranty. [36d] to the Revolution of the Same and of the Uniform. For this alone He suffered to remain uncloven, whereas He split the inner Revolution in six places into seven unequal circles, according to each of the intervals of the double and triple intervals, three double and three triple. These two circles then He appointed to go in contrary directions; and of the seven circles into which He split the inner circle, He appointed three to revolve at an equal speed, the other four to go at speeds equal neither with each other nor with the speed of the aforesaid three, yet moving at speeds the ratios of which one to another are those of natural integers.”
As Cornford remarks, Plato gives a very detailed account of the motions of the heavens for a discussion on creation.
"The conclusion is that the Laws (certainly) and the Epinomis (quite possibly and, I should say, probably) are perfectly consistent with the theory of the Timaeus , which ascribes a compound motion to the seven planets. The conception is fundamental in the system of Eudoxus, who was working at the Academy before the Timaeus was written and who died before Plato. It is equally fundamental in Aristotle's adaptation of Eudoxus’ system of spheres. The system must have been known to Plato, and the probability is that he incorporated in the Timaeus as much of it as he could accept, consistently with his belief that the proper motion of each planet keeps to a circular track. It should not be forgotten that the Timaeus is a myth of creation, not a treatise on astronomy. The surprising thing is that Plato should have found room for so many details in his broad picture of rational design in the cosmos, not that he should have simplified by omitting subtleties which would contribute nothing to his main purpose and which might be superseded at any time, as indeed they were very soon afterwards." (2)
The order that has been imposed on the cosmos by the Demiurge is through number, and geometry. In Republic, Plato suggests the divine is linked to a mystery perfect number. This is opposed to another number, also very enigmatic, which is linked to mortals, not the divine. Many commentators have tried to find a number which fits the description given by Plato:
“ [546b] the men you have bred to be your rulers will not for all their wisdom ascertain by reasoning combined with sensation, but they will escape them, and there will be a time when they will beget children out of season. Now for divine begettings there is a period comprehended by a perfect number, and for mortal by the first in which augmentations dominating and dominated when they have attained to three distances and four limits of the assimilating and the dissimilating, the waxing and the waning, render all things conversable and commensurable [546c] with one another, whereof a basal four-thirds wedded to the pempad yields two harmonies at the third augmentation, the one the product of equal factors taken one hundred times, the other of equal length one way but oblong,—one dimension of a hundred numbers determined by the rational diameters of the pempad lacking one in each case, or of the irrational lacking two; the other dimension of a hundred cubes of the triad. And this entire geometrical number is determinative of this thing, of better and inferior births.”
There is no consensus on what this period comprehended by a perfect number is, nor if it is the same as the perfect number of time in Timaeus, but it is possible that, in Republic, Plato is alluding to the same perfect number which qualifies, or quantifies, the divine aspect of creation and time in both dialogues.
2. What could the “perfect number of time” be?
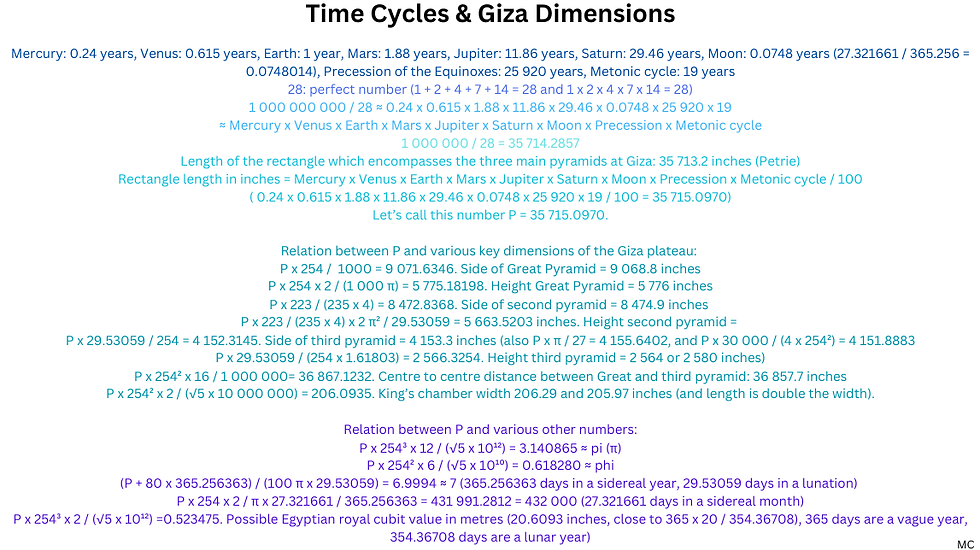
One might hypothesise about Plato’s 'perfect number of time' by exploring numerical cycles that align with ancient cosmological thought, especially those favouring multiples of 6, 9, and 12. The time it takes for the planets (Mercury, Venus, Mars, Jupiter and Saturn) to orbit the sun, as well as the orbit of the moon round the earth, and the precession of the equinoxes, and perhaps other cycles also, might be considered. These are the orbital periods defined in terms of earth years:
Mercury: 0.24 years
Venus: 0.615 years
Earth: 1 year
Mars: 1.88 years
Jupiter: 11.86 years
Saturn: 29.46 years
Moon: 0.0748 years (27.321661 / 365.256 = 0.0748014)
Precession of the Equinoxes: 25 920 years
The value of 0.0748 years for the Moon is derived by considering the synodic month, the time it takes for the Moon to return to the same position relative to the Sun as seen from Earth, and based on a sidereal year of 365.256 days. Below are some possible candidates for a perfect number of time, combining the cycles of the seven ‘planets’ and precession:
All these numbers multiplied together are 187 974.1949 years. This doesn’t seem to be a very mathematically interesting number however.
In Àryabhatiya, the Indian astronomer Āryabhata (c. 499 CE) offers a similar perspective to Plato’s on cosmic cycles. He describes the solar year as the basic unit, equating twelve thousand of these years to a planetary yuga. Thirty such yugas comprise a ‘year of the Fathers,’ while twelve of the Fathers’ years correspond to a ‘year of the gods.’ Finally, twelve thousand godly years complete a planetary yuga, a full cycle of cosmic alignment.
“Thirty of these make a year of the Fathers. Twelve years of the Fathers make a year of the gods. Twelve thousand years of the gods make a yuga of all the planets.”
A Yuga of all the planets in years: 12 000 x 30 x 12 x 12 000 = 51 840 000 000.
Might 51 840 000 000 be Plato’s perfect number of time? Dividing this by the planetary cycles and precessional cycle gives 275 782.5351 years. This doesn’t seem to fit with multiples of 3, 6, 12 or any other cycle. The same goes for when the number 19 is brought in too, for the Metonic cycle.
In the ancient Indian tradition, a yuga is 43 200 000 years. Could the number 43 200 000 be the number, if a way could be arrived at to connect various astronomical cycle numbers to obtain it? If we multiply the planetary cycles in earth years, together with the precessional cycle and Metonic cycle in years (19), and the number of sidereal months in a Metonic cycle (254), and 2 sidereal months in days, and then divide the result by a a tropical year in days (365.242199) and pi, the result is close to 43 200 000.
0.24 x 0.615 x 1.88 x 11.86 x 29.46 x 0.0748 x 25 920 x 19 x 254 x 2 x 27.321661 / (365.242199 x π) = 43 200 803.40 ≈ 43 200 000
However, while the elusive number is more or less achieved, there is a mix cycles expressed in days and years, so while the numbers work, the units of time don't necessarily. So a unit of 4 320 000 years is still a mystery, unless taken as purely symbolic. Despite attempts to correlate planetary revolutions, lunar cycles, and axial precession within a 4,320,000-year yuga, an exact alignment remains elusive.
If all the planetary cycles and precession are multiplied together, but the moon's orbit is omitted, the result is 2 513 023.9957 years. This is close to 800 000 π years, which is an interesting result, as it features the important number 8, which is the total number of circles created by the demiurge (seven for the ‘planets’ and one for the background motion of the stars), as well as pi, which implies the geometry of the circle. One might be forgiven for thinking there was some sort of divine geometry at play here. However, the downside is that it doesn’t include the moon. Is 800 000 π the perfect number of time?
Another possibility is to divide an earth year by the other cycles (planets and precession): 1 / (0.24 x 0.615 x 1.88 x 11.86 x 29.46 x 0.0748 x 25 920). The result is close to the fraction 158 / 29 700 000 = 0.000005319 8653198, but offers no connection to a sexagesimal system, or the important ancient numbers such as 4 320 or 25 920. Still, could 158 / 29 700 000 be the number of time?
Another interesting possibility is to multiply the cycles of precession, Mercury, Venus, Mars, Jupiter, Saturn together, and dividing by a moon cycle as 0.0748, which gives a result close to 33 600 000. This number can be interpreted as 28 x 1 200 000, or 1296 x 25 920, or 6 x 7 x 8 x 100 000. The results are important ancient numbers: 28 is the number of days in a sidereal month, rounded up from 27.321661, 12 is the number of months in a year, 1 296 is 6⁴, and 25 920 is the number of years in a precessional cycle. 6, 7 and 8 are also important.
25 920 x 0.24 x 0.615 x 1.88 x 11.86 x 29.46 / 0.0748 = 33 596 577.4823 ≈ 33 600 000
Is 33 600 000 the perfect number of time? This would suggest that the role of the moon may have been to harmonise other celestial cycles, producing a numerical total divisible by the numerologically significant values of 6 and 9.
33 596 577.4833 = 1 296 x 25 923.285 ≈ 1 296 x 25 920
= 28 x 1 199 877.7672 ≈ 28 x 1 200 000
= 20 / 9 x 108 x 108 x 1296.16425 ≈ 20 / 9 x 108 x 108 x 1 296
= 4 320 x 108 x 72.007775 ≈ 4 320 x 108 x 72
≈ 6 x 7 x 8 x 100 000
≈ 43 200 000 x 7 / 9
7. The number 1 296 is especially interesting in this context, as many researchers, over the centuries, have settled for 1 296 as the best candidate for Plato’s number, from the Republic. Indeed, if we omit the precessional cycle, and simply multiply the planetary cycles together and divide by the moon cycle, the result is 1 296.1643, very close to 1 296, or 6⁴. Could this be the perfect number of time? 6 is a perfect number, and here it has been multiplied by itself three times.
8. Since a precessional cycle is 25 920 years, multiplying the planetary cycles together and dividing by the moon and precession gives 1/20.
0.24 x 0.615 x 1.88 x 11.86 x 29.46 / (0.0748 x 25 920) x 20 = 1.0001267
0.24 x 0.615 x 1.88 x 11.86 x 29.46 / (0.0748 x 25 920) = 1 / 20
This is a very nice numerical coincidence, but is 1/20, or even 20, Plato’s perfect number of time?
9. Another possibility is to start with 100 000 000, and divide this by all seven planetary cycles, and precession, and also by a Metonic cycle, of 19 years. The result is almost exactly 28.
100 000 000 / (0.24 x 0.615 x 1.88 x 11.86 x 29.46 x 0.0748 x 25 920 x 19) = 27.999364 ≈ 28
This is a very pleasing result because 28 is a perfect number. However, this result includes an astronomical cycle Plato doesn’t mention, namely the Metonic, a key ancient astronomical cycle. After a Metonic cycle, lunar phases closely align with the solar year, 235 synodic months being roughly equal to 19 solar years. As this cycle is really only just a combination of the earth’s, moon’s and sun’s (apparent) cycle, that is, of the moon’s synodic, and sidereal, months and the earth’s yearly course around the sun, it does make sense to include the Metonic cycle, even though Plato doesn't mention it. As 28 is a perfect number, literally, is it the best contender for Plato’s perfect number of time? Although Plato does not explicitly mention 28 in his work, he may have viewed it as significant due to its ties to Pythagorean thought, where it was classified as a "perfect number." The Pythagoreans believed perfect numbers like 28 embodied harmony and completeness, resonating with cosmic order. Given that 28 was already known as the approximate length of the lunar month, a critical element for calendars and religious ceremonies, its significance would not have been lost on Plato. The number 28 can be connected to some of the other possible numbers of time listed above: 5 184 x 7 / 1 296 = 28. Another intriguing aspect of 28, is that 1 / 28 can be translated geometrically to a ratio between pi and the square root of three, to within a small margin of error.
2π / (3 x √3) x 29.53059 x 1 000 = 35 708.3769 (the number of days in a lunation is 29.53059)
1 000 000 / 28 = 35 714.2857
0.24 x 0.615 x 1.88 x 11.86 x 29.46 x 25 920 x 0.0748 x 19 / 100 = 35 715.0970 In addition to the 28 day month, and the various cycles mentioned earlier being linked to 100 000 000 / 28, there is a solar cycle of 28 years. This is the time it takes for the days of the week to repeat on the same dates, in the Gregorian calendar, or any 365 day calendar system which features weeks of 7 days. The 28-year solar cycle arises from the way the Julian calendar handles leap years and days of the week: the same date advances by one day of the week each year (e.g., January 1 advances from a Monday to a Tuesday in the following year). Every four years, a leap year adds an extra day, which shifts the weekday by two days (e.g., from Monday to Wednesday). Because of this shifting pattern, it takes 28 years for the same date to align with the same day of the week again. This is the length of time it takes for the pattern of leap years and weekdays to repeat in the Julian calendar.
This 28 year cycle can be combined with the Metonic cycle of 19 years, so that the lunar phases will occur on the same calendar dates, and the same calendar date will fall on the same day of the week. Multiplying the two (19 × 28) yields a 532-year cycle, which is when both lunar phases and the weekdays of the Julian calendar realign. Thus, after 532 years, the full sequence of Easter dates repeats identically, Easter being a movable feast, dependent on both the sun and the moon. In 1582, the Gregorian reform corrected the drift of the equinox in the Julian calendar, which was essential for aligning the date of Easter with the spring equinox. The Julian calendar itself had inaccuracies that caused the spring equinox to drift slowly forward over the centuries. The Gregorian reform adjusted for this by skipping certain leap years. So the 532 year cycle only applies to the Julian calendar, with weeks of 7 days and a year of 365 days plus a leap year every four years. While there is no clear evidence that the 532 year Easter cycle was directly derived from ancient systems in Egypt, Babylon, or other civilisations, the concept of combining lunar and solar cycles was certainly a common practice. Ancient cultures often aimed to reconcile the lunar and solar calendars over longer periods, which is conceptually similar to the 532 year cycle in the Christian tradition. The seven day week is very old, and was used in Ancient India and Babylon, among other places. The presence of a seven day week is crucial for the 532 year cycle to work, as it ensures the periodic repetition of Easter Sunday and other significant days on the same days of the week.
It’s possible therefore to look again at the equation seen earlier, with 100 000 000, and divide this by all seven planetary cycles, and precession, and also by a Metonic cycle, of 19 years, the result being almost exactly 28, and think of 28 as a number of years. This is, as we’ve just seen, the cycle after which days of the week repeat on the same dates, on condition of an unbroken period of 365 day years, with a leap day every 4 years, and no other leap days.
100 000 000 / (0.24 x 0.615 x 1.88 x 11.86 x 29.46 x 0.0748 x 25 920 x 19) = 27.999364 ≈ 28
This can be modified to:
100 000 000 / (0.24 x 0.615 x 1.88 x 11.86 x 29.46 x 0.0748 x 25 920) = 27.999364 x 19 ≈ 28 x 19
28 x 19 = 532
There are 532 years in the periodic repetition of Easter Sunday and other significant days on the same days of the week. So a cycle of 532 years (the 28 and 19 year cycles combined) multiplied by the cycles of the seven ancient ‘planets’ and precession is 100 002 271.67419, close to 100 000 000.
In exploring Plato’s "perfect number of time," these calculations reveal intriguing alignments with prominent ancient values and cycles. It is up to the reader to decide which, if any could fit the description of the perfect or complete number of time governing the cosmos. Although definitive interpretation remains speculative, these findings invite further reflection on the role of "teleios arithmos tou chronou" as a philosophical symbol of cosmic unity, where divine intention and mathematical precision converge.
3. Giza
“There still remain, for the freeborn, three branches of learning: of these the first is reckoning and arithmetic; the second is the art of measuring length and surface and solid; the third deals with the course of the stars, and how they naturally travel in relation to one another. [818a] “
These three branches of learning, arithmetic, measure, and astronomy, may all be connected both in Plato's philosophy, and in the design of the Great Pyramid of Egypt, and it's accompanying pyramids. Perhaps they may be combined in other places from the ancient world too. As with Plato, in both Indian and Chinese cosmology, celestial bodies and their movements were tied to earthly events, morality, and cycles of time. Plato’s concept of eight revolutions governing the cosmos has parallels in Indian and Chinese systems, though expressed differently: nine celestial bodies in Indian thought, and five planets plus the Sun and Moon in Chinese thought. These traditions share a belief in cyclical time and a cosmic order that governs both the heavens and human life, influencing moral and political structures, and it is likely that other parts of the world held similar beliefs. The movements of celestial bodies were seen as fundamental to understanding the world and humanity's place within it, connecting Plato’s perfect cosmic harmony to the Indian Rita and the Chinese Dao. It is likely that many ancient cultures held similar views, and in particular, ancient Egypt.
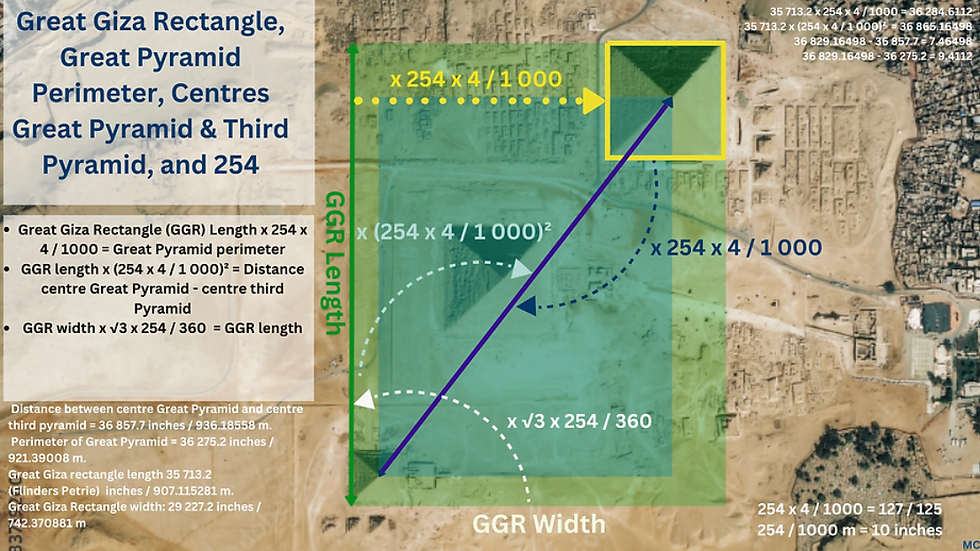
One link that is worth exploring is between the last suggestion for a perfect number of time, and the geometry and size of the Giza plateau, in Egypt. In the late 19th century, sir William Matthew Flinders Petrie measured the site extensively and accurately. He gave the north-south length of the rectangle formed by the northernmost side of the Great Pyramid and the southernmost side of the third pyramid as 35 713.2 inches. Several independent researchers have shown that in many ancient sites, from ancient Egypt to Megalithic Britain, an inch represents a day. Perhaps, in this case, an inch may also represent a year: if we start with the number 12, and divide it by all the orbital periods used earlier, and precession, and then multiply it by 10¹¹, the result is 35 717.9240, just a few inches more than the length as given by Petrie, 35 713.2 inches.
The length in inches is remarkably close to 1 000 000 / 28 = 35 714.2857 inches. And 1 000 000 / (2976.4937 x 12) = 27.9971, almost 28. Another way of interpreting this is to read the length in English feet. With Petrie's value, this is 2 976.1 feet. And the orbit of the earth as 1 year, divided by all the other orbits listed, and precession, is 2 976.4937.
The Great Pyramid of Giza, measuring approximately 280 Egyptian royal cubits in height (where 1 cubit is 20.62857 inches or 0.5239657 meters), might imply a connection between its dimensions and the number 28. This number is associated with the lunar month, as the sidereal month is just over 27 days and rounds up to 28. Moreover, 28 divides easily into four weeks of seven days, a structure central to ancient timekeeping. Some ancient cultures also divided the Zodiac into 27 or 28 segments, known as lunar mansions, which parallel divisions of the cubit rod and underscore the lunar significance of 28. Beyond astronomy, the number 28, like the number 6, is a "perfect number," meaning it equals the sum of its divisors (1, 2, 4, 7, 14). This fact deepens its symbolic role in ancient numerology, where it may have represented cosmic harmony. The fraction 1 / 28 holds additional interest when applied to astronomical cycles. For instance, the expression 2 π x 29.53059 / (3000 x √3), with 29.53059 representing the lunation period (number of days in a lunar month), suggests further astronomical connections when these cycles are interpreted through irrational numbers. Similarly, calculating a yuga of 4,320,000 years multiplied by 3 x √3 / (200 π) approximates the Giza rectangle’s length in inches as 35,726.1124, a value close to the measurement given by Petrie at 35,713.2 inches, and intriguingly near 1,000,000 / 28 ≈ 35 714.2857 inches. Interestingly, when dividing a yuga of 4,320,000 years by each planet’s orbital period, lunar cycles, precession, and the Metonic cycle of 19 years, the result approximates 1/28, or 2 π x 29.53059 / (3 √3) closely:
4 320 000 / (0.24 x 0.615 x 1.88 x 11.86 x 29.46 x 0.0748 x 25920 x 19) ≈ 2 π x 29.53059 / (3 √3)
Thus, dividing 100,000,000 earth years by these same cycles, the result nears exactly 28:
1 000 000 / (0.24 x 0.615 x 1.88 x 11.86 x 29.46 x 0.0748 x 25 920 x 19) = 27.999364
Interestingly, the length of the Giza rectangle, approximately 1 000 000 / 28 inches, aligns with the cycle of all known planetary orbits, sidereal months, precession, and the Metonic cycle in earth years. The width of this same rectangle, coincidentally, also approximates 80 years in days, further suggesting that Giza’s dimensions may subtly encode ancient cycles of time. The number 28 is not just about the approximate number of days in a lunar cycle, but also about uniting the cycles of the seven "planets" of the ancient world. This might give further support to the reason why dividing a cubit ruler, or the zodiac, into 28 parts, may have been so important, and by extension, dividing by 7 more generally, as 28 is 4 x 7. The Great Giza Rectangle holds remarkable connections to various astronomical cycles and dimensions of the pyramids at Giza, reflecting a profound understanding of planetary orbits, lunar cycles, and geometric proportions. The width of the rectangle, measuring 29,227.2 inches, corresponds closely to ten octaeteris cycles, each cycle representing a period of eight solar years, during which the phases of the moon return to the same day of the year. These ten cycles also align with ten Venusian cycles, as Venus completes its orbit around the sun in eight Earth years. In terms of days, 80 solar years (or ten octaeteris cycles) account for 29,220 days, a value almost identical to the width of the rectangle in inches. This suggests a symbolic or numerical link between the width of the rectangle and these lunar and Venusian cycles.
The length of the rectangle, approximately 35,713.2 inches, encodes a more complex relationship to planetary orbits, precession, and lunar cycles. When we multiply the orbital periods of Mercury (0.24 years), Venus (0.615 years), Earth (1 year), Mars (1.88 years), Jupiter (11.86 years), Saturn (29.46 years), the moon (0.0748 years), the precession of the equinoxes (25,920 years), and the Metonic cycle (19 years), then divide the result by 100, we arrive at a value of 35,715.0970, a value which is very close to the measured length of the rectangle in inches. This demonstrates that the rectangle’s length appears to have been designed to reflect these astronomical cycles in Earth years, reinforcing the notion that ancient builders possessed significant knowledge of the cosmos.
The symbolic value of 28, as evidenced in architectural measurements, astronomical cycles, and mathematical constants, may indeed align with the idea of a “perfect number of time” in ancient thought. These findings suggest that 28, as a mathematical and symbolic value, potentially served as an implicit yet powerful emblem of cosmic order, mirroring Plato’s vision of a universe bound by divine mathematics and universal harmony.
The measurements of the pyramids also align with mathematical relationships derived from the rectangle. For example, dividing the sum of the rectangle’s length and width by 700π results in 29.53024, a close approximation to the duration of a lunation (29.53059 days). Similarly, the third pyramid’s side length (4,153.3 inches) is nearly identical to the result of multiplying the rectangle’s length by π and dividing by 27 (4,155.4195). These correspondences between the rectangle’s dimensions and the pyramids’ architecture underscore a deliberate integration of cosmic cycles into the design of the Giza complex.
The side of the Great Pyramid (9 068.8 inches) can be expressed as 0.24 x 0.615 x 1.88 x 11.86 x 29.46 x 0.0748 x 25 920 x 19 x 254 x / 100 000 = 9 071.6346 inches. The height of the Great Pyramid (5,776 inches) is simply the side x 2 / π. The value of the height in inches as directly linked to another astronomical calculation further supports the idea that the length of the great rectangle is also derived from astronomical cycles. And the height as divided into 280 Egyptian royal cubits supports the idea of the length of the rectangle being a division by 28 also.

Just as the path of the sun along the zodiac was divided into 28 parts, here the north-south span of the site, from the north side of the Great Pyramid to the south side of the third pyramid, seems to represent a division by 28 also, in this case, of a million inches by 28. An Egyptian royal cubit is also divided into 28 parts, or 7 x 4.
Why not simply make the rectangle 28 000 inches long? Possibly because the Giza design is based on a dynamic system, in which various elements are meant to be added, subtracted, divided or multiplied by each other, or by key numbers. Also, there fraction 1/28, has other interesting connections to astronomical cycles, when these cycles are seen as numbers of days, and in conjunction with irrational numbers.

The distance between the centres of the Great and third pyramids can be arrived at by multiplying the side of the Great Pyramid by 254 x 16 / 1 000 = 36 867.1232 (Petrie gives 36 857.7 inches)
These precise measurements suggest a deep understanding of mathematical and astronomical principles that guided the construction of the Giza complex, linking the architecture of the pyramids to the natural cycles of the heavens. They also show a possible connection to a perfect number, 28, which could be the number Plato had in mind when he wrote about a perfect number of time.
The Great Giza Rectangle holds remarkable connections to various astronomical cycles and dimensions of the pyramids at Giza, reflecting a profound understanding of planetary orbits, lunar cycles, and geometric proportions. The width of the rectangle, measuring 29 227.2 inches, corresponds closely to ten octaeteris cycles, each cycle representing a period of eight solar years, during which the phases of the moon return to the same day of the year. These ten cycles also align with ten Venusian cycles, as Venus completes its orbit around the sun in eight Earth years. In terms of days, 80 solar years (or ten octaeteris cycles) account for 29 220 days, a value almost identical to the width of the rectangle in inches. This suggests a symbolic or numerical link between the width of the rectangle and these lunar and Venusian cycles.
The length of the rectangle, approximately 35 713.2 inches, encodes a more complex relationship to planetary orbits, precession, and lunar cycles. When we multiply the orbital periods of Mercury (0.24 years), Venus (0.615 years), Earth (1 year), Mars (1.88 years), Jupiter (11.86 years), Saturn (29.46 years), the moon (0.0748 years), the precession of the equinoxes (25 920 years), and the Metonic cycle (19 years), then divide the result by 100, we arrive at a value of 35 715.0970, a value which is very close to the measured length of the rectangle in inches. This demonstrates that the rectangle’s length appears to have been designed to reflect these astronomical cycles in Earth years, reinforcing the notion that ancient builders possessed significant knowledge of the cosmos.
The measurements of the pyramids also align with mathematical relationships derived from the rectangle. For example, dividing the sum of the rectangle’s length and width by 700π results in 29.53024, a close approximation to the duration of a lunation (29.53059 days). Similarly, the third pyramid’s side length (4 153.3 inches) is nearly identical to the result of multiplying the rectangle’s length by π and dividing by 27 (4 155.4195). These correspondences between the rectangle’s dimensions and the pyramids’ architecture underscore a deliberate integration of cosmic cycles into the design of the Giza complex.
The height of the Great Pyramid (5 775 inches) and the side lengths of the second and third pyramids also exhibit correlations with the rectangle's dimensions when adjusted by ratios involving the lunar cycle, the number 254, and other constants. Even the distances between the centres of the pyramids, such as the east-west and north-south measurements between the Great Pyramid and the third pyramid, demonstrate proportional relationships to the rectangle’s length when calculated using astronomical factors such as the Metonic cycle and the precession of the equinoxes.



These precise measurements suggest a deep understanding of mathematical and astronomical principles that guided the construction of the Giza complex, linking the architecture of the pyramids to the natural cycles of the heavens.
Conclusion
The search for Plato’s "perfect number of time" reveals interesting patterns in ancient cosmological thought, where the cycles of celestial bodies reflect a deeper order and harmony in the universe. By multiplying planetary orbits, precession, and the moon’s cycle, we arrive at values that suggest a connection between cosmic periods and mathematical precision.
The exploration of 28 as a key number in both astronomical and geometrical contexts provides compelling evidence for its importance in ancient cosmological systems. The close numerical connection between the length of the Giza rectangle, the lunation cycle, and cosmic proportions supports the idea that the number 28 could indeed represent Plato's perfect number of time. Not only is 28 a perfect number in its own right, but it also unifies the celestial cycles through its relationship with lunar and planetary orbits, particularly when factoring in the Metonic cycle. This integration of planetary and lunar cycles suggests a harmony that may have influenced Plato's thinking, even if indirectly. By applying the relationships between planetary revolutions, precession, and lunar cycles to the concept of a Yuga or a Great Year, we see a striking convergence of ancient time-keeping systems across cultures.
In this sense, Plato's perfect number of time could symbolise not just a mathematical culmination but also a deeper understanding of cosmic unity, where numbers, geometry, and time cycles all reflect the same underlying order. The inclusion of the Metonic cycle, which reconciles the solar year with the lunar months, provides further weight to the argument that these ancient systems sought to capture the entirety of celestial motion in symbolic, yet mathematically profound terms. This connection may serve as a bridge between the cycles of the heavens, the divisions of space, and the lived experience of time, highlighting a shared cosmological vision across ancient civilisations.
Summary of Possible "Perfect Numbers of Time":
Product of all cycles (187,974.1949 years): Multiplying all planetary cycles (Mercury through Saturn), the moon’s synodic month, and the precession cycle yields this total. However, it doesn’t reveal a strong connection to ancient significant numbers or numerical interest.
Āryabhata’s planetary yuga (51,840,000,000 years): Using the yuga calculations from Indian cosmology as a basis, dividing this yuga by planetary and precessional cycles results in 275,782.5351 years—an unaligned outcome with no apparent multiples of 3, 6, 9, or 12.
Indian yuga (43,200,000 years): Approximated by combining planetary cycles, precession, the Metonic cycle, and sidereal months. While the calculation nearly matches, it involves mixed units (years and days), making the connection uncertain beyond symbolism.
Without the moon’s orbit (800,000π years, or 2,513,024 years): Excluding the moon cycle and multiplying the other planetary and precessional cycles results in a number close to 800,000π. This intriguing total suggests divine geometry with π and the number 8, but omits the moon’s role.
Inverse of combined planetary and precessional cycles (158/29,700,000 ≈ 0.00000532): Dividing an earth year by all planetary and precessional cycles gives a fraction close to 158/29,700,000. This result lacks connections to known ancient numerological systems or significant numbers like 4,320 or 25,920.
Product of precession, Mercury, Venus, Mars, Jupiter, Saturn, divided by moon cycle (33,600,000 years): This figure links closely with ancient numbers (e.g., 1,296, 28, and 25,920) and aligns with lunar symbolism, suggesting a cosmological harmonisation. This total is divisible by 6 and 9, numerologically significant values.
1,296 (6⁴): Omitting precession and dividing the product of planetary cycles by the moon cycle yields a number close to 1,296. Since 6 is a perfect number, 1,296 (or 6⁴) resonates with Plato’s symbolic values and could represent divine order.
1/20 or 20 years: By dividing planetary cycles by the moon and precessional cycle, a value close to 1/20 arises. This fractional alignment, while a striking coincidence, does not directly connect with Plato’s specified numbers.
28 (perfect number): Starting from a base of 1,000,000,000 and dividing by all planetary, precessional, and Metonic cycles yields nearly 28, a perfect number with connections to lunar cycles. This outcome aligns well with ancient cosmology, though Plato does not directly reference the Metonic cycle.
The search for Plato’s perfect number of time uncovers intriguing patterns in ancient cosmological thought, where celestial cycles appear to reflect an underlying mathematical order. By multiplying planetary orbits, precession, and lunar cycles, we derive values that might symbolise cosmic timeframes, with results such as 187,974 years or approximately 2,513,024 years (close to 800,000 π years). These figures suggest grand cycles governing celestial motion.
The number 28, appearing frequently in both geometrical and astronomical contexts, provides compelling evidence for its importance in ancient systems. Its relationship to the lunation cycle, as well as the dimensions of the Giza rectangle, aligns it with cosmic cycles, reinforcing the possibility that Plato’s "perfect number of time" might be linked to this number. Not only is 28 a mathematically "perfect" number, but it also harmonises celestial cycles through its association with lunar and planetary orbits, especially when including the Metonic cycle. This integration of planetary and lunar periodicity demonstrates a harmony that may have influenced Plato's cosmological philosophy. By extending the relationships between planetary revolutions, precession, and lunar cycles to the concepts of the Yuga or the Great Year, we find a convergence among ancient timekeeping systems across cultures.
Ultimately, while Plato’s exact "perfect number of time" remains open to interpretation, the broader view endures: the universe’s mathematical structure reflects an intrinsic order, a harmonious design that invites both contemplation and wonder. Plato clearly believed that human creations, from the yearly calendar, to human births, from cities to the laws that govern them, should be designed and take place according to the same system the demiurge worked within, the system which gave rise to the motion of the stars and the planets. It is a profoundly mathematical and astronomical view. This is the ideal according to which Plato tells us we should live our lives by. Whatever the perfect number of time is, it makes sense to look for expressions of numbers associated with the movements of the moon, of the sun, and of the planets and stars in ancient architecture.
Bibliography
Bailly, Jean-Sylvain, 1775, History of Ancient Astronomy, Paris, Histoire de l'astronomie ancienne, depuis son origine jusqu'à l'établissement de l'École d'Alexandrie - Google Play Books
Diès, Auguste, 1940, “Le nombre de Platon. Essai d’exégèse et d’histoire.” Mémoires présentés par divers savants étrangers à l’Académie, Année 1940. Available at: https://www.persee.fr/doc/mesav_0398-3587_1940_num_14_1_1118.
Heath, Richard and Heath, Robin. "The Meaning of Le Ménec: A Study of the Moon Using the Circumpolar Stars." Academia.edu. Available at: https://www.academia.edu/5384849/The_Meaning_of_Le_Menec_A_Study_of_the_Moon_using_the_Circumpolar_Stars.
Petrie, W.M. Flinders, 1883, The Pyramids of Gizeh. https://www.ronaldbirdsall.com/gizeh/petrie/index.htm
Plato. Laws, Book VII, in Plato in Twelve Volumes, Vols. 10 & 11, translated by R.G. Bury. Cambridge, MA: Harvard University Press; London: William Heinemann Ltd., 1967 & 1968. Available at: https://www.perseus.tufts.edu/hopper/text?doc=Perseus%3Atext%3A1999.01.0166%3Abook%3D7.
Plato. Timaeus, in Plato in Twelve Volumes, Vol. 9, translated by W.R.M. Lamb. Cambridge, MA: Harvard University Press; London: William Heinemann Ltd., 1925. Plato. Timaeus, 360 B.C.E., 39d, Translated by Benjamin Jowett. Available at: https://classics.mit.edu/Plato/timaeus.html.
Plato, Republic, 546, from Plato in Twelve Volumes, Vols. 5 & 6 translated by Paul Shorey. Cambridge, MA, Harvard University Press; London, William Heinemann Ltd. 1969. https://www.perseus.tufts.edu/hopper/text?doc=Perseus%3Atext%3A1999.01.0168%3Abook%3D8%3Asection%3D546b
The Aryabhatiya of Aryabhata. Translated with notes by Walter Eugene Clark. Chicago: University of Chicago Press, 1929. Available at: https://ia801309.us.archive.org/35/items/The_Aryabhatiya_of_Aryabhata_Clark_1930/The_Aryabhatiya_of_Aryabhata_Clark_1930.pdf.
Notes
Timaeus, Plato, 360 B.C.E, 39d, Translated by Benjamin Jowett, https://classics.mit.edu/Plato/timaeus.html
Ibid.
Ibid
Ibid
Plato, Timaeus, 39, from Plato in Twelve Volumes, Vol. 9 translated by W.R.M. Lamb. Cambridge, MA, Harvard University Press; London, William Heinemann Ltd. 1925. This passage comes with a note from the translator: “the Great World-Year, which is completed when all the planets return simultaneously to their original starting points. Its length was variously computed: Plato seems to have put it at 36,000 years (Cf. Rep. 546 B ff.).”
Plato, Republic, Book 10, 617, from Plato in Twelve Volumes, Vols. 5 & 6 translated by Paul Shorey. Cambridge, MA, Harvard University Press; London, William Heinemann Ltd. 1969. https://www.perseus.tufts.edu/hopper/text?doc=Perseus%3Atext%3A1999.01.0168%3Abook%3D10%3Apage%3D617
Plato, Laws, Book 7, from Plato in Twelve Volumes, Vols. 10 & 11 translated by R.G. Bury. Cambridge, MA, Harvard University Press; London, William Heinemann Ltd. 1967 & 1968. https://www.perseus.tufts.edu/hopper/text?doc=Perseus%3Atext%3A1999.01.0166%3Abook%3D7
Plato, Timaeus, 36, from Plato in Twelve Volumes, Vol. 9 translated by W.R.M. Lamb. Cambridge, MA, Harvard University Press; London, William Heinemann Ltd. 1925.
Cornford, Francis Macdonald, 1937, Platos Cosmology, pp92-93
https://archive.org/details/in.ernet.dli.2015.221748/page/n111/mode/2up
Plato, Republic, book 8, 546, from Plato in Twelve Volumes, Vols. 5 & 6 translated by Paul Shorey. Cambridge, MA, Harvard University Press; London, William Heinemann Ltd. 1969. https://www.perseus.tufts.edu/hopper/text?doc=Perseus%3Atext%3A1999.01.0168%3Abook%3D8%3Asection%3D546b
The Aryabhatiya of Aryabhata, An Ancient Indian Work on Mathematics and Astronomy, translated with notes by Walter Eugene Clark, Professor of Sanskrit in Harvard University, The University of Chicago Press, Illinois, 1929. p.8
Plato, Laws, Book VII, from Plato in Twelve Volumes, Vols. 10 & 11 translated by R.G. Bury. Cambridge, MA, Harvard University Press; London, William Heinemann Ltd. 1967 & 1968.
See for example Heath Richard & Heath Robin, The Meaning of Le Ménec, https://www.academia.edu/5384849/The_Meaning_of_Le_Menec_A_Study_of_the_Moon_using_the_Circumpolar_Stars
Appendix
Some pages from Bailly's Histoire de l'Astronomie Ancienne on the subject of planets.


















Comments