The three biggest pyramids at Giza can be encompassed by a huge rectangle on the ground, from the top, north-east corner of the Great Pyramid, to the bottom, south-west corner of the third pyramid. The width of this rectangle measures 29 227.2 inches, according to Flinders Petrie. The width in inches is very close to ten times the number of days in 8 years.

There is a historically important period of time of 8 years, called the octaeteris. 8 solar years of 365.25 days contain 2922 days, and this period also closely aligns with a cycle of Venus of 8 years, as well as 99 lunations (99 x 29.53059 = 2923.52841 days). The 8 year period of time is therefore important in reconciling the cycles of the sun, of the moon and of Venus. Can we think of the width of the Giza rectangle as expressing a cycle of time in inches? If so, what other cycles of time can we find expressed in the dimensions, or the proportions, at Giza? If we were to find a pattern of time cycles expressed in the proportions, or the dimensions, or both, at Giza, we might be able to put forward the case that:

1. expressing a mathematical and astronomical system in stone was indeed part of the design of the architects, and

2. more generally, dimensions of ancient sites can encode valuable information and should therefore be taken, and read carefully.

Firstly, we'll look at the proportions only, and secondly we'll look at the role certain key units of measure play.

Part 1. Astronomical cycles found in the proportions at Giza

Leaving aside for now the thorny issue of how modern inches might have been used in a place designed several thousand years ago, let's begin with a look at the proportions at Giza.

Let's stay with the rectangle we just looked at (see the diagram above), which encompasses the outer corners of the Great Pyramid and the third pyramid, and give it a name, so we can refer to it later: Great Giza Rectangle, or GGR. What proportions can be said to define this rectangle?

Great Giza Rectangle Proportions

This is one of the most written about parts of the Giza plateau, especially since John Legon published a study on this rectangle (5), in which he defined the length to width ratio as √3 :√2. However, this provides only a loose fit to the site dimensions, which seems out of keeping with the high degree of precision found at this site. This brings up the question: how much leeway do we have in our interpretations of the proportions and dimensions of this ancient site? Sir Flinders Petrie was very clear in his work that the precision which the architects and builders worked with was high. In his study of the site, the error margins he gives are very narrow, and the difference in length between the four sides of a pyramid is within a few inches. While we should allow for some error, especially on a large scale, like the width and length of the site, we should look for the numerical interpretation of the architecture that fits best with, and which is found to be the most consistent with the site with both other sections of it, and with an overarching theme or idea found elsewhere at Giza.

The rectangle dimensions given by Sir Flinders Petrie are 29 227.2 inches for the width and 35 713.2 inches for the length. A quick note on these dimensions: Petrie gives these dimensions to within a tenth of an inch, which is in keeping with the precision found at Giza. He also gives his measure for the length and width of the site in relation to a consideration which is useful in achieving greater precision, and this is the orientation of the pyramids. He writes:

For him, the site is orientated to a point diverging from true north by approximately – 5' 40", and this is the orientation he has used to measure the north-south span.

Why do I use the measures found by Petrie and not those by Glen Dash? Because the figures that Flinders Petrie published are much more precise. We need to take into account certain variations or errors when interpreting the measurements: errors in the building process, errors in the subsequent measurement process, erosion and vandalism, and later when we look at the units involved, tiny variations in the length of the inch over time, and in astronomical cycles, both as they were thousands of years ago, and as they were measured thousands of years ago. But we also need to aim for the highest degree of precision possible in matching our interpretation to the measurements.

Let's see how a √3 :√2 ratio between length and width works:

Petrie Width giving the calculated length

29 227.2 x  √3 / √2 = 35 795.8633

Petrie Length giving the calculated width

35 713.2 x √2 / √3 = 29 159.7057

Differences between the Petrie and calculated measures:

29 227.2 - 29 159.7057 = 67.4943

35 795.8633 - 35 713.2 = 82.6633

67.4943 inches = 5.624525 feet = 1.7144 metres

82.6633 inches = 6.8886 feet = 2.0996 metres

The difference between the width multiplied by √3 / √2 and the actual length as measured by Flinders Petrie is 82.6633 inches, or 2.0996 metres. The difference between the length multiplied by √2 /√3 and the actual width measured by Flinders Petrie is 67.4943 inches or 1.7144 metres. Just for comparison, Glen Dash gives 741.3 +-1.2 metres for the width and 908.2 +- 1.2 metres for the length.

John Legon's theory has the merit of introducing the square roots of two and three into the interpretation of the design. However, the rectangle dimensions don't fit well with a √3 :√2 ratio. Because there is a difference of 5 or 6 feet or so off a true √3 :√2 ratio, the theory is undermined, as a lack or precision, even across so great an area, seems out of character with the rest of the Giza site, where a high degree of accuracy can be found, for example in the relative lengths of the sides of the pyramid bases.

A brief word on the subject of irrational numbers in relation to ancient Egypt, as they are the subject of some controversy in this context. The ancient Egyptians, who designed and built the pyramids of Giza, structures which are beyond our capabilities today, and which are still standing after thousands of years, are not widely supposed to have gained knowledge of irrational numbers. An analysis of the Giza dimensions and proportions suggests otherwise, as well as an analysis other ancient sites in Egypt and of cubit rod markings. Who are we to say what the ancient Egyptians were and weren't capable of? It is true that there is no clear explanation of irrational numbers in the texts that have survived from ancient Egypt, but how can we possibly take those texts as complete expressions of everything they knew? We certainly can't prove that the ancient Egyptians didn't know about irrationals, anymore than we can prove that they did. As it happens, a close examination of the site, at Giza, suggests not only that pi and other irrationals were used, but also that approximations close to our own today, as they appear on a calculator, work best when trying to interpret the design.

The best known use of an irrational at Giza is the ratio between the height and side of the Great Pyramid, as the height multiplied by pi / 2 gives the side. If we compare how well the value given by a modern calculator works , and an often suggested value, 22 / 7, then calculator pi is a much closer fit. The height is given by Petrie as 5776.0 ± 7.0 inches, and the side as 9068.8±0 .5  inches. Moreover, if we take the value of the height as 10 000 / √3 inches, and then multiply by pi / 2 (calculator values), then the correspondence to the Petrie values is very good. A single ratio doesn't prove anything, especially when we take into account the error margin given by Petrie, but there is certainly room for debate about whether the Giza architects used a value of pi close to our modern approximation. Another thing to bear in mind is that the ancient Greek philosophers, some of whom spent time in Egypt, though of course much later than the pyramid building, knew about irrational numbers. The Greeks accorded a special status to irrational numbers, and guarded knowledge of them as an important secret, as we know from the story of the murder of Hippasus, who was punished for revealing it. According to John Neal:

What other ways can we find to try to understand the proportions of this GGR rectangle?

A 9:11 ratio is already a much better fit. The difference between the width and length being multiplied by this ratio and the actual measured width and length is only in the region of a few inches, 7.309 and 1.06667 respectively. And because it is a better fit, it is better to try to interpret this 9:11 ratio as a possible intention of the architects, rather than the previous one, put forward by John Legon. I didn't come up with this ratio myself, but I don't know who was the first to do so.

Width giving the length

29 227.2 x 11 / 9 = 35 722.1333

Length giving the width

35 713.2 x 9 / 11 = 29 219.89

Difference between the Petrie values and the results

29227.2 - 29 219.89 = 7.309

35 723.2 - 35 722.1333 = 1.06667

The significance of 9 and 11 can be interpreted as being connected to the approximately 99 lunations in an 8 year period (9 x 11 = 99). We saw that an 8 year period can be linked to the length in inches of the width of the Great Giza Rectangle.

99 x 29.53059 = 2 923.52841

8 x 365.25 = 2 922

However, a grid drawn over the Giza site of 9 x 11 (in blue below) or 4 x 5 (in pink and green below) doesn't seem to offer any insight as to the layout of the pyramids.

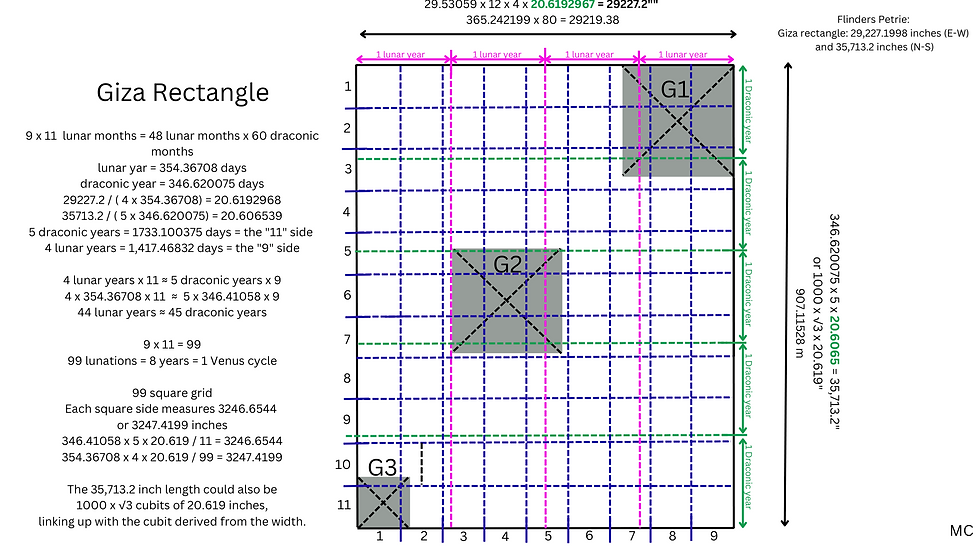
Do we find this 9:11 ratio elsewhere at Giza? Possibly, there may be a connection with the second pyramid, in terms of the length of its side in inches and the Metonic period of 19 years, as the number of days in this period, if taken to be a whole number of days, as Meton of Athens did in the 5th century B.C., is 6,940, which, multiplied by 11 / 9 is 8482.222, about 7 inches more than the side of the second pyramid. Maybe a 7 inch difference is too great to take this seriously as a good fit.

But staying with proportions for now, and with this large rectangle surrounding the pyramids, what other possible ratios might we consider? I've come up with a few options, listed below.

Of these options, the two ratios that match the width and length of the Great Giza Rectangle most closely are:  length x 354.36708 x 4 /(1000 x √3), and length x 365 / (2 x 223). However, all the ratios listed are quite close matches, and they can perhaps all be considered possibilities. The square root of three has made a return here, and we have a few new players: the lunar year, of 354.36708 days, the lunation of 29.53059 days, the draconic year of 346.6201 days, the Saros cycle of 223 lunations, and also 254 sidereal months. The difference between the result and the width as measured by Flinders Petrie is also noted below.

35 713.2 x 354.36708 x 2 x √2  x 12⁴ / (254 x 10⁵) = 29 222.5693, difference of 4.6307 inches (result is 99.98416 % of Petrie's measure).

35 713.2 x 354.36708 x 9 / 10 000 x √2 x π / √3 = 29 216.5471, difference of 10.6529 inches (result is 99.9636 % of Petrie's measure).

35 713.2 x 354.36708 x 4 / (346.6201 x 5) = 29 209.1137, difference of 18.0863 inches (result is 99.9381 % of Petrie's measure).

35 713.2 x 29.53059 x 48 / (1000 √3)  = 29 226.8156, difference of 0.3844 inches (result is 99.9987 % of Petrie's measure).

35 713.2 x 365 / (2 x 223) = 29 227.1704, difference of 0.0296 inches (result is 99.9999 % of Petrie's measure).

35 713.2 x 360 / (√3 x 254) = 29 223.8156, difference of 3.3844 inches (result is 99.9884 % of Petrie's measure).

35 713.2 x 9 / 11 = 29 219.8909, difference of 7.3091 inches (result is 99.97499 % of Petrie's measure).

   The results are quite close to Petrie's value. The ratio between width and length which is the closest to Petrie's measures is not necessarily the best way of describing the proportions of this rectangle, there may be several valid ways. Moreover, we have to take into account errors in the measuring process, errors in the building process, erosion, etc, as well as remember that we cannot know the intentions of the architects, only try to come up with a model that fits well overall and has meaning.

Cycles of Time

  In terms of astronomy, trying to get back to what would have been known by the architects of the pyramids of Giza is tricky. It helps to look to what the Greeks knew, since they have left us many texts. But we can't use the limitations of Ancient Greek or 18th century European astronomical knowledge as proof of the limitations of ancient Egyptian knowledge, which goes back much further in time. If this sounds contradictory, because we are often told that the Greeks were the first to achieve various things in maths and astronomy, we can go back to the History of Ancient Astronomy written by Jean-Sylvain Bailly in the 18th century and see there proof that it would have been impossible for anyone in 3000 BC to come up with some of the things they knew in terms of astronomy in such a short time as a few hundred years. Furthermore, Bailly shows that the many connections we see in astronomy around the world are pieces, or as Bailly calls them, debris, of a former common and very ancient system.

If we are going to recognise astronomical cycles in the dimensions of Giza, we need to know what the cycles are. These are some of the main cycles harmonising the motion of the sun and the moon and the stars as seen from earth:

We can also add to this the various types of lunar month (synodic, sidereal, draconic), or year (sothic, tropical, sidereal), as well as other moon cycles such as the lunar nodal period of 18.6 solar years, and the motion of planets such as Venus and Mars.

The Saros cycle is a period of approximately 18 years, 11 days, and 8 hour, or 223 synodic months, almost exactly equivalent to 254 sidereal months, after which the Sun, Earth, and Moon return to approximately the same relative geometry. This results in a similar alignment of the three bodies and a potential for eclipses to recur in a series. The Saros cycle was important in ancient astronomy for predicting eclipses. The Metonic cycle relates to the recurrence of the positions of the Sun and Moon in relation to the Earth, and lasts approximately 19 years, which is almost exactly 235 lunations, after which the phases of the Moon and the days of the year align nearly perfectly. It arises from the relationship between the synodic periods of the Sun and Moon. Like the Saros cycle, the Metonic cycle is used to synchronise lunar and solar calendars and predict the occurrence of specific celestial events, such as full moons or equinoxes.  Any system of astronomy capable of knowing these cycles is incredibly sophisticated, and already many centuries old.

The way astronomy is practised now is totally different to the way it would have been thought of a few centuries ago, or in antiquity. To try to learn as much as possible about this other kind of astronomy, I have learned a lot from 17th and 18th century books, as well as from ancient Greek and Indian texts. In particular, the work of Jean-Sylvain Bailly has been invaluable.

Jean-Sylvain Bailly (1736 – 1793) was not only an astronomer, but also a mathematician, historian, and a member of both the prestigious Académie des Sciences, and the Académie Francaise.

Bailly published A History of Ancient Astronomy in 1775. In it he systematically reviews all the evidence at hand from ancient sources, as well as accounts from travellers in his own time, and as an astronomer, is able to piece together a narrative about the history of this science. In 1787 Bailly also published a treatise on Indian and Eastern astronomy.

In the 18th century, people were much better versed in the Latin and Greek writers than today, so I have found his help invaluable from that perspective too. Bailly is a fascinating character, and an original thinker, as we can see when we read his History of Astronomy. His correspondence with Voltaire is also well worth reading.

Bailly was also a politician who became mayor of Paris, in dangerous times. After regrettably giving orders to shoot into a crowd to disperse a protest, he was guillotined.

Also of huge interest is the story of Guillaume Le Gentil, or rather, with his full name, Guillaume Joseph Hyacinthe Jean-Baptiste Le Gentil de la Galaisière (1725 – 1792). He was an astronomer, and also a member of the Paris Royal Academy of Sciences. He was one of many astronomers taking part in an international effort to observe the 1761 transit of Venus from many points of the earth, which would help to determine the earth's distance from the sun. Over a hundred observers were sent out into the world, and Le Gentil was sent to Pondicherry in India. Only, when he arrived, he was told the city, which had been in French hands until recently, had since been taken by the British, and that they could not stay. When the day of the transit came, the ship was still at sea, and Le Gentil was unable to take measurements. So, having travelled so far, he decided to stay on till the next transit of Venus, just a short eight year wait away. In the meantime he studied Indian astronomy. On his return to Paris, where he learned to his dismay that he'd been presumed dead, and that his property had been passed on to relatives, he published an account of his adventures and studies.

The three main pyramids

Let's have a look at some other proportions at Giza. How does this Great Giza Rectangle (GGR) relate to the sides of the main three pyramids? In the diagram below, the width of the rectangle provides the starting point. I have used one of the possible ratios seen above to link it to the length of the rectangle, but any of the others in the diagram above would work too. The length of the rectangle is then linked to the distance between the centres of the Great Pyramid and the third pyramid by the fraction: (254 x 4 / 1 000 )², and to the perimeter of the Great Pyramid by the fraction: 254 x 4 / 1 000. There is a remarkable similarity between these two fractions, which suggests a pattern, and an intention. The length of the GGR multiplied by 254 x 4 / 1 000 gives the perimeter of the Great Pyramid, which itself multiplied by 254 x 4 / 1 000 gives the distance between the centres of the Great and third pyramid. We'll return to the question of the significance of 254 when we look at the units used. For now, it's enough to say that it's the number of sidereal months in a 19 year Metonic cycle.

The diagram below shows how the side of the third second pyramid is linked to the length of the Great Giza Rectangle (GGR) by a combination of astronomical numbers derived from the Saros and Metonic cycles: 223 and 235. And the GGR length is linked to the side of the Great Pyramid and to the side of the third pyramid through the number 254, a number derived from the Saros cycle, and 29.53059, the number of days in a lunation, in the case of the third pyramid.

We've seen that the length of the GGR multiplied by 254 /1000 gives the side of the Great Pyramid. The length of the GGR multiplied by 223 / 235 x 1/4 gives the side of the second pyramid. The length of the GGR multiplied by 29.53059 / 254 gives the side of the third pyramid. Below are the figures with the dimensions.

The mean base side of the Great Pyramid is given by Petrie as 9068.8 inches and the length of the GGR is 35 713.2 inches.

35 713.2 x 254 / 1000 = 9071.1528, a difference of 2.3528 inches (result is 100.0259% of Petrie's measure)

The mean base side of the second pyramid is given by Petrie as 8 474.9 inches and of the third pyramid as 4 153.6 inches.

35 713.2 x 223 / 235 x 1 / 4 = 8472.3868, difference of 2.513 inches (result is 99.9703% of Petrie's measure)

35 713.2 x 29.53059 / 254 = 4 152.09396, difference of 1.506 inches (result is 99.9637 % of Petrie's measure.

Interestingly, 223 / 235 x 29.53059 = 28.0226, very close to 28. (And as we shall see later to √3 x 3000 / (29.53059 x 2π) = 28.00463)

So we can link the sides of the pyramids to each other very simply in this way: Great Pyramid side x 223 / 235 x 29.53059 / 30 gives the second pyramid side, albeit a little smaller than Petrie's measure, by 3.8 inches, and Great Pyramid side x 29.53059 x 1000 / 254² gives the third pyramid side, also a little smaller than Petrie's measure, by about 2.6 inches. And so, second pyramid side x 30 000 / 254² x 235 / 223 gives the third pyramid side.

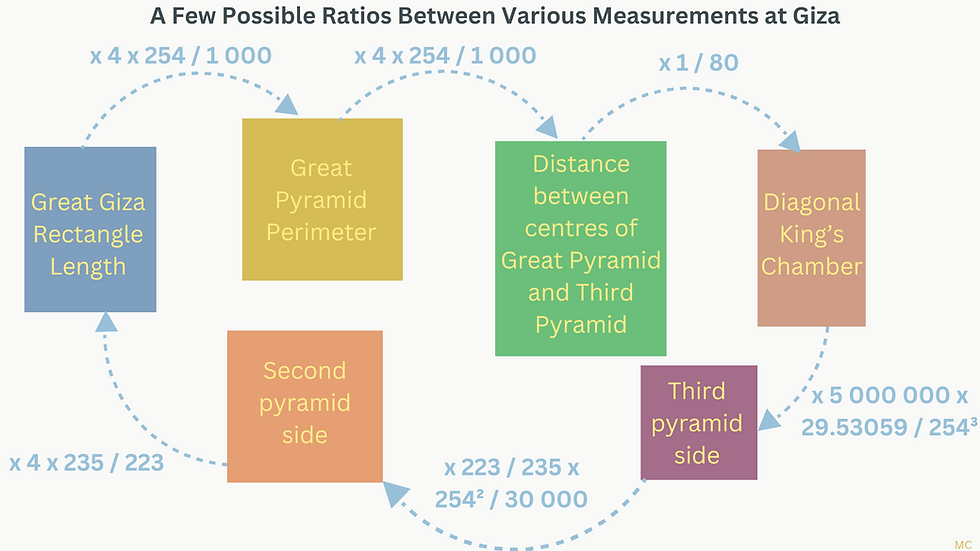
What is the meaning of the x 4 x 254 coefficient? A lunar year is on average 354.36708 days, and this number, combined with 360, the number of degrees in a circle, links the perimeter of the Great Pyramid to the length of the GGR, and top the distance between the centres of the Great Pyramid and the third pyramid, as the image below shows.

The combination of the numbers 354.36708 and 360 may indeed harp back to this notion, explained by Bailly, that there was a practice of dividing up the lunar year into 360 parts, for calculation purposes. The following fractions have very close values and can be replaced one by the other without changing the results much:

360 / 354.36708 = 1.01589572

30 / 29.53059 = 1.01589572

254 x 4 / 1000 = 1.016

So if the GGR length is multiplied by 254 x 4 / 1 000 to give the Great Pyramid perimeter (35713.2 x 254 x 4 / 1 000 = 36 284.6112, which divided by 4 is 9071.1528, compared to Petrie's 9068.8 inches), this could also be a reference to multiplying by 360 / 354.36708, or by 30 / 29.53059. (35 713.2 x 360 / 354.36708 = 36 280.8870, which divided by 4 is 9070.2218 inches).

What does it mean to convert from inches to metres? One inch is 2.54 cm, so to convert from inches to centimetres we can multiply by 254 / 10 000, or, approximately also by 360 / (354.36708 x 40), or more simply by 9 / 354.36708.

The number 360 is 6 x 60, and associated with the sexagesimal number system of the Babylonians. In The Meaning of Le Ménec (12) Richard and Robin Heath observe that three lunar orbits are roughly 82 days and that after this period the moon returns to the same constellation. Richard and Robin Heath show that the number 82 was crucial to the design of le Ménec, and they show"the evidence for megalithic lunar simulators, using metrological geometrical constructions involving 82 elements, based upon stones discovered set within a partial ring", adding that "This control of time counting within geometrical structures reveals that almost all of Le Menec’s western cromlech and alignments express a necessary form, so as to represent a megalithic study of (a) circumpolar time as having 365 time units, (b) the moon’s orbit as having 82 times 122 of those units and (c) the variations of successive moonrises over most of a lunar nodal period of 18.6 solar years."

Indeed, it may well be that the megalithic yard, as a number of feet, is so close to a tenth of a draconic month in days, as 3 draconic months of 27.2122 days make 10 megalithic inches, and 40 such megalithic inches make a yard of 32.65464 inches or 2.72122 feet. For this reason, we should not be surprised to find megalithic yards at Giza if indeed, transferring time counts to spatial counts was practised there.

There is a curious numerical correspondence which brings together the number 82, the number of days in a lunation (29.53059), the number of days in a draconic month (27.2122), the number of times the circumpolar stars will have rotated in 365 days(366), 360, 108, and pi and the square root of three.

82 x 366 x π / (2 000 x √3) = 27.21787

(27.2122 x 3 +1) x 2π x 366 x 29.53059 / (9 000 x √3) = 359.999901 ≈ 360

(27.2122 x 3 +1) x 2π x 366 x 29.53059 / (3 000 x √3) = 107.999970 ≈ 108

The 2π x 29.53059 / (3 x √3) element of these equations will come back again later.

To go back to the ratios between the GGR length and the sides of the three main pyramids, one of the ratios used there is GGR length x 223 / 235 to give the perimeter of the second pyramid. Why are 223, the number of months in a Saros period, and 235, the number of months in a Metonic period, being used together in this way? Bailly tells us that the period of 223 lunar months (lunations), i.e. the Saros period as Halley termed it, was very useful for predicting eclipse patterns but:

(History of Astronomy, p 65, see below)

The way these two periods would have worked together adds significance to the ratio between the GGR length and the second pyramid perimeter. The ratio 235 / 223 comes back frequently at Giza.

A Metonic rectangle

Could the large rectangle that links the centres of the Great Pyramid and third pyramid be Metonic in its proportions? Sir Flinders Petrie gives the length of the distance between the centres of the Great Pyramid and the third pyramid as 36 857.7 inches, which is 936.18558 m. If this line is taken as the diagonal of a rectangle, the length of this rectangle, north-south, is 29102 inches, or 739.1908 m, and the width, west-east, is 22 616 inches, or 574.4464 m.

   If we take the width and multiply it by 29.53059, the number of days in a lunation, and 38, we get the length. And conversely, if we multiply the length by 365.242199, the number of days in a year, and divide by 470, we get the width again.

   22616 x 38 / 29.53059 = 29102

  29102 x 365.242 / 470 = 22616

   A lunation of 29.53059 days, multiplied by 47 x 10, and divided by 19 and 2, gives the solar year, approximately, as 365.24677. This means the number of lunar months on average per year can be simplified as 470/38, which is 12.36842 and 365.242199 / 29.53059 = 12.368266. This ratio echoes a discovery made by Jim Wakefield at the Rollright Stones in England initially, and subsequently also at other stone circles such as Stonehenge. At the Rollrights, the radius of a circle was 47 Saxon feet (of 13.2 inches), and the circumference was approximately 10 lunar months in days expressed as Saxon feet also. The other important lunar aspect of a circle with a radius of 47 units, which Jim Wakefield found, was that the area of such a circle will have almost exactly the same number of square units as there are days in a Metonic cycle.

• Metonic cycle: 29.53059 x 235 = 6939.68865 days

• 19 solar years are almost exactly 235 synodic months or lunations, as well as almost exactly 254 sidereal months.

• The circumference of a circle with a radius of 47 units is 295.30971

• The area of circle with radius of 47 units: 47² x π = 6939.77817 units squared

• A Metonic period in days is also approximately equal to π x 235² / 5²
  

    At Giza we have a rectangle instead of a circle, and the ratio between the width and length is 38 / 29.53059, to within about half an inch: which is roughly equal to 365.242199 / 470. The width of 22 616 x 38 / 29.53059 = 29 102.29697. The length as given by Flinders Petrie is 29102. This 29102 multiplied by by 365.242199 / 470 = 22 615.47379, which is 0.5262 inches less than Flinders Petrie's figure. The Metonic rectangle does not rely on any unit of measure, but only on proportion. It shows the possible use of geometry to express the Metonic cycle not just with a circle, as at the Rollrights, but also with a rectangle.  It is ironic that the geometric shapes at Giza are primarily the rectangle, the square and the triangle, the last two of which make up a squared based pyramid. Yet the repeated presence of pi suggests the circle is also present, if only in spirit.

The importance of irrational numbers at Giza and how they interact with astronomical numbers, in the proportions of the pyramids and of the site plan

Another instance of the geometry of the circle being expressed indirectly through another shape is in the Great Pyramid itself. The Great Pyramid's base and height are linked by pi (π), and the height and the slope are linked by Phi (1.61803). The height multiplied by π/2 gives the side, and the height multiplied by π/4 x 1.61803 gives the slope. We saw that the proportions of the GGR can be understood as one of several possibilities which combine irrational and astronomical numbers, such as length x 29.53059 x 48 / 1000 √3 = width, or length x 365 / (2 x 223) = width. The same is true of the ratios between side and height in the three main pyramids, either an irrational number on its own, or combined with a lunar number. See the three diagrams below.

The second and third pyramids also embody irrational numbers in their proportions. The second pyramid side multiplied by 2π² / 29.53059 gives the height, as the diagram below shows.

And the side of the third pyramid divided by Phi gives the height.

There are also possible ratios between the pyramids.

These ratios can be interpreted as pleasing ways to model the pyramids. But perhaps there is a deeper meaning to these numbers. For a start, irrational numbers have a special status, because they are impossible to define exactly. There is no known number, which squared, produces the number 2, or 3, or 5. (8) Of course, we can use excellent approximations, but there is always something elusive about irrational numbers. This could point to a connection to another world, beyond the scope of human understanding, beyond finitude, in the eyes of a culture which is profoundly interested in questions of the divine. When an irrational number is combined with a number derived from astronomy, such as the number of days in a lunation, it be a reflection on the divine nature of the universe, within which these key cycles of time operate, and seems to be the creation of a supernatural mind.

Curiously, when irrational numbers are used together with astronomical numbers, they blend into each other. That is, the astronomical time cycles can be approximated quite well by equations featuring irrational numbers, and irrational numbers can be approximated quite well by equations which feature astronomical numbers. Below is a list of some time cycles which can be approximately expressed with the use of irrational numbers combined with other time cycle numbers, as well as 2, 3, 44, or multiples of 10.

And below is a list of irrational numbers which can be approximated by astronomical numbers, as well as 2, 3, 44 and multiples of 10.

The diagram below shows that a lunar year in inches can be drawn out as a line, as the outcome of a process which begins with a square with sides of 20 metres. The diagonal of the square is turned into the circumference of a circle, and the diameter of this resulting circle will give the lunar year in days with a day represented by an inch, with an error of 0.08812 inches, or 2.238 mm. To obtain an exact correspondence, within the limits of applied geometry, we would need a metre of 39.360286 inches.

Geometry and astronomy are also linked for example with the megalithic yard and the draconic year. A square with sides of 8,000 inches will have a diagonal of 346.6201 megalithic yards of 32.64 inches, or 0.829058 m.

A circle with a circumference of 235 units will have a diameter of 19 x 254 units. Or we can also say, a circle with a circumference of 235 inches will have a diameter of 1.9 meters (or 190 cm).

Another example of linked geometry and astronomy is with the circle:

The number of lunations in a year, on average, can be approximated by this fraction: π x √3 x 100 / 44 = 12.36681. So that even pi and the square root of three can be considered lunar. The 440 cubits present in the mean base side of the Great Pyramid can also be related to this equation. And the mean base side of the Great Pyramid can be understood as π x √3 x 10 000 / 6 = 9068.9968 inches.  If the base side is π x √3 x 10 000 / 6 inches, the average number of lunar months per year can be understood as base side in inches x 6 / 4400 = 12.36681. In this way 440 can be understood as a lunar number, and also 44 lunar years are approximately 45 draconic years. The base is also 365.242199 / 29.53059 x 10/6 x 440 = 9070.0619 inches.

  A synodic lunar month (lunation) can be approximated by 10 000  π x √2 / (47 x 32) = 29.5404451.

The mean base side of the Great Pyramid, as 5000 π / √3, can be multiplied by √2 to obtain the diagonal of the base square, multiplied by 3 / (47 x √3 x 16) = 29.5404451, or more simply (5/4)² x 1 000 π x √2 / 235, the 235 being the number of lunations in a Metonic cycle, and (5/4)² x 1 000 / 235 being approximately equal to 47 x 10 x √2 .

A lunar year, of 354.36708 days, can be approximated by 100 x √12 = 200 x √3 = 346.4102

The heights of the Great and second pyramids can be approximately linked by a coefficient of 29.53059 x 223 x π² / (30 x 47²). The Saros cycle is 223 lunations of 29.53059 days. The height of the Great Pyramid is given as 5 776.0 ± 7.0 and of the second pyramid as 5 664 ± 13, by Petrie.

5 776 x 29.53059 x 223 x π² / (30 x 47²) = 5 664.8308

The difference between this result and Petrie's measure is 0.8308 inches.

The height of the Great Pyramid and the side of the second pyramid can be linked by a coefficient of 29.53059 x 223 x π² / (30 x 47²), the same as above except that pi and 47 are not squared, and there is a multiplication by 10. The mean side of the second pyramid is 8 474.9 inches.

5 776 x 29.53059 x 223 x π / (300 x 47) = 8 474.9068

The difference between this result and Petrie's measure is 0.006814 inches.

This tells us that the ratio between height and base side of the second pyramid can be understood as 10 π / 47. The base side is 8474.9 inches, the height is 5664 inches.

8474.9 x 10 π / 47 = 5664.8263

As mentioned earlier, another good for the ratio between side and height in the second pyramid is side multiplied by 2π² / 29.53059 to give the height, as 2π² / 29.53059 is very close to 10 π / 47 (equal to 0.6684325915 and 0.6684239688 respectively), so can be used interchangeably in this context.

By comparison, a 3:4:5 model for the second pyramid is not quite as good a fit. The idea is that the half base is the "3" side of a 3:4:5 triangle, and the height the "4" side. 8474.9 / 2 gives the half base, and then divided by 3 and multiplied by 4 should give the height, but this figure is 8474.9 / 6 x 4 = 5649.9333, a difference of 14 inches from Petrie's figure, who gives a margin of error of 13 inches. Still, it is a strong possibility.

The 3:4:5 triangle is very important in megalithic structures, as are other Pythagorean triangles. It is interesting to note that: (223/ 235)² x 100 / 54 ≈ 5 / 3

and (360 x 235 x 19)² / 10¹¹ x 2 / π³ ≈ 5 / 3, with 223 being the number of lunations in a Saros cycle, 235 the number of lunations in a Metonic cycle, and 19 the number of years in a Metonic cycle. The number 54 is the number of digits in a metre, and twice 27.

We can see some of these astronomical numbers at work in the ratio between the heights of the Great and second pyramids. 47 is 235 x 2 / 10.

Also in the ratio between the height of the Great Pyramid and the side of the second.

The diagonal of the GGR multiplied by 9 / 100 gives the side of the third rectangle. This shows that while it is difficult to find nice integer numbers of a particular unit around Giza, there are definite correspondences between elements of the site.

    Sometimes these connections are not straightforward and imply the intermediary of a circle, as the diagram below shows.

A mathematical astronomical system

According to this model, there seems to be a pattern of astronomical cycles being expressed in the proportions, both within a structure, such as a rectangle on the ground, or a pyramid, and also between structures. The ratios mix astronomical numbers with irrational numbers. 223 / 235​ and 29.53059 / 30, could be significant in the context of ancient astronomy or time-keeping systems.

It is well known that ancient sites were often connected to astronomy, all around the world, from Stonehenge to Chichen Itza, from Machu Picchu to Karnak and Carnac. While it's perhaps not that surprising to find a connection to astronomy at Giza, what is surprising is that the proportions of the structures, and of the site itself, may depend on an pre-existing body of astronomical knowledge, which could have been used to give shape and form to these numbers. It is a mathematical system which connects the various parts of the site in terms of astronomical cycles, which uses not only key astronomical numbers, but also irrational numbers, neither of which is widely accepted to have been used by the ancient Egyptians.

Part 2. Astronomical systems found in the units of measure at Giza

We've seen that some of the proportions at Giza seem to match certain astronomical numbers. Let's look at how the dimensions expressed in various units might also reflect these astronomical numbers.

Did the builders of the pyramids of Giza encode astronomical and calendrical information into the layout and dimensions of these structures using systems of measurement? One initial problem is the lack of concrete evidence supporting the existence of the units of spatial measure designed to convey units of temporal measure, in the historical archives. Yet, analysing ancient sites from the megalithic world, and other pyramids does produce similar theoretical outcomes, which we can see in the work of researchers such as the Heath brothers, and Howard Crowhurst, for example. In particular, their work has produced the concept of the "day-inch" as a historical unit of measurement, used by ancient peoples, to encode cycles of time in spatial designs. Jim Wakefield has shown that the Saxon foot was used to encode lunar cycles at the Rollright Stones, at Stonehenge and at Stanton Drew, as well as at Giza (including Mars cycles).

While the concept of encoding time cycles into linear measures remains controversial, it's an attempt to understand the design of these impressive monuments, and their relationships with astronomy and timekeeping.

There is additional controversy in the types of units we find associated with time cycles in the dimensions of ancient sites. While there is no historical evidence that the pyramids of Giza were designed with measures presented in Egyptian cubits, the cubit remains at the forefront of most researchers' minds when analysing the dimensions of the pyramids. While there is no evidence that the designers of the pyramids did not use inches, megalithic yards, Saxon feet, metres or inches, many researchers prefer the idea of cubits in association with the architects of Giza, and go as far as to state that these other units just mentioned, inches, feet, megalithic yards, inches, were not used in the design. Either way, there is no written evidence to base such statements on, so we should analyse the dimensions, without prejudice, and evaluate the results as best we can, in good faith, to come up with models that fit the site.

The number 1 (or 10 000, or 1 000 000) as a starting point

   The Pythagoreans believed that all is number, or ἐν τῷ ἀριθμῷ δέ τε τὰ παντ' ἐπέοικε,

meaning everything fits in the number. (7) But what is all, or everything - the container or the contained? The number 1 is a good place to start thinking about the universe, in the old sense of the word, that thing within which everything is. And 1 is also the primary building block of the universe, the beginning of division, multiplication, of things.

Plato, in Phaedrus, says the journey of a soul is 10 000 years.

The number 10 000 can be considered a starting point of the Great Pyramid and Great Giza Rectangle (GGR) dimensions, even though there is no clearly marked out linear length of 10 000 inches, or metres, or cubits. The height of the Great Pyramid can be interpreted as 10 000 / √3 inches, and the length of the GGR can be interpreted as 1 000 000 / 28 inches. We will return to the numbers 28 and  √3, but for now, what is the significance of 10 000 and 1 000 000?

   Firstly, these large numbers could simply be references to the number 1, with all the symbolism that it offers, and 10 000 or 1 000 000 might be said to embody a similar role, albeit in different orders of magnitude.

   Secondly, this could be a reference to an actual period of time, a cycle of 10 000 years, about which very little is known. Jean-Sylvain Bailly writes:

   The 60 and 180 year cycles clearly point to reconciling the movement of the sun and the moon as seen from earth: the 60 year cycle is well known, and 3 such cycles make up 180 years. But there is no other reference in the history of astronomy to a 10 000 year cycle, nor does it seem to be very useful.

   Thirdly, as Howard Crowhurst points out (15), the number of hours in a lunation multiplied by 127 and divided by 9 is very close to 10 000. The number 127 multiplied by 2 is 254, which we have seen already at Giza.

29.53059 x 24 x 127 / 9 = 10 001.02648.

   This means the height of the Great Pyramid in inches could be interpreted as 29.53059 x 24 x 127 / (9 x √3) = 5774.0953, which could be simplified to 29.53059 x 4 x 254² / (√3 x 30 000). Since 29.53059 x 4/3 is close to 10 000 / 254, we can then also think of the height in metres simply as 254 / √3 = 146.64697.

   The Heath brothers have made a connection between 366 sidereal months and the number 10 000. In The Meaning of Le Ménec, they write:

The diagram below shows how some key elements of the Giza site can be linked to 1 000 000.

Dividing by √3: the Great Pyramid Height

The diagram below gives various possible interpretations of the height of the Great Pyramid in inches and metres, which can be multiplied by √3 to obtain approximately 10 000.

  There is a connection between these two numbers, in that the Great Pyramid height can be approximated both by 10 000 / √3 inches, and by 1 000 / 28 x 254 x 2 / π inches, which means that 280 x π / (254 x 2) approximates √3, as 1.731587. And the lunar year in days is close to 28 000 x π / 254. A similar logic applies to the length of the GGR, as it can be described as the side of the Great Pyramid side x 1 000 / 254. Because of these correspondences, even though they are not exact, it may be that these numbers are extra significant.

The height of the Great Pyramid can be connected to the draconic year. Dennis Payne has made the connection between the draconic year and the height of the Great Pyramid via the number 100 / 6. This may provide a compelling reason to explain the connection to the square root of three here, as it may be a way of interpreting geometrically the draconic year. The space diagonal of a cube (the line connecting two opposite corners of a cube), and the height of an equilateral triangle are both √3 units, when the sides are 1 unit. There are 346.6201 days in a draconic year, and 346.6201 x 100 / 6 = 5777.001667. This is very close to 10 000 / √3, and indeed 5777.001667 x √3 is 10 006.0604. As a measure in inches, 5777 is within Flinders Petrie's error margin for the height of the Great Pyramid. Also, 5 draconic years in days are 1 733.1005, which, divided by 1000, is close to the first few digits of √3, which are 1.73205.

The side of the Great Pyramid, if taken as 9072 inches, divided by the distance between the centres of the Great and third pyramid, as 36 865 inches, is approximately 120 x 29.53059, that is 10 lunar years. (Found by Dennis Payne)

There is another possibility for the height of the Great Pyramid, expressed in inches. The Àryabhatiya of Àryabhata states:

The height, as 5776 inches, is compatible with a thousandth part of the number of revolutions of the moon in a yuga: 57 753 336. The Àryabhatiya of Àryabhata (c. 499) says that a solar year is a year of men.

Therefore a year of the Fathers is 360 years, a number that seems to occur at Giza, and a yuga is 30 x 12 x 12 000 = 4 320 000 years. Time is mostly counted in terms of the stars in the Àryabhatiya, so we have to take the sidereal year and month. Using today's estimates:

4 320 000 x 365.25636 / 27.321661 = 57 752 984.9009.

Using the estimate for the sidereal year from the Àryabhatiya, 365.25868 days, we get an estimate for the sidereal month of:

4 320 000 x 365.25868 / 57 753 336 = 27.321668.

(The Jupiter figure given in the Àryabhatiya, is also quite close to the value in metres for the height of the Great Pyramid: 146 564 compared to 146.7104 m.)

It just so happens that the number of revolutions of the moon in a yuga (57 753 336 according to Àryabhata) is very close to 10⁸ /√3. So it would seem natural to illustrate this relationship between the moon a yuga of 4 320 000 sidereal solar years with an equilateral triangle, which has a height to side ratio of √3 / 2, or with a pyramid with a height of 10 000 / √3 inches.

The square root of three is important at Giza, and we see it in other ratios. Also, the number 440, which is the number of royal cubits in the side of the Great Pyramid, and the number 254, which links various parts of Giza, can combine to create an approximation of √3 in this way: 440 / 254 = 1.732283. A remarkable instance of √3 was found by Quentin Leplat, by lining up one side of each of the main three main pyramids in metres, to create a diameter of a circle, the circumference of which is close to 1 000 x √3 metres. (16)

In any case, we should consider the possibility that Àryabhata, even though he lived hundreds of years after the pyramids were built, was working in a tradition of astronomy and mathematics that held something in common with the tradition the pyramid builders worked in. Therefore the height of the Great Pyramid could be expressing in inches the number of sidereal months in a yuga, divided by 1 000.

The number 4320 is important as it links the Great Pyramid dimensions to the size of the earth, which itself is linked to the length of a day through the number 4320.

The equatorial circumference is estimated today as 24 901.461 miles, converted to inches, 1 577 756568.96 inches. Divided by 4 320 000, this gives 365.221428, which is very close to the year of 365.242199 days. While the mean side of "of the original base of the Great Pyramid casing on the platform" was estimated by Petrie to be 9068.8 inches, the "mean socket sides" were estimated as 9125.9 inches. This mean side of 9125.9 inches corresponds to the number of days in a 25 year cycle, with 365 days per year, approximately equivalent to 309 lunations. 365 x 25 is 9125. Four times this 25 year cycle is 36 500 days long, which is almost exactly 1236 lunations long also. This is 2 x 618, which offers a connection to phi, as Gulya Priskin has observed. Therefore the base perimeter is equivalent to 100 years of 365 days, which is approximately 2 000 x phi lunations. And it is this socket perimeter which relates to the earth's size, through 43 200.

9 125.9 x 4 x 43 200 = 1 576 955 520. This translates into miles as 24 888.81818.

100 x 365 x 43 200 = 1 576 800 000. This translates into miles as 24 886.3636

If the polar circumference is 24 859.73 miles and the equatorial 24 901.461 miles, (Wikipedia) the mean, converted to inches, is 1 576 434 530.88, which are 24 880.5955 miles. If this mean circumference is divided by 43 200 and 4, the result is 9 122.885 inches, which is only 3 inches or so from Petrie's estimate for the mean socket sides.

• earth's equatorial circumference in inches / 4 320 000 gives sidereal solar year in days.

• mean earth circumference in inches /43 200 gives Great Pyramid socket perimeter

• number of lunations in 4 320 000 sidereal years gives height of Great Pyramid in inches.

If we are open to looking at the evidence without preconceived ideas, we can see that it is not just the metre that is linked to the circumference of the earth, but the inch too. Indeed, the metre relates to the meridian circumference, as a spatial measure, and the inch relates to the equatorial circumference, as a spatial and temporal measure. As the earth spins on its axis fully in a day, a point on the equator can be thought of as travelling through space in a spiral motion, but also in a large ellipse, as it orbits the sun. In a sidereal year, this is a long path. And 365.256363 sidereal days, or turns of the earth on its own axis, multiplied by 4 320 000, gives the equatorial circumference of the earth in inches, to within 2.382 miles of the current estimate. There is no reason not to believe that the metre and the inch are as old as each other, and part of the same system.

When the earth's dimensions can be linked to the perimeter of the Great Pyramid, the moon's revolutions in a yuga of 4 320 000 sidereal years can be linked to the Great Pyramid height, and the length of a year can be linked to the earth's equatorial circumference by the number 4 320 000, the case that these numerical links are historical fact, and were deliberately designed, is strengthened.

We can also relate the megalithic yard to this, because the perimeter of the Great Pyramid, as 9068.8 inches, multiplied by 432/120,000, is 32.64768 inches, which is a megalithic yard. The metre and the megalithic yard are probably part of the same system because we can consider the megalithic yard as 12⁴ x 4/100,000 = 0.82944 metres. Similarly, the side of the Great Pyramid can be interpreted as 16 x 12 x 12/10 metres, so the perimeter is 10,000/9 megalithic yards. And we can think of the side of the Great Pyramid as 120,000 / 432 megalithic yards of 0.82944 metres, or simply as 120,000 / 432 x 12⁴ x 4 / 100,000 metres. Or 4 x 12⁵ / 4,320 = 12⁴ / 90 = 230.4 m. Metres therefore work well in the 432 system, and in the imperial system. We can interpret the side of the Great Pyramid as 12⁵ x 4,000 / (432 x 254) inches. We can also relate the side of the Great Pyramid to the height of the second pyramid in inches, with the number 4,320.

9068.8 x 4320 / (4 x 12³) = 5668,

or 12⁵ x 4,000 / (432 x 254) x 4320 / (4 x 12³) = 5,669.2914 inches,

which simplifies to 1,440,000 / 254 = 5,669.2914 inches, or 144 metres. The side of the Great Pyramid then connects to the height of the second pyramid by multiplying it by 90/144.

We can here also connect the number 28, since the height of the Great Pyramid, 5776, multiplied by 2π gives the perimeter of the Great Pyramid, which, divided by 3 and by 432 gives, 28.002838. This rounds up pretty well to 28, which is the number of days in a lunar cycle, because a sidereal month has 27.3216 days. 28 is a key number, and the height of the Great Pyramid is compatible with an interpretation of 2 / (28 x π) x 254)² = 146.6863 meters, or 1,000 / 28 x 254 x 2 / π = 5,775.0508 inches.

280 is also the number of cubits generally attributed to the height of the Great Pyramid. So we could think of a cubit as the number of sidereal months in 4,320,000 sidereal years multiplied by pi and 20 / 3, and divided by 432. This also almost equals 365.242199 / 354.36708 x 20. So we can also interpret the height of the Great Pyramid in inches as 432² x 354.36708 x 3 / (π x 400 x 27.321661) = 5,778.6336.

We could also interpret a cubit as (360 x 235 x 19 / (10⁶ x π))² x 2 = 0.52357 meters. This would therefore create an interpretation of 280 x (360 x 235 x 19 / (10⁶ x π)² x 2 = 146.6008 meters for the height of the Great Pyramid.

Dividing by 28: the Length of the Great Giza Rectangle (GGR)

   Let's look at the length of the Great Giza Rectangle. We saw that the height of the Great Pyramid can be interpreted as 280 Egyptian royal cubits. The number 28 returns here. The length of the GGR is 1000 000 / 28. The measure provided by Petrie is 35 713.2 inches. It's a close match, and it's expressed in inches. The number 28 is associated with the lunar month, in that the sidereal month is just over 27 days long so rounds up to 28. As a number of days, 28 is of course easy to divide up into 4 weeks of 7 days. Also, some divisions of the zodiac historically were divided in 28 parts, or lunar mansions (and some into 27). The Zodiac and cubit rod's divisions into 28 parts also allude to this equivalence.

Bailly shows in his History of Astronomy that dividing by 27 or 28 was a reference to the sidereal month, and this number was used to create 27 or 28 divisions of the zodiac in many ancient cultures.

The number 28 is also a perfect number.

There is another side to 28, that seems to be expressed here at Giza, in that it links up to the pi and the square root of three, which are found in the Great Pyramid, and elsewhere.

   The Great Pyramid is 5 776 inches high, and this can be interpreted as 280 cubits, of 20.62857 inches / 0.5239657 m. We can find the number 28 clearly displayed in inches in the length of the GGR, or at least 1 000 000 / 28, expressed in inches. The measure of 35 713.2 inches is close to 1 000 000 / 28 = 35,714.285714 inches, and as it's such a close match to the dimension given by Petrie, it should be taken as a possible interpretation.

   Just as the path of the sun along the zodiac was divided into 28 parts, here the north-south span of the site, from the north side of the Great Pyramid to the south side of the third pyramid, seems to represent a division by 28 also, in this case, of a million inches by 28. An Egyptian royal cubit is also divided into 28 parts, or 7 x 4.

    Why not simply make the rectangle 28 000 inches long? Possibly because the Giza design is based on a dynamic system, in which various elements are meant to be added, subtracted, divided or multiplied by each other, or by key numbers. Also, there fraction 1/28, has interesting connections to astronomical cycles, when these cycles are seen as numbers of days, and in conjunction with irrational numbers.

There is another possible reason for the 35 713.2 inches of the length of the GGR, linked to the motions of the planets. We think of the orbit of the seven traditional planets, Mercury, Venus, Earth, the moon, Mars, Jupiter, and Saturn, and add to this an eighth motion, the precession of the equinoxes, as more or less defining the motions of the sky. In Timaeus, and Republic, Plato refers to eight celestial motions, reflecting a concept of an ordered universe governed by precise mathematical proportions and geometrical harmony.

These are the orbital periods of the moon and planets known to antiquity defined in terms of earth years, and precession:

• Mercury: 0.24 years

• Venus: 0.615 years

• Earth: 1 year

• Mars: 1.88 years

• Jupiter: 11.86 years

• Saturn: 29.46 years

• Moon: 0.0748 years (adjusted to the Earth year cycle)

• Precession of the Equinoxes: 25,920 years

   The value of 0.0748 years for the Moon is derived by considering the synodic month, the time it takes for the Moon to return to the same position relative to the Sun as seen from Earth, and based on a sidereal year of 365.256 days. The result of all these numbers multiplied together is 187 974.1949 years.

If we start with the number 12, and divide it by all the orbital periods listed above, and precession, and then multiply it by 10¹¹, the result is 35 717.9240, just a few inches more than the length as given by Petrie, 35 713.2 inches. Or another way of understanding this is to read the length in English feet. With Petrie's value, this is 2 976.1 feet. And the orbit of the earth as 1 year, divided by all the other orbits listed, and precession, is 2 976.4937. And 1 000 000 / (2976.4937 x 12) = 27.9971, almost 28.

What if we include the Metonic cycle? If we divide 100 000 000 earth years by all these cycles: the planetary orbits, the sidereal month, precession, and the Metonic cycle, all expressed in earth years, we get very nearly 28 exactly.

1 000 000 / (0.24 x 0.615 x 1.88 x 11.86 x 29.46 x 0.0748 x 25 920 x 19) = 27.999364

   The Metonic cycle is a period of 19 years after which the lunar phases (synodic months) align closely with the solar year. It reconciles 235 lunar months with 19 solar years, a near-perfect synchronisation used by many ancient calendars to harmonise lunar and solar timekeeping.

   So could 28, which is a perfect number anyway, be Plato's perfect number of time? It does make sense to include the Metonic cycle, even though Plato doesn't mention it, because this cycle of 19 years reconciles the earth's year to the sidereal month and to the lunation, or synodic month. 19 years are approximately 6 939.6018 days, which is close to 235 lunations, or 6 939.6887 days, and close also to 254 sidereal months, or 6 939.7016 days.

   This might give further support to the reason why dividing a cubit ruler, or the zodiac, into 28 parts, may have been so important, and by extension, dividing by 7 more generally, as 28 is 4 x 7.

   Although Plato does not explicitly mention the number 28 in his works, it would have been well-known to him, especially through his connection to Pythagorean philosophy. The Pythagoreans regarded 28 as a "perfect number" because it is the sum of its divisors (1, 2, 4, 7, 14) and had a symbolic connection to harmony and cosmic order. Moreover, 28 was widely recognised in the ancient world as representing the approximate length of the lunar month, a critical timekeeping unit for calendars and religious practices. Given Plato's deep interest in numbers, mathematics, and their role in the structure of the universe, it is curious that he never specifically refers to 28 (as far as I know). However, it is likely that he would have viewed it as important, especially in the context of cosmic cycles, lunar periodicity, and the harmony of time. His omission of 28 may reflect a broader philosophical focus on different mathematical concepts, but the number’s resonance with Pythagorean and celestial thinking would certainly not have been lost on him.

   The Giza rectangle measures 1 000 000 / 28 inches in length, and 1 000 000 / 28 is very close to all the cycles in earth years multiplied together: Mercury, Venus, Mars, Jupiter, Saturn, Moon, precession, and Metonic, expressed in earth years. This could be key to understanding the site, looking for measurements expressed in inches,and interpreting them as units of time, as part of a sophisticated body of astronomical knowledge expressed in stone.

The √3 / π, or π / √3 equation

If we break this equation into two parts, we have on the one side √3 / π, a fraction reminiscent of the proportions and dimensions of he Great Pyramid (height as 10 000 / √3

inches, side as 5000 π / √3 inches.) And on the other side we are left with 3 / 29.53059, this last number being the number of days in a lunation, and this fraction comes up elsewhere at Giza, and 1 / 2.

So 1 / 28, or its rough equivalent 2 π x 29.53059 / (√3 x 3) is a key element in both the Great Pyramid dimensions and the Great Giza Rectangle dimensions. This can also be found elsewhere such as the Queen's Chamber. The mean floor length is given as 227.5 inches, or 5.7785 metres, and this works out as equivalent to 2 π / (3 x √3) x 29.53059 x 1.618034 / 10 = 5.777737 metres, or 227.47 inches. And just as the Great Pyramid height in inches can be interpreted as 10 000 / √3, the length of the floor in the Queen's Chamber can be interpreted as 10 / √3 = 5.773502 m. The same connections can be made to these other interpretations of the Great Pyramid height in inches: √3 x 365.242199 / 354.36708 x 2 000 x 1.618034 = 5777.032, also 366 x 27.32166 / √3 = 5 773.33272, or √3 / π x 300 000 / 29.53059 x 365.242199 / 354.36708 = 5 772.8127, or as Dennis Payne found, 346.6201 x 100 / 6 = 5 777.002: divide by 1 000 and then call it metres.

The height of the Great Pyramid in inches (and by extension the length of the Queen's Chamber in metres x 1000) can also be related to 200 x pi x Phi x 29.53059 / (3 x √3) = 5777.7225. So the 10 000 inches that are then divided by the square root of three are also linked to this equation: 200 x pi x Phi x 29.53059 / 3 = 10 007.31

The Mayan unit called a zapal is thought to be 57.75 inches long, and the connection between it and the Great Pyramid was made by David Kenworthy. (9)

The diagram below shows how some of the key dimensions at Giza can be linked to 2 π / (√3 x 3), whether multiplied by 29.53059 or not.

Amazingly, the equations 2 π x 29.53059 / (√3 x 3) and  π / √3 also connects key time cycles, in an approximate way.

An astronomical meaning to π and √3?

  The fraction π /  √3 can be considered lunar because it links key lunar and solar time cycles, as periods in days (see below).

Why 365 days and not 364 (13 months of 28 days), 365.25, 365.242199, or 366 days? Richard and Robin Heath observe in their work at le Ménec: "At this smaller scale of time, within a single day, the sun’s movement, per solar day, is 1/365th of the daily rotation of the circumpolar stars and therefore, for this purpose, the circumpolar region can be represented on the flat earth as a circle made up of 365 day-inches or multiples thereof." and "after a year of 365 days, the circumpolar stars will have rotated 366 times, the small amount of angular change per solar day is 1/365th of one complete rotation of the circumpolar stars. This unit of time will be called the chronon 8 and its duration is 3 minutes and fifty-six seconds. The 365 divisions of Le Menec’s forming circle were effectively counting time in units of 24 inches, each unit representing a chronon in the angular rotation of the Earth 9 . There are then 365 + 1 chronons in a solar day, that is 366 chronons."(12) It is therefore possible that a unit of 365 days was employed at Giza also, where similar principles of counting time through geometry were employed.

So: 365 x 346.6201 x 3 x √3 / (20 x π x 29.53059) = 354.30436

365 x 346.6201 / 354.30436 = 20 x π x 29.53059 / (3 x √3)

And also: 80 x 365.25 / 29.53059 x √3 / 360 x 254 ≈ 2000 π / (3 x √3)

80 years in days are approximately equivalent to: 2000 x π x 29.53059 / (3 x √3) x 360 / (√3 x 254) = 80 000 π x 29.53059 / 254 = 29 219.8692

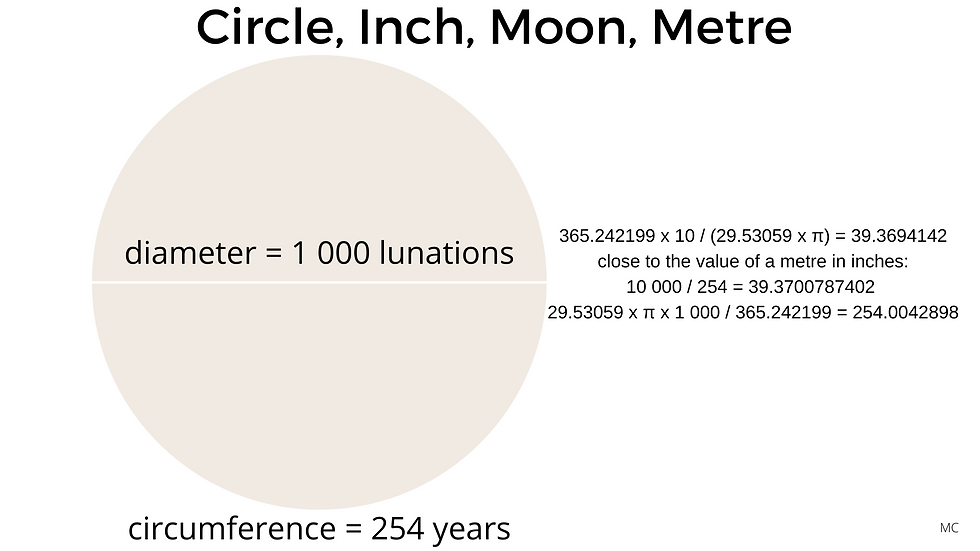
This works because of a coincidence with inches, metres, sun, moon and the circle. A circle with a diameter of 1 000 x 29.53059, or 1 000 lunations in days, will have a circumference of approximately 254 years in days. Or another way of putting this is to say a circle with a diameter of 29.53059 x 10 cm will have a circumference of 365.24836 inches, as 2.54 cm are an inch.

We can interpret the width of the GGR as 365.25 x 80 inches, or 80 000 π x 29.53059 / 254 inches, and the length of the GGR as 2000 π / (3 x √3) x 29.53059 inches. In this model, the ratio of the GGR's width and length is 120 x √3 / 254.

We can interpret the π / 2 ratio between the side and the height of the Great Pyramid as connected to the presence of pi in these interpretations of the length and width of the GGR. We can also connect the presence of √3 in the interpretation of the length of the GGR to the height in inches or metres of the Great Pyramid: 254 / √3 = 146.64697 m or 10 000 / √3 = 5 773.5027 inches. And we can interpret √3 and π in the Great Pyramid, the GGR, and elsewhere at Giza, as connected to expressions of time, such as:

• 1 year in days ≈ π x 29.53059 x 1 000 / 254 = 365.2487

• 80 years in days ≈ 80 000 π x 29.53059 / 254 = 29 219.8692

• 1 draconic year in days ≈ 20 π x 29.53059 / (3 x √3 x 354.36708 / 365 = 346.6815

• 1 civil year in days ≈ 354.30436 / 346.6201 x 20 π x 29.53059 / (3 x √3) = 365.0646

• 1 lunar year in days ≈  365 x 346.6201 x 3 x √3 / (20 x π x 29.53059) = 354.3044

What happens when we multiply the GGR length by 223 / 235? We get the perimeter of the second pyramid.

Taking the GGR length as 2000 π / (3 x √3) x 29.53059 = 35 708.3769 inches, the perimeter of the second pyramid becomes 2000 π / (3 x √3) x 29.53059 x 223 / 235 = 33 884.9704 inches, and the side 8 471.2426 inches. Breaking the numbers down, we can see the first part of the equation is 2000 π / (3 x √3), which is equivalent to 80 x 365.25 x √3 / (29.53059 x 360), 80 x 365.25 is the width in inches of the GGR, 2000 π / (3 x √3) x 29.53059 is the length (both approximately). And the result is the side of the second pyramid, also equivalent to 19 x 223 x 2 = 8474 inches, or days.

So we can say:

• GGR width (80 years as 80 000 π x 29.53059 / 254) x 254 / (120 x √3) = 35 708.3769, GGR length

• and GGR length (as 2000 π / (3 x √3) x 29.53059) x 223 / 235 x 1/4 = 8 471.2426, the second pyramid side, also equivalent to 223 x 19 x 2 = 8474 inches.

• and GGR length (as 2000 π / (3 x √3) x 29.53059) x 254 / 1 000 = 9 069.9277, the Great Pyramid side.

• and GGR length (as 2000 π / (3 x √3) x 29.53059) x 29.53059 / 254 = 4 151.5332, the third pyramid side.

If we take the perimeter of the Great Pyramid, in inches, it can be described as 20 000 π / √3, perhaps a reference to the astronomical connections above.

More irrational numbers: Pi and Phi in the Great Pyramid

If we look to the Great Pyramid, we can find more examples of irrational numbers being used to define space. One example is the side of the Great Pyramid. We've seen that it can be interpreted as 5 000 π / √3 inches. And because pi and Phi can be approximately connected by the fraction 5/6, we can also say that the side of the Great Pyramid is 6 000 x Phi² / √3 inches. Consequently, the length of the GGR can be interpreted as 6 x 10⁶ x 2.618034 / (√3 x 254) inches, or 600 x Phi² / √3 metres. And the third pyramid side as 6 x 10⁶  x 2.618034 x 29.53059 / (√3 x 254²) = 4 151.1707 inches, or 600 x 2.618034 x 29.53059 / (√3 x 254) metres. The third pyramid height as 6 x 10⁶ x 1.618034 x 29.53059 / (√3 x 254²) = 2565.5646 inches, or 600 x 1.618034 x 29.53059 / (√3 x 254) = 65.1653 metres.

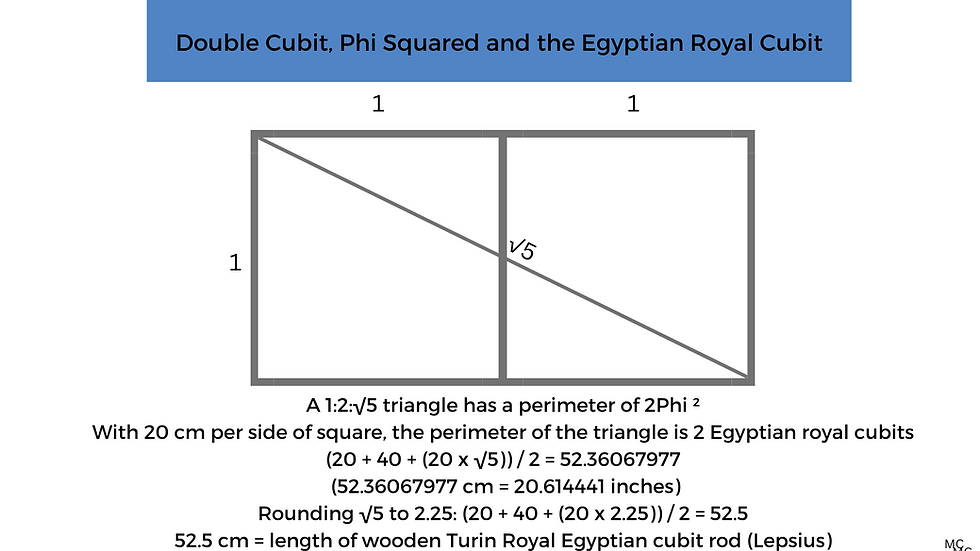
Another example is in the King's Chamber, in the Great Pyramid. The King's Chamber is essentially a 1:2 rectangle, or double square. As a result there is already an inbuilt link to the square root of 5, and to Phi squared, as the diagram below shows.

What can these dimensions tell us about the possible use of the inch, the cubit and the metre at Giza?

The perimeter of the King's Chamber offers intriguing insights into the possible intention of the architects: the perimeter as measured by Petrie is approximately 0.7 inches over an exact 2 000 x phi inches, with phi = 0.61803402. 60 cubits of 20.614125 inches measure 1236.847 inches, which is close to the perimeter measured by Petrie. 1236.8475 inches would be the circumference of a circle with a diameter of 10 metres.

If we take as a starting point the cubit which is linked to Phi, and measures Phi² x 20 centimetres, this can be said to be generated by a double square, each square having sides of 20 cm, because the perimeter of the triangle with sides 20, 40, and 20 x √5 cm is equivalent to 2 Egyptian royal cubits of 52.36068 cm. A perimeter of 2 000 x 0.618034 inches, as we see in the King's Chamber, is equivalent to 254 / 1.618034³ such cubits.

Egyptian royal cubit of 52.36068 cm = 20.6144409 inches

20.6144409 x 254 / 1.618034³ = 1 236.068 inches.

Or we can say that the perimeter is 2 / 10 x 254 / 1.618034 = 31.396126 metres. This is close to 10 x pi metres, though there is a 1.98 cm difference. Almost exactly equal to 31.396126 metres is 29.53059 x 354.36708 x 3 / 1 000 = 31.39401 metres, with 29.53059 the number of days in a lunation and 354.36708 the number of days in 12 lunations, or a lunar year. We can therefore say that 29.53059 x 354.36708 x 3 / 1 000 is approximately equal to 2 / 10 x 254 / 1.618034. Or that Phi is approximately equal to 200 x 254 / (29.53059 x 354.36708 x 3) = 1.61814324.

The use of the inch and the metre

  The use of lunar cycles in the dimensions themselves, represented by values such as 354.36708 (the average number of days in a lunar year) and 29.53059 (the average length of a synodic lunar month, or lunation), reflects the astronomical patterns we can see in the proportions at Giza. And if this reading is valid, then we can put forward the theory that the architects used the modern inch and metre as well as the royal cubit. Here are three indications that this may well be the case.

1. As we've seen, the width of the Giza site as drawn out by the layout of the three main pyramids, i.e. west side of third pyramid to east side of great pyramid (Great Giza Rectangle or GGR) measures 29 227.2 inches according to Petrie, which is close to 80 x 365.25 = 29 220 inches, and could represent 80 years. The 8 year cycle was key to may ancient astronomical systems.

2. A length of 10 000 inches is assumed, indirectly, to exist, in the height of the great pyramid, as it is 10 000/ √3 = 5 773.503 inches. A length of 1 000 000 inches is assumed, also indirectly, to exist in the length of the site, the Great Giza Rectangle (north side of the Great Pyramid to south aide of the third pyramid), as it measures 1 000 000/28 = 35714.2857 inches. By comparison Petrie gives 5776 inches for the GP height and 35713.2 inches for the GGR length.

3. The side of the Great Pyramid can also be said to be based on the inch. The diagram below shows that a circle with a radius of 1000 x Phi squared inches fits inside an equilateral triangle with sides equal to the GP.

The value of the circumradius, here in inches, 5236, is equivalent to the value of an Egyptian royal cubit in metres.

Linking the King's Chamber diagonal to other aspects of the Giza site, and to  π / √3

If we consider the King's Chamber's diagonal in relation to the distance between the centres of the Great Pyramid and the third pyramid, which is the diagonal of the Metonic Rectangle, there is a connection. The King's Chamber diagonal is close to being 80 times smaller than the Metonic Rectangle diagonal, and this connection is closest if we calculate the diagonal based on the south and west mean dimensions, which as slightly smaller than the north and east ones.

It is curious how we find a number expressed in metres in one places and in inches elsewhere. For example, in the King's Chamber, the diagonal in inches, as 460.715, is the same number of metres in two sides of the Great Pyramid. One side of the Great Pyramid is 9068.8 inches or 230.34753 metres. Multiplied by 2 this is 460.69504 metres. Or if we take the length of the Great Giza Rectangle (GGR) in metres and multiply it by 10, we get 9 071.15281, the side of the Great Pyramid in inches. Consequently, the length of the GGR x 254² x 2 / 10⁷ is 460.81456, the King's Chamber diagonal in inches.

Another example is the height of the Great Pyramid in inches, 5776. The mean floor length of the Queen's Chamber is given as 227.5 inches, as converted to metres this is 5.7785, almost exactly 1000 times smaller than the Great Pyramid height in inches.

Is the Egyptian Royal cubit the best way to read the length, width and diagonal of the King's Chamber in? The length and width of the chamber are in 2:1 ratio, which means the diagonal will be the width x √5. But when we divide the length by 20 and the width by 10, we obtain:

Length (N) 412.4 inches = 20.62 x 20

Length (S) 412.11 inches = 20.6055 x 20

Width (W) 205.97 inches = 20.597 x 10

Width (E) 206.29 inches = 20.629 x 10

The average value of sub-unit deduced from these measures: 20.612875 inches. The south and west values, added together and divided by 30 give 20.6026667 inches, which could seem a little on the small side for a royal cubit. One way to interpret the dimensions of the chamber is with 2 π / √3 x 254 / 100 x √5 = 20.603356. The length is indeed close to 20 x 2 π / √3 x 254 / 100 x √5 inches and the width is close to 10 x 2 π / √3 x 254 / 100 x √5 inches. The diagonal is then 10 x 2 π / √3 x 254 / 100 x 5 = π / √3 x 254 = 460.7050 inches. Multiplied by 80 this gives 36 856.4031 inches, close to the 36 857.7 given by Petrie, only just over an inch difference in fact, and this in turn multiplied by 1 000 / (16 x 254) is 9068.9968, pretty much exactly Petrie's estimate for the side of the Great Pyramid (9068.8 inches).

As a result, we can interpret the width and length of the King's Chamber as 10 x 20 cubits of  π / √3 x 254 / (10 x √5) inches (or π / √3 x 254² / (√5 x 100 000) metres. However this cubit doesn't then quite match the Phi cubit seen above.

 π / √3 x 254 / (10 x √5) x 254 / 2 0000 = 2.616626, a difference of roughly 0.001408 from Phi squared.

Which cubit works best? They both have advantages, and the Phi cubit an be linked to other parts of Giza.

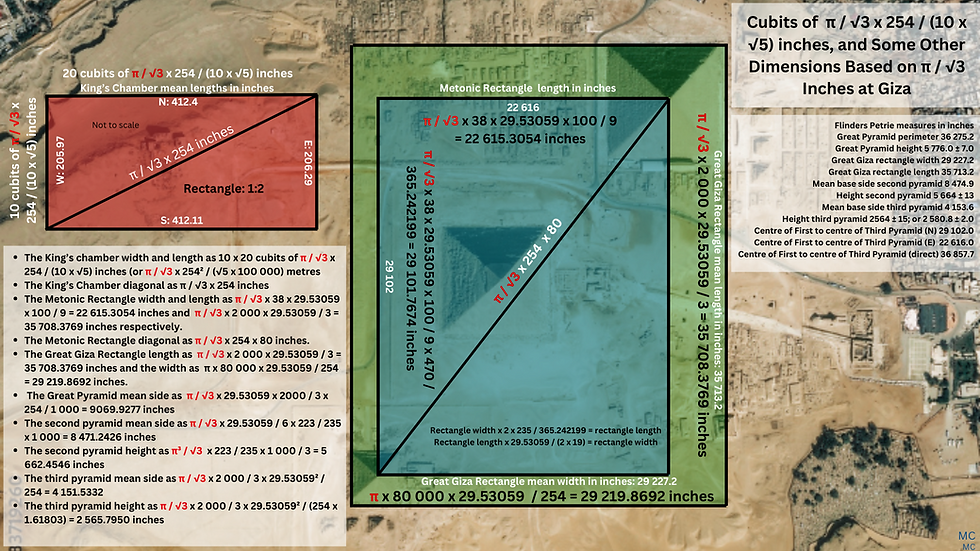
If we go back to the side of the second pyramid, given in inches by Petrie as 8474.9, this is also close to Phi³ x 2 000 = 8472.1361 inches. As a result, since the Egyptian royal cubit can be understood as 0.2 x Phi² metres (0.5236068 m), then the side of the second pyramid can be interpreted as Phi x 254 = 410.980636 Egyptian royal cubits of 0.5236068 m / 20.614441 inches. By contrast, in this case, Phi x 254 cubits of 2 π / √3 x 254 / 100 x √5 = 20.603356 inches falls short of Petrie's measure.

Since we can interpret the length of the side of the second pyramid as Phi³ x 2 000 = 8 472.1360 inches and π / √3 x 29.53059 / 6 x 223 / 235 x 1 000 = 8 471.2426 inches, we can say that the ratio between the number of lunations in a Saros cycles (223) and the number of lunations in a Metonic cycle (235) can be approximated by Phi³ x 8 x 3 x √3 / (2π x 29.53059).

We can also approximate the number of days in a Metonic cycles and Saros cycle with geometry in the following way:

We can even use the dimensions of the site to calculate the number of days in a Metonic or Saros cycle, by simply dividing the length of the Great Giza Rectangle by the perimeter of the second pyramid, or vice versa, and then multiplying by 29.53059, and then either 223 or 235, as the diagram shows.

Another interpretation of the side of the second pyramid in inches is:

365.242199 / 354.36708 x 10 x (223 / 235)² x 29.53059² x π / 3 = 8 475.6779

Here we can see the ratio between the solar and lunar years, and the ratio between the Saros and Metonic cycles, combined with a lunation and pi.

So Phi³ is approximately equal to 365.242199 / 354.36708 x (223 / 235)² x 29.53059² x π / 600.

And Phi is approximately equal to 365.242199 / 354.36708 x (223 / 235)² x 29.53059² / 500 .

We can interpret the Great Pyramid side and height in terms of 354.36708 / 360, 365.242199 / 19, and 223 / 235, ratios seen elsewhere at Giza.

Let's return to the Great Giza Rectangle, this time with units of measure in mind instead of proportions.

Cycles of time expressed in the width of the Great Giza Rectangle (GGR)

We've seen that astronomical interpretations are a good fit to the proportions at Giza, are these astronomical interpretations reflected in any way in any particular unit used at in the Great Giza Rectangle? Let's start with the dimensions as given by Flinders Petrie, which happen to be in inches. We saw in the beginning that by coincidence, the width of the GGR expressed in inches was close to the number of days in an 80 year period.

If we start out with the width of the GGR as 80 years, expressed in inches, then we can interpret other aspects of the site as related to this period of time, as the diagram above shows.

How can we interpret the dimensions of the GGR in other units?

   There are in fact many possibilities that resonate with an astronomical interpretation. For example, the width can be interpreted as 10 Venus cycles of 8 years, which of course brings us back to the 80 years expressed in inches which seem to characterise the width of the GGR. And if we look at the dimensions in metres, this opens up two other cycles: the 60 or 600 year period, and the 24 000 year period, both associated with ancient India and the Chaldeans, among others. In fact, Àryabhata states: "12. The planets moving equally (traversing the same distance in yojanas each day) in their orbits complete the circle of the asterisms in sixty solar years, and the circle of the sky in a divine age [caturyuga]."(15)

24 000 years are 8766 000 days, which, multiplied by 2 and divided 600 gives the number of days in 80 years. The average number of lunations in a solar year is also close to 29220 / (60 x 39.37001787402). We can also simply interpret 80 years as 24 000 / 300 years.

The width in metres divided by 60 gives  approximately the average number of lunations per year: 12.372848.

The width of the GGR can also be interpreted as 360 x 365.242199 / 354.36708 x 2 = 742.0959737 metres. We saw earlier that 360 / 354.36708 = 30 / 29.53059 = 1.01589572, which is close to 254 x 4 / 1 000 = 1.016.

According to this interpretation, units such as the Roman or Saxon feet, metres or megalithic yards, would be parts of a mathematical and astronomical system, designed to express a particular aspect of the system alongside other aspects of the system expressed in different units. Here we can see that the width of the rectangle has different associations depending on what unit the length is read in.

Expressing time cycles with the inch, and the metre

  There is another example of the day-inch possibly being used at Giza in the outer-perimeter of the Great Pyramid. he outer perimeter, running along the socket sides, is 4 x 9125.9 inches. The mean side of 9125.9 corresponds to the number of days in a 25 year cycle, with 365 days per year, approximately equivalent to 309 lunations. 365 x 25 is 9125. Four times this 25 year cycle is 36 500 days long, which is almost exactly 1236 lunations long also. This is 2 x 618, which offers a connection to phi, as Gulya Priskin has observed. Therefore the base perimeter is equivalent to 100 years of 365 days, which is approximately 2 000 x phi lunations. Here the astronomical value corresponds to the actual measure in inches.

More often, it seems units of time are combined with other units of time or numbers derived from geometry. Both the side and the height of the Great Pyramid can be linked to the mean number of lunations per year. 12.368266 x 4400 / 3 π = 5774.18074 Great Pyramid height in inches, and the side is then 12.368266 x 2200 / 3 inches, or 300π / (√3 x 44) inches.

Why do we have 280 cubits in the height of the Great Pyramid and 440 in its side?

We've seen that 28 / 10 000 is a good approximation of 3√3/ (20 x π x 29.53059). There are 280 Egyptian royal cubits in the height of the Great Pyramid. So the height of the Great Pyramid can be interpreted as 3√3/ (20 x π x 29.53059) x 2 000 000 x 365.242199 / 354.36708 = 5772.81266 inches, which simplifies to √3 /π x 300 000 / 29.53059 x 365.242199 / 354.36708, and if multiplied by √3 is 9 998.8048, close to 366 sidereal months of 27.32166 days. 

   The mean number of lunations per year multiplied by 440, as a measure in day inches, can be related to various parts of the Giza site, as the image below shows. But since 44/100 multiplied by the average number of lunations in a year is also close to π x √3, we can substitute one by the other. For example, the Great Pyramid height in inches can be interpreted as 12.368266 x 4400 / 3π = 5774.18074, or 1 000 x π x √3 / (3π) = 5773.5027. And the Great Pyramid base can be interpreted as 12.368266 x 440 / 6 = 9070.06173 inches, or 1 000 x π x √3 / 6 = 9068.9968 inches.

   In the table above we can see that often the number of lunations per year is multiplied by 440 or 4400. As 12.368266 x 440 is about equal to π x √3 x 1000, one can be substituted for the other.

There are 235 lunations in a Metonic period, and also 254 sidereal months. The side of the Great Pyramid can be interpreted as 254 × 29.53059 × 2π /(3 x √3 ) = 9,069.9277 inches. The height is then 254 / √3 x 29.53059 x 4/3 inches, and since 4/3 x 29.53059 inches is close to a metre, we can also say that it is 254 / √3 = 146.6470 metres high. There are 10 000 / 254 inches in a modern metre. 254 x 29.53059 x 4/3 = 10 001.0265

The side of the second pyramid can be understood as simply 223 x 38 = 8 474 inches, 223 being the number of lunations in a Saros period (Halley) and 38 being related to the Metonic period, as 365.242199 x 38 / 470 gives approximately the number of days in a lunation.

·Or as the diagram above shows, the side of the second pyramid can be interpreted as 100 π x 223 x 29.53059 / (47 x 3 x √3) = 8474.2426 inches.

Curiously, another link can be made between these two types of lunar month, and a solar year, and it is to the Metonic period, in days, which is 19 years or 235 lunations, which are 6 939.68865 days. This period is almost equal to 27.32166 x 29.53059 x π x 1000 / 365.242199 = 6 939.8187 days. So the sidereal month can be defined, approximately, as 235 x 29.53059 x 365.242199 / (29.536059 x 1000 x π), simplified to 235 x 365.242199 / (1 000 x π) = 27.31148, or 365.242199² x 19 / (29.53059 x 1 000 x π).

     If we return to the Metonic rectangle seen earlier, and consider the dimensions, these are also of interest.

• Metonic Rectangle Width

The 22 616 inch side is approximately equivalent to 7200π = 22 619.4671 inches, or to 1900 / 3 x 2π x 29.53059 / (√3 x 3) = 22 615.3054. This is interesting as a lunation in days multiplied by 4/3 is, in inches, approximately a metre, and when this is multiplied by pi and divided by 6, you would expect to obtain a cubit. So here we have 29.53059 x 4/3, a metre, x  π/6, a cubit, and then x 1 900 / √3, the width of this rectangle. (Substituting the 254 for the 29.53059 x 4 / 3, we get 1 900 / 254 x π / √3 x 10 000 / 6 = 22 612.9842.

Curiously, 19 000 / 254 x π = 235.001025. This is because 365.242199 / 29.53059 x 254 / 1 000 = 3.1415396, which is approximately equal to pi. So to a good degree of accuracy, 235 x 254 / 19 000, so number of lunations in a Metonic period x number of sidereal months in a Saros period / number of years in a Metonic period and divided further by 1 000, gives pi, approximately.

If we replace pi by 235 x 254 / 19 000, we can get a rectangle width of 235 / √3 x 1 000 / 6 = 22 612.8855.

Elsewhere, the  π / √3 and 2π x 29.53059 / (√3 x 3) themes return here too. (The GGR length is 2 000π x 29.53059 / (√3 x 3) inches long, the Great Pyramid side is 254 x 2π x 29.53059 / (√3 x 3) inches, and the Metonic Rectangle diagonal is 254² x 16 x 2π x 29.53059 / (√3 x 3000) inches)). The width can be defined also as 235 x 19² x 2 000 / (29.53059 x 254) = 22 620.3447, though this is perhaps a bit long, as Petrie gives 22 616 inches.

• Metonic Rectangle Length

The 29 102 inch side is approximately equal to 29.53059 x 20 π / (√3 x 3) x 470 x 190 / (3 x 365.242199) = 29 101.7564078. Here we can see the core element seen elsewhere: 29.53059 x 20 π / (√3 x 3), and it is multiplied by 190, the number of years in 10 Metonic cycles, and 470, which is the number of lunations in 2 Metonic cycles (235 x 2), and divided by the number of days in a solar year of 365.242199 days.

• Metonic Rectangle Diagonal

The diagonal of this Metonic rectangle is of interest also. The diagonal is 36 857.7 inches, which is close to the perimeter of the Great Pyramid x 4 x 254 / 1 000, so 9 068.8 x 4 x 4 x 254 / 1000 = 36 855.6032, two inches under Flinders Petrie's measure. 254 is the link between a metre and an inch, as 2.54 cm are an inch, and it's also a link between the solar year and lunation, as 29.53059 x π x 1 000 / 365.242199 = 254.0042898. As a result, the Great Pyramid base perimeter of 9068.8 x 4 inches multiplied by 354.36708 x π and divided by 3 and 365.242199 is 36 856.2254 inches, just over an inch under the Metonic rectangle diagonal as measured by Flinders Petrie. Another way of expressing this is 2000 π / (3 x √3) x 354.36708 / 365.242199 = 36 857.0252 inches. In a similar way, the base side of the Great Pyramid can be expressed in inches as 5000 π / √3, the second pyramid base side as 14 000 π / (3 x √3), and the third pyramid base side as 29.53059² x 864 x √3 / 100 π. So this diagonal fits in with the use of pi and the square root of three elsewhere. And we've seen it is 80 times the diagonal of the King's Chamber.

   Another way of interpreting the diagonal, though it's a less precise match, is 6 x 6 x 7 x 8 x 8 x 9 x 254 / 1 000 = 36 868.608. Equally, if we consider the side of the Great Pyramid to be 9 072 inches, then the perimeter can be 9! / 10 inches. This has important consequences in historical metrology. 9! / 14 = 25 920, and 9! / 12 = 30 240, and these numbers are linked to values in inches of units such as the vara, the Persian foot, etc.

We can also give an astronomical interpretation to the two smaller rectangles which link the centres of the three main pyramids.

The perimeter of the Great Giza Rectangle is interesting if we think of it as equivalent to the circumference of a circle: this circle would have a diameter of 29.53059 x 1 400 inches. Dennis Payne has observed that the sum of the heights of the three main pyramids at Giza can be thought of as equal to 1 400 (depending on the values chosen for these heights.) For example if we take 5774, 5665 and 2561, inches for the main three pyramids, that gives a total of 14 000 exactly. Petrie gives, in inches, 5 776 for the Great Pyramid, 5 664 for the second pyramid, and 2 564 or 2 580.8 for the third pyramid, with various margins of error. So we could think of the Great Giza Rectangle perimeter as a circle with a circumference based on a lunation and the heights of the three main pyramids, which is represented by a rectangle.

How can we find patterns in interpreting the dimensions at Giza? One way is to interpret the key dimensions in terms of the variations on the same numbers. The three diagrams below show that this can be done in several ways, partly because of equivalences shown in the middle diagram.

We can see in the diagrams above that many measurements at Giza, when expressed in inches, including the side of the Great Pyramid can be understood as incorporating the ratio between the Metonic and Saros cycles (in red, as 235 / 223). Also apparent is the importance of Phi, often cubed, and of 254. What this diagram shows is that we can create a model made up of measures in inches that incorporate pi, Phi, the Saros and Metonic cycles, lunations and lunar years, which matches the dimensions given by Flinders Petrie fairly well. The inch is not, of course, the only significant unit. Where the inch is divided by 254 and multiplied by 10 000, we have a metre, and where the inch is divided by 254, divided by 1000 and multiplied by 2 and Phi², we have an Egyptian royal cubit (one particular type of cubit, among others).

How can we calculate the number of days in a Metonic cycle? We can multiply 29.53059 x 223, or we could multiply the side of the Great Pyramid, in inches, by a lunar year in days, divided by 360, and multiply by a lunation in days and the number of months in a Saros cycle, and then divided by Phi cubed and 2 000.

Expressing these time cycles is not limited to the inch. In metres, the side of the Great Pyramid is 254 π / (2 x√3) metres. But we can also think of it as √2 / √3 x 100 000 / 354.36708 = 230.409828 metres, equivalent to 9071.253087 inches. If we understand the megalithic yard as √2 / √3 x 40 = 32.65986 inches, as put forward by David Kenworthy, then we can also interpret the side as 10⁸ / ( 4 x 254 x 354.36708) = 277.749267 Megalithic Yards. This is close to 2500 / 9 Megalithic Yards, as 354.36708 x 254 = 90 009.23832, very close to 90 000.

We can also interpret the side of the Great Pyramid as √2/√3 x 100 000 / 354.36708 = 230.40983 metres, equivalent to 9071.2531 inches. A lunar year is 354.36708 days. An inch is 2.54 cm. The existence of the metre owes a lot to the number 254, in its role with the inch as "day-inch". A lunar year can be represented geometrically as 200 000 x √2 / (254 x π) = 354.45524, which basically means that 20 x √2 /π metres are very nearly equal to a lunar year in day-inches. This is especially interesting when 20 cm x 2.61803, which is Phi squared, make a royal Egyptian cubit, as 52.3606 cm (20.6144 inches), and also, if we were to add a value of 2.61803 cm to this cubit in cm, we would obtain a nice approximation of pi, 3.141636. Furthermore, if we were to multiply that cubit as a value in inches by the number of days in a lunar year and divide by 20, we would obtain a nice approximation of the solar year in days: 20.6144 x 354.36708 / 20 = 365.2534. This gives a strong indication that the metre and the inch are both intimately connected to the Giza plan, and to each other.

The base side as 230.338602 metres is 29.53059 x 3 x 364 / 140 metres. The 364 being sometimes given as the number of days in a year, as per the Book of Enoch, because this is exactly 13 months of 28 days, and 10 days more than a lunar year approximated to 354 days. Coincidentally, 230.34 is the number of inches in the height of the King's chamber, as pointed out by Dennis Payne, and so the same lunar associations can be made there, but in inches instead of metres.

Another interpretation of the base side is with a value of 9067.5311 inches, equivalent to 365.242199 x 12 x 223 x 29.53059 x π / 10 000. This brings in 223, the number of lunations in a Saros cycle (Halley's Saros of 223 lunations, not the Babylonian Saros).

Another interpretation is this: the 600 year period known to the Chaldeans and ancient Brahmins is 7420.9597 lunations of 29.53059 days. So that, approximately, 7421 x 29.53059 = 600 x 365.242199. The 7421 days, multiplied by 11 / 9 are 9070. As a measure in inches, 7421 x 11 / 9 = 9070.1111, or 11 / 9 x 7420.9597 = 9070.06, very close to the base side of 9068.8 inches. The 11 / 9 fraction can also be seen in the ratio between the width and length of the great Giza rectangle. Base = 7421 x 11/9 =9070.111 inches. As we will see, the width of this rectangle in meters is also close to 742.1 (or 7,420.9597 x 1.0002766 / 10 = 742.3012 m, using the coefficient 1.0002766 to bring the astronomical values closer to Flinders Petrie's measurements).

The Planets

Venus

We've seen that Venus is represented by the width of the GGR, which represents 80 years, so 10 cycles or 8 years. The width of the Great Giza Rectangle is 29 227.2 inches and this is very close to 80 x 365.25 = 29 220 days.

A connection to a Venus day can also be found by combining the sides of the Great and third pyramids. If the values for these measures are given in metres, then the resulting rectangle has an area of 24 300 square metres. A Venus day lasts for 243 earth days.

Mars

We can find Mars numbers in the Great Pyramid, as well as the distance between the centres of the Great Pyramid and the third pyramid:

   The synodic orbital period Mars is 779.94 days (close to 780). With 779.94 / 10, we get 779.94 / 10 x 29.53059 = 230.32088, which is the value of the base side in metres, equivalent to 9067.75132. Or rounding the Mars period up, we get 780 / 10 x 29.53059 = 230.3386 for the measure in metres, equivalent to 9068.4489 inches. The metre seems to express lunar cycles in conjunction with other cycles, such as Mars, here, or in the Great Giza Rectangle, Venus. The sidereal orbital period for Mars is 686.980 days. If we take 9068.4489 inches for the Giza base side, this is 13.2 x 687, that is 687 Saxon feet. So we can think of the ratio between the metre and the Saxon foot in terms of Mars and the Moon, as approximately 687 x 29.53059 x 100 / 780.

The number 687 is close to 6876, an important number in ancient geometry, as a circle with a diameter of 6876 units will have a circumference which divides almost perfectly into 21 600 units, or 6³ x 100. This lends an extra dimension to the sidereal orbital period for Mars, and 687 x π / 2 160 = 0.999201. Dividing circles into 360 degrees and then further into minutes (360 x 60 = 21 600) is fundamental to practical geometry and geography today, as well as time keeping. So it's curious that a diameter very close in value to 10 Mars sidereal orbital periods should be so useful in dividing a circumference that way.

6876 x π = 21 601.591.

We can also interpret the width and length of the King's Chamber as 687 / 10 x 3 = 206.1 inches and 687 / 10 x 6 = 412.2 inches respectively. The Mars interpretation fits the measurements made by Petrie better than the 21 600 and pi interpretation, whereby the length of the chamber would be 21 600 / π x 6 / 100 = 1 296 / π = 412.5296, but it is still a possibility. The width would then be 648 / π = 206.2648 inches.

We might also interpret the side of Great Pyramid in inches as 24 x 378 = 9072, with 378 being the number of days in Saturn's cycle. Or we could read the side of the Great Pyramid as 2 x 378 feet. Since we can interpret the side of the Great Pyramid also as 29.53059 x 100 x 780 / 254 = 9068.45 inches, that is, 100 lunations in days multiplied by 780, the number of days in a Mars cycle, divided by 254, then we can say that, approximately, a Mars cycle of 780 days is equal to 24 x 378, the number of days in a Saturn cycle, multiplied by 254 / (100 x 29.53059).

Mercury

Mercury is also represented in the numbers: the side of the Great Pyramid can be interpreted in relation to the number 88, which is the period in days of the sidereal orbit of Mercury. And the 440 cubits often given to the side of the pyramid can be thought of as half of 88.

Expressing time cycles with the Egyptian royal cubit and the megalithic yard

Interesting connections between aspects of the site when measured in cubits can be made, as the diagram below shows. If you multiply the side of the Great Pyramid in Egyptian royal cubits by the side of the third pyramid, also in royal cubits, an rectangle with an area equivalent to 3000 x 29.53059 square royal cubits is formed.

Looking at various aspects of Giza can help to come up with different ideas of how the cubit could be defined. However, there are different values for the cubit, for example the cubit derived from the solar and lunar years in inches, or pi and Phi squared in metres, fits well in the side of the Great Pyramid. This is slightly smaller than the cubit we saw in the diagram above, which also fits, but if we try to link the larger cubit of 20.6193454 inches to the average number of lunations per year, then the side of the Great Pyramid becomes 9071.4468 inches.

Below are some possible values for an Egyptian Royal Cubit.

We can also think of the megalithic yard in terms of some of the ratios found at Giza. For example, it can be 40 x √2 / √3 inches, or 12⁴ x 4 metres, or 2 π / √3 x 9 inches. We can think of the perimeter of the Great Pyramid as 20 000  π / √3 inches, and if the megalithic yard is 2 π / √3 x 9 inches then the perimeter is then 10 000 / 9 megalithic yards.

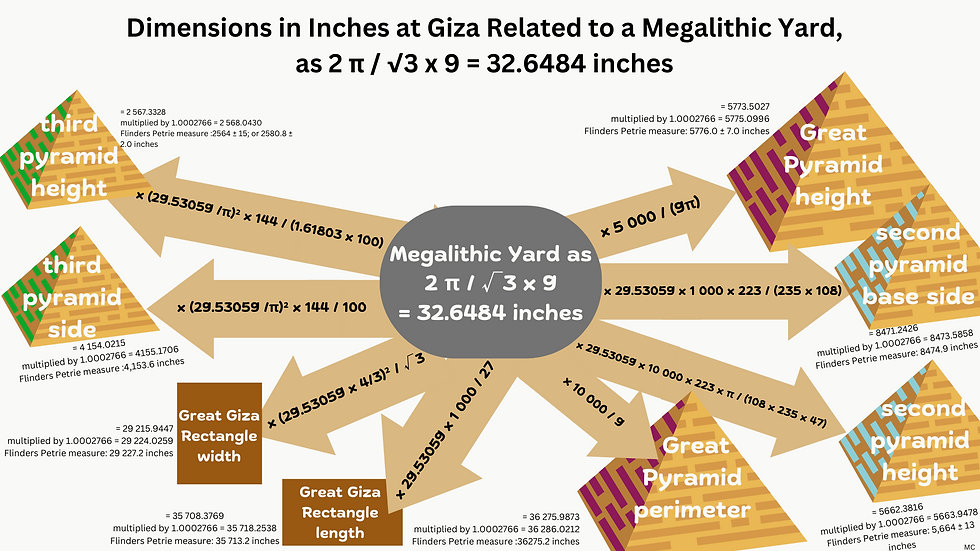
We could even define the ratio between the  2 π / √3 x 9 inch megalithic yard and the π / 6 metre cubit as 108 / √3 x 254 / 10 000, which is interesting because 108 is an important number in ancient metrology. So 108 / √3 x 254 / 10 000 cubits are a megalithic yard. And then the perimeter of the Great Pyramid would be equivalent to 12 x 254 / √3, or 4 x 254 x √3 Egyptian royal cubits. This resonates with the ratio 4 x 254 that we find linking the Great Giza Rectangle length to the perimeter of the Great Pyramid, and this perimeter to the distance between the centres of the Great and third pyramid.

35 713.2 (GGR length inches) x 254 x 4 / 1 000 = 36 284.6112

36 284.6112 (GP perimeter inches) x 254 x 4 / 1 000 = 36 865.1650 (Distance centres GP - G3 inches)

The number 108 appears in connection with a measure in megalithic yards in the Great Pyramid base: the diagonal of the square base, which is the side multiplied by √2, is equivalent to 108 x 9 x 64 / 100 Egyptian royal cubits of 20.616673 inches. Or this diagonal can be interpreted as 10 000 / 36 x √2 megalithic yards.

The height of he second pyramid can be thought of as expressing half a draconic year in megalithic yards if we take into account the error margin given by Petrie. 173.31 x 2 π / √3 x 9 = 5 658.2922.

We can also think of the length of the Great Giza Rectangle as expressing 5 draconic years in Egyptian royal cubits, the width as 48 lunar months in royal cubits, and the side of the second pyramid as 411 royal cubits (411 days is a lunar cycle).  

The megalithic yard can be connected to the draconic year via the square.

The links between these three units, inch, metre and cubit, and to astronomy and geometry, may hint at something of the symbolic and religious significance of the cubit in ancient Egyptian cosmology and astronomy. It could also explain some of the enduring appeal of the metre and the inch.

The main factors at Giza: π , √3, 223, 235, 254 and 29.53059

The same numbers crop up in the ratios between elements of the Giza plateau, often  π , √3, 223, 235, 254 and 29.53059.

254

A centimetre is 2.54 inches. At Giza, the number 254 is found as a factor which links various linear dimensions. What does it mean to multiply or divide a linear measure by 254? What does it mean to convert inches to metres, or vice versa?

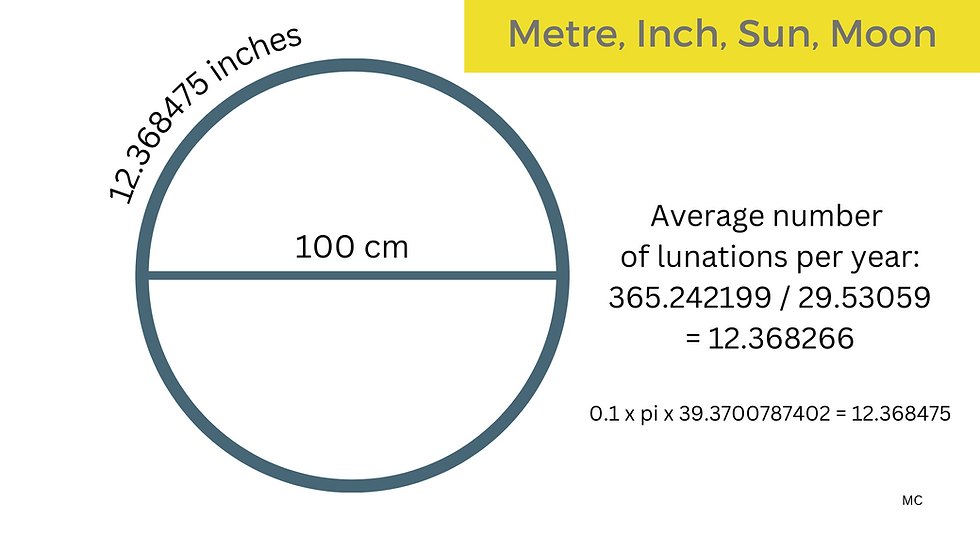
   As 29.53059 x 4/3 inches are close to 10 000 / 254 inches, as are 365.242199 / 354.36708 x 100 / 2.61803 inches, they can be used almost interchangeably to interpret the dimensions at Giza.

10000 / 254 = 39.3700787402

29.53059 x 4/3 = 39.37412

365.242199 / 354.36708 x 100 / 2.61803 = 39.368871

 The side of the Great Pyramid can be interpreted as 254 × 29.53059 × 2π /(3 x √3 ) = 9,069.9277 inches. The height is then 254 / √3 x 29.53059 x 4/3 inches, and since 4/3 x 29.53059 inches is close to a metre, we can also say that it is 254 / √3 = 146.6470 metres high

  The number 254 is linked to both astronomy and geometry. It is linked to pi, to Phi squared, and to the solar and lunar years, as well as being the number of sidereal months in a Metonic period. We often find the number of days in a lunation divided by 3 or 30. Why? 30 / 29.53059 is very close to 254 x 4 / 1 000. and indeed a metre in inches is very close to 4 /3 x 29.53059.

For example the length of the Great Giza Rectangle multiplied by 30 / 29.53059, gives the perimeter of the Great Pyramid, which is almost equivalent to multiplying the length by 254 / 1000 (ratio between metre and inch) to obtain a side of the Great Pyramid.

  The number 29.53059, which is the number of days in a lunation, comes up again and again. Why is it used as a factor to multiply things by? By a strange natural coincidence, 29.53059² x 25 x pi / 365.242199 = 18.99999274, almost 19, the number of years in a Metonic cycle. And 29.53059 x 25 / pi = 234.99696855. 235 is the number of lunations in a Metonic cycle. The Metonic cycle comes up simply by squaring this lunar number, 29.53059, and multiplying it by pi and 25, or by pi and 100 / 4.

   When linear dimensions at Giza are multiplied or divided by 254, it's not necessarily that in one place inches were used and in another metres were used. The metre and the inch can both be said to co-exist at Giza, and even if the metre hadn't appeared in the 18th century it would still appear at Giza. But the use of 254 could in fact be a reference to the ratio between the solar and lunar years combined with Phi squared, or to pi divided by the mean number of lunations in a year.

   If we think of possible links between the metre, the inch and the Egyptian royal cubit, the number 254 also makes an appearance, since 2.54 cm are an inch. Converting between inches and metres allows us to see ways of thinking about the Egyptian royal cubit, in relation to geometry and astronomy.

  Another interesting connection to be made to the number 254 is that there are 127 kerb stones around the base of Knowth, in Ireland. As Howard Crowhurst points out in his book Carnac the Alignments:

The presence of the number 127 in Ireland seems to suggest a link to the repeated presence of 254 at Giza. The 127 stones at Knowth also suggest we should take the inch - metre connection seriously when analysing ancient sites.

254 x 4 or 360 / 354.36708?

The number 254 is often found together with 4 as a ratio, and the following extract from Bailly's Treatise on Indian and Eastern Astronomy, quoted earlier in relation to the proportions at Giza, may shed some light on the measures too.

There seems to have been a practice, at least in India, but perhaps also further afield, according to which the solar and lunar years were both divided into 360 theoretical parts, for calculation purposes, as a circle would be divided into 360 degrees. Indeed, in the ancient Indian system, an age of Brahma is 100 years. and each year of Brahma has 360 days and 360 nights. This could be the reason for the 254 x 4, or 360 / 354.36708 ratio linking the length of the Great Giza Rectangles to the Great Pyramid perimeter, and this perimeter to the distance between the centres of the Great and third pyramids. Curiously, the distance between these two centres, 36 857.7 inches, is 8 x 13 x 354.36708, the last number being the days of a lunar year of 12 lunations. another interpretation of the perimeter of the Great Pyramid then opens up, as 354.36708² x 13 x 8 / 360 = 36 277.5190 inches.

254, 235 and 223: The Saros and Metonic Cycles

223 synodic months make up a Saros cycle or 18.029 years, and are also approximately equivalent to 242 draconic months and 19 eclipse years. The number 19 return in the Metonic cycle, but with respect to regular solar years, as 19 years are approximately equivalent to 235 lunations or synodic months, and also to 254 sidereal months. when we see the numbers 19 and 254 together, or 19 and 235, we can interpret this as an attempt to synchronise the lunar and solar years, either from the viewopint of the stars, or from earth.

The Saros and Metonic cyles are expressed in a dynamic way at Giza, as factors or ratios between elements. The Saros cycle is a period of time used for predicting eclipses. The Metonic cycle is the one that directly relates to the recurrence of the positions of the Sun and Moon in relation to the Earth. It's a period of approximately 19 years after which the phases of the Moon and the days of the year align nearly perfectly. It arises from the relationship between the synodic periods of the Sun and Moon. Like the Saros cycle, the Metonic cycle is a human construct used to synchronise lunar and solar calendars. the images below show various ways in which numbers derived from these cycles can be found at Giza.

The Great Pyramid side can be understood in terms of 223, 235 and 254.

Or 223 x 38 = 8 474 inches, 223 being the number of lunations in a Saros period (Halley) and 38 being related to the Metonic period, as 365.242199 x 38 / 470 gives approximately the number of days in a lunation.

Overview of Connections

Below are some diagrams illustrating some of the proposed connections between aspects of the Giza site, in terms of the dimensions in inches. The first one focuses on the astronomical numbers, and plays down the role of pi, Phi, and the square roots of three and five as much as possible.

The diagram below shows that often, geometric and astronomical numbers can be combined to come up with quite accurate connections.

A brief comparison to the Antikythera Mechanism

While I don't understand all the workings of the famous ancient Greek Antikythera Mechanism, with its many gears and dials, I have read various papers about it and watched videos on YouTube, and been struck by the presence of essentially the same numbers as at Giza. Here are a few screenshots from a video by Spencer Connor.

19/ 254 is approximately the ratio between a solar year and a sidereal month.

19/1016 is 19 / (254 x 4), approximately the ratio between 4 solar years and a sidereal month.

A Saros cycle can be approximated as 940 x 7 days

4 237 is 19 x 223, the number of eclipse years in a Saros season, and 223 is the number of lunations in a Saros cycle. 4 237 is also 8 474 / 2, and 8 474 is the side of the second pyramid in inches (Petrie 8 474.9 inches). The 19 eclipse years correspond to a pattern, by which solar and lunar eclipses will take place at the same node of the lunar orbit after that period. The numbers 477 and 4237 in the diagram above can also be related to the second pyramid side, as 365.242199² x 477 x 200 / (354.36708 x 4237) = 8 476.1462, which as a value in inches would be just over two inches above Petrie's estimate.

From a geometric point of view, it just so happens that Phi³ = 4.23607 is almost equal to 19 x 223 / 1 000 = 4.237. And we can obtain a nice theoretical cubit with 19 x 2 x 223 / (1.618034 x 254) = 20.618976, as a value in inches.

The mechanism has two spiral dials on the back plate indicating the Saros and Metonic cycles. We see the interplay of the Saros and Metonic cycles numbers at Giza.

Conclusion

Without written historical records, it remains speculative to make any comment about the intention of the pyramid architects. However, the data does support the hypothesis that the dimensions of the Giza pyramids were influenced by astronomical cycles, the golden ratio, pi and the square roots of 2, 3 and 5, expressed mainly in inches, but also in metres, cubits, Saxon feet, and megalithic yards, among others. The consistency of measurements across different pyramids suggests a unified design principle. The data shows clear mathematical relationships which use numbers derived from astronomical cycles. These cycles are present numerically and in inches, at other ancient sites, and so connections between them and Giza could be explored further. We should take the dimensions of ancient sites elsewhere seriously. The numbers which seem to link the various proportions at Giza are the same numbers which are expressed in the dimensions, be it in inches, metres, Saxon feet or cubits. While it remains a little controversial to read the Giza dimensions in inches, the numbers that emerge from reading the site in inches are, in various configurations, often multiples, or close to multiples of the same numbers we find in the proportions, or at least the model of the proportions suggested here. These proportions are both astronomical and geometric.

Probably, the best explanation of the use of any unit of measure at Giza is one that is going to tie in with the proportions, on the one hand, and some theme or pattern that suggests itself throughout the site that is revealed through that unit. It is possible that the theme behind the design at Giza is a mix of astronomy and geometry. In order to find it, it is helpful to forget the idea of finding integer numbers of such and such a unit expressed simply in the dimensions, such as 12 units here, 6 units there. Instead we can look for combinations of astronomical and geometric numbers.

The links between various linear dimensions is interesting. For example the link between the diagonal of the kings chamber and the diagonal of the rectangle that links the centres of the Great Pyramid and the third pyramid is a coefficient of 80. As we saw, the King's Chamber diagonal is the diagonal of a 1x 2 rectangle, expressing √5. If this is multiplied by 80, we get the diagonal of another rectangle, with a different length to width ratio, which is in fact Metonic. If we find the same numbers linking proportions as ratios a Giza as we do expressed in lengths in inches, metres, or whatever other unit, the that gives a good indication that that particular unit is indeed part of the design.

Do we need to rely on any cubit value to interpret the design of the Giza structures? There is no historical evidence that we should, and no proven historical link based on written sources between cubit rods that exist still today and the design at Giza. In fact there are no written historical sources giving an account of the design of the Great Pyramid at all that are contemporary with it. If we infer from a particular part of the site a certain cubit, for example, from the King's Chamber dimensions, which we could choose to interpret as 20 x 10 cubits, we then have to try and apply that cubit value elsewhere in the site and hope to see a pattern emerge. My experience has not been successful in that regard. Personally, I have found temporarily leaving cubits aside helpful in trying to determine any patterns in the dimensions and proportions, and allow numbers such as  π, √3, 223, 235, 254, 8, 365.25, 360, 29.53059, etc to come through.

We can read, in number, valuable information. There are probably many more instances of astronomical patterns to be found at Giza. Dennis Payne has found many, for instance.

There are also examples of astronomical connections in other sites from ancient Egypt. One example is a wall at the Saqqara necropolis, found by Jean-Philippe Lauer, and referenced by Robert Bauval in his book The Egypt Code (17). Bauval writes about a boundary wall there, with "a total of 192 recesses and protrusions, 14 false doors, four corner bastions and one main entrance. Few researchers, however, had paid much attention to the hundreds of slender horizontal panels that were also an integral part of the design. What was most intriguing about these panels was that the west side of the boundary wall contained 1,461 of them, and the east side 1459. The uncanny similarity of these values to the Sothic cycle of 1,460-1 years was obvious". Bauval does not give the dimensions but an online source gives 540 x 280 metres, which is intriguing.

It's not unusual in ancient and even medieval buildings and stone circles to find references to time cycles in the dimensions or proportions. Keith Critchlow makes the point that the sun spire at Chartres Cathedral in France is 365 feet high. And the moon spire is 28 feet less than the sun spire. (10) The 28 feet indicate a moon cycle of 28 nights. Each individual foot represents one day in a solar year.

The difference in height between the Great Pyramid and the second pyramid is 112 inches, if we take 5776 and 5664 inches, as per Flinders Petrie. Curiously, if we take out cue from Critchlow's analysis of Chartres, and divide the difference by 28, we get exactly 4 inches. Alternatively we could read the Great Pyramid height as 5773.2 inches (close to 10 000 / √3), and the difference between the two pyramid heights is then 27.3 x 4 inches. A sidereal month is 27.32166 days.

And Jim Wakefield has shown that stone circles such as the Rollrights, Stonehenge and Stanton Drew in England have diameters that can be identified as having radii of 47 units (Saxon feet for example at the Rollrights) which means that the circumference will have 10 x a lunation in days in that same units.

If the Giza proportions and dimensions are indeed based on a dynamic astronomical system, then perhaps we can also think of the units found there as linked to this system too.

Several units belonging to historical metrology can be linked, if only theoretically, to the numbers and ratios derived from Giza. A lunar number such as 28 may have given rise to a geometric and astronomical equivalence using the square root of three and pi. As we've seen 3000 x √3 / (2 π x 29.53059) = 28.004633. If we multiply 28 by 10, and divide by the square root of 2, we obtain 198.02266, which in inches is a unit of 16.5 feet, sometimes called a rod, sometimes called a perch. If we divide this further by 300 we get a shusi of 0.66 inches. Or if we divide instead by 15 we get a Saxon foot of 13.20151 inches.

Bailly writes that in ancient Indian tradition, there was a custom of dividing up both the solar and lunar year into 360 parts. We can tentatively link the English yard of 36 inches to this practice.

The proportions and dimensions of the Giza site can perhaps tell us something about an ancient astronomical practice, elements of which survived in different traditions of the ancient world. Bailly tells us:

The writer and researcher Simon Ferandou came up with the concept of astrocreation, to denote something that had been made according to principles of astronomy, in particular pertaining to the ancient world. Giza, as a site, seems to be an astrocreation, as a place defined by dynamic principles derived from a complex and sophisticated model of the workings of the universe, specifically the sun, the moon, the stars and the planets as seen from earth.

Notes

1. Bailly, Jean-Sylvain, Histoire de l'astronomie ancienne, depuis son origine jusqu'à l'établissement de l'École d'Alexandrie, p 64-65

Histoire de l'astronomie ancienne, depuis son origine jusqu'à l'établissement de l'École d'Alexandrie - Google Play Books

2. Ibid.

3. Ibid

4. Diodorus of Sicily, with an English translation by C.H. Oldfather, Book II, 47 https://archive.org/details/diodorusofsicily02dioduoft/page/38/mode/2up

5. A brief word on Flinders Petrie. Others have measured the Giza plateau. Why use a survey from 150 years ago? To a large extent it's about personal preference. Flinders Petrie's survey was precise, with values given down to a tenth of an inch, usually within a particular error margin. This is not the case in some of the more modern surveys. Also, Flinders Petrie realised from his work that the Great Pyramid and the second pyramid were not exactly orientated to the cardinal points, which he interpreted as deliberate and so orientated his survey to the position of the pyramids, not to true north. While this makes only a small difference, it's an important one. Lastly, when we compare measurements taken by Flinders Petrie to Google Earth measurements, they hold up very well.

5. Legon, John, 'The Plan of the Giza Pyramids', Archaeological Reports of the Archaeology Society of Staten Island, Vol.10 No.1. New York, 1979, Revised 2019 Academia.com https://www.academia.edu/41077254/The_Plan_of_the_Giza_Pyramids

6. Neal, John, "Murder of Hippasus", https://www.academia.edu/38290213/Murder_of_Hippasus_docx

7. From Jamblichus, Vita Pythagorica, 162 (p. 205 Deubner).

8. See Norman Wildberger's excellent video https://www.youtube.com/watch?v=REeaT2mWj6Y

9. See https://www.youtube.com/watch?v=G9DVk73OVfs

44. The Giza Great Pyramid and the Mayan Zapal by David Kenworthy

10. See here: https://www.youtube.com/watch?v=Au5uaxQZ1c0&t=2120s I haven't been able to find written evidence of these claims or any precise measurements for Chartres Cathedral.

11. Crowhurst, Howard, 2001, Carnac the Alignments, p 51

12. Heath Richard & Heath Robin, The Meaning of Le Ménec, https://www.academia.edu/5384849/The_Meaning_of_Le_Menec_A_Study_of_the_Moon_using_the_Circumpolar_Stars

13. The Aryabhatiya of Aryabhata, An Ancient Indian Work on Mathematics and Astronomy, tranlsated with notes by Walter Eugene Clark, Professor of Sanskrit in Harvard University, The University of Chicago Press, Illinois, 1929. p.8

https://ia801309.us.archive.org/35/items/The_Aryabhatiya_of_Aryabhata_Clark_1930/The_Aryabhatiya_of_Aryabhata_Clark_1930.pdf

14. Ibid.

https://ia801309.us.archive.org/35/items/The_Aryabhatiya_of_Aryabhata_Clark_1930/The_Aryabhatiya_of_Aryabhata_Clark_1930.pdf

The Aryabhatiya of Aryabhata (476) is one of the most important texts in the history of Indian mathematics and astronomy. He composed this work at the age of 23. Aryabhata wrote that the Moon and planets shone by reflected sunlight, and that the orbits of the planets were ellipses, explained the causes of eclipses of the Sun and the Moon, valued the sidereal year at 365 days 6 hours 12 minutes 30 seconds, which is only 3 minutes 20 seconds longer than the modern value of 365 days 6 hours 9 minutes 10 seconds. He also estimated the value of pi this way: "Add four to one hundred, multiply by eight and then add sixty-two thousand. The result is approximately the circumference of a circle of diameter twenty thousand. By this rule the relation of the circumference to diameter is given." In other words, Pi = 2 832 / 20 000 = 3.1416. Aryabhata also accurately gave the Earth's circumference as 24,835 miles, very close to the current estimate for the polar circumference of 24 859.73 miles. As Aryabhata was only 23, when he wrote this work, and as in any case it would have taken centuries of observations to arrive at these figures, he was certainly drawing on the work of others.

15 Ibid. p 54

16. Leplat, Quentin, 2024, LE GÉNIE DES ARPENTEURS ÉGYPTIENS, https://www.academia.edu/121533482/LE_GE_NIE_DES_ARPENTEURS_E_GYPTIENS

17. Bauval, Robert, 2006, The Egypt Code, Random House, pp 56-57

18.Plato, Republic, Plato in Twelve Volumes, Vols. 5 & 6 translated by Paul Shorey. Cambridge, MA, Harvard University Press; London, William Heinemann Ltd. 1969. https://www.perseus.tufts.edu/hopper/text?doc=Perseus%3Atext%3A1999.01.0168%3Abook%3D10%3Apage%3D617

19. Plato, Timaeus, Translation by David Horan, https://www.platonicfoundation.org/translation/platos-timaeus/

1. Plato, Phaedrus, 248, from Plato. Plato in Twelve Volumes, Vol. 9 translated by Harold N. Fowler. Cambridge, MA, Harvard University Press; London, William Heinemann Ltd. 1925. https://www.perseus.tufts.edu/hopper/text?doc=Perseus%3Atext%3A1999.01.0174%3Atext%3DPhaedrus%3Asection%3D249d