The term ‘incommensurable’ means ‘to have no common measure’ and refers to two magnitudes, or to two paradigms. The idea apparently has its origins in Ancient Greek mathematics, where it described the problem of how an irrational number such as pi or the square root of two can define the relation between two parts of a shape. No “rational number” can express the square root of 2 for example, so it is impossible to measure exactly the diagonal of a square in relation to it's side, or the number of units the side is made of. There is no common unit of measurement between the side and the diagonal. Every time you try to define the length of the diagonal in relation to aliquot parts of the side, there will always be a little bit left over. The same issue of course comes up in a circle, in a pentagon, and in many other geometrical shapes where square roots, pi or Phi define relations between lengths. There is simply no common unit of measure between a diameter and a circumference, or between the side and the length of a pentagon.

The paradox is that pi, Phi and various square roots (mainly √2, √3 and √5) are central to various units of measure, as they have been understood over time, in the field of historical metrology. Irrational numbers, which normally would scupper any chances of a reconciliation between diameter and side, or diameter and circumference, actually define relations between remen and cubit, or between foot and metre. Bizarre really! And yet this seems to be the case so often. Just one example is the circumference of a circle measuring 6 Egyptian Royal Cubits, which has a diameter of 1 ancient metre. If there is no common unit between a diameter and a circumference, for example, why on earth should two units of measure be derived from the diameter and circumference of a circle?

Ok, so that's not exactly true. It's not really actual irrationals at work here. What defines the relations between these ancient units is approximations of pi, Phi and the various square roots. So in practice, pi is sometimes 22/7, sometimes 25/8, sometimes the Rhind Papyrus fraction 256/81= of 3.160493827, or 3.141818181818, or even just plain old 3.15. And Phi is often approximated as a fraction of two consecutive Fibonacci numbers, like 55/34, or 34/21. In the same way, Phi squared, which comes up a lot in ancient metrology, can be found seemingly as 55/21, or 144/55. The square root of two is mostly found as 99/70. The apparent use of such tricks to bypass the thorny problem of irrationality has led some to deduce from this that the 'ancients' knew nothing about irrationals and hadn't figured out pi or Phi at all. Science requires evicence, and there is no evidence of irrationals before a certain point in time.... therefore humans hadn't figured them out yet. There are obvious problems with this logic, in the context of the ancient past, of which not everything that was ever written down has survived. This view also rests on a general idea that back then, maths was pretty basic, and they still had a lot of work to do. How could they possibly have understood the concept of irrationality, or been actually able to better approximate pi, when Newton hadn't even been born yet? This is despite stories of Pythagoreans throwing one poor chap overboard their boat, to his doom, as punishment for revealing the fact that the square roots of 2, 3 and 5 for example were irrational. This view also goes hand in hand with an idea that before the Greeks, people were pretty dim-witted, probably went around grunting in animal skins, and were generally pretty unsophisticated. This is despite many accounts of very sophisticated science coming from places such as India long before Plato's time, and being deduced from very old sites such as Stonehenge and Giza. Basic maths, basic humans and a lack of written evidence are the main arguments agaisnt the discovery of irrationals in the ancient world. Needless to say, there are many researchers who are convinced that science and maths had reached dizzying heights long before the Greek 'miracle', at various stages of so-called pre-history. The concept of incommensurability defines not just the task at hand in trying to piece together the way ancient measures actually worked, but also the conflicting approaches of various researchers trying to figure it all out. An incompatibility of paradigms underlies contemporary understandings of ancient geometry.

Here are some examples of units of measure found in the ancient world that seem to be linked by approximations of pi, Phi and √2. The Egyptian Royal cubit of 20.625" multiplied by 144/55 and 0.7291666667 (the Egyptian digit in inches) gives the ancient metre in inches, of 39.375". (Such an ancient metre is not universally accepted by any means, but it's art of a theory of units which includes as a main building block a 0.7291666667" digit, 54 of which make up this 39.375" unit). The 20.625" length is in turn the diagonal of a square with sides of 20 of these digits, and the ancient metre of 39.375 is the diagonal of a square with sides of 20 x 144/55 x 0.729166667 x 0.7291666667 inches, all of course with 99/70 for √2.

Here are some other examples.

So there are lots of possible links between units of measure which require the use of 22/7, 25/8, 99/70, 144/55 or 55/21 for example. Were these approximations actually used? If so, were they used because that was the best they could do, being unable to define actual pi or Phi or root 2? Or was there a purpose to this practice, which might ultimately betray a high degree of sophistication?

Let's take the example of the Great Pyramid of Giza. It's well known that the side of the pyramid is in pi ratio with the height. Or rather, two sides multiplied by 7/22 (approximately 1/pi) give the height. This would suggest that the unit or units that make up the height are themselves in 'pi' ratio with the units that constitute the base sides.

Matthew Flinders Petrie gave his estimate for the height of the Great Pyramid as 5,776 inches, and the mean base side (they are all slightly different) as 9068.8". If we modify these slightly by thre inches or so, to say 9,072" and 5,773.090909", then yes, it's perfect. All thanks to 22/7. And it turns out that what 22/7 allows is to bring in the square root of two and Phi squared by the back door. Base side and height are linked not by pi but by an approximation of pi. How could it be otherwise, since the pyramid exists in this world, with a certain material tangible height and sides, that are measurable. If both are measurable then clearly, real pi has not been used. If the base side and the height are linked by an approximation of pi, can they also be linked by approximations of other irrationals?

What becomes apparent from comparing the height and side of the Great Pyramid in a many ways as possible is that pi as 22/7 = 99/70 x 20/9, which offers a connection to the approximation for the square root of two, and also 22/7 = 55/21 x 6/5, which offers a connection between pi, root 2 and Phi squared. This last one is quite well known, and in fact the 20.625" Egyptian Royal Cubit (which is 20.618181818 multiplied by 3,025/3,024, which is the exact ratio between two phi squared approximations, 144/55 and 55/21, and also between two pi approximations 22/7 and 864/275), works out as 0.5238095238 metres, of the 39.375" kind. (This is the metre that is made up of 54 digits of 0.7291666667", the digit which times 16 makes up the Roman Foot, or times 20 the Remen, and many other units). 0.5238095238, or 11/21, is a very nice number in that it is the difference between 22/7 and 55/21, the pi and Phi squared approximations.

22/7 = 3.142857142857... and 55/21 = 2.619047604764067...

So this Egyptian Royal Cubit seems quite happily linked with approximations of pi and Phi, which in turn seem quite happily linked to each other, and to 99/70 for root 2, and to the dimensions of the Great Pyramid of Giza.

As for the height of the Great Pyramid, it also works out as a nifty 12⁴/100 x 70/99 ancient metres, and the base side, being the height x 99/70 x 10/9, works out as 12⁴/90 ancient metres. 1 ancient metre is the diagonal of a square with sides of 500 x 21/55 x 21/55 x 55/144 inches.

The perimeter of the GP, if you think of it as 3024 feet, is also 1,760 x 20.618181818 inches, so one side is (21/55)² x 99/70 x 4 x 11 x 1,000 inches. The height, being in 11/7 ratio to the base side, is then very close to (21/55)² x 99/70 x 4 x 7 x 1,000 = 5,773.090909 inches (3 inches off Petrie's value).

The Great Pyramid of Giza is just one of many examples in the ancient world of a sophisticated metrology at work, which involves approximations of √2, Phi and pi not just because that was the best the engineers and mathematicians could come up with, but because these values worked together so nicely. Indeed, it might be helpful to think of the various units and important numbers that we find in sites like the Great Pyramid as so many combinations of Phi and root 2 approximations, i.e. 55/21 and 21/55, 144/55 and 55/144, and 99/70. So: 54 as 21/55 x 99/70 x 100, or of 1134 as 21/55 x 99/70 x 2100, or 20.618181818 as 21/55 x 21/55 x 99/70 x 100, and 20.625 as 21/55 x 99/70 x 55/144 x 100, the remen as 55/144 x 21/55 x 100, and the Saxon foot of 13.125" as 55/144 x 21/55 x 90, as all the units seem to flow into each other nicely like that. The 0.729166667" digit is 21/55 x 55/144 x 1/2 = 210 / 288 = 70/96, and the English yard = 64/70 x 54 x 70/96 inches, the English foot = 70/96 x 18 x 64 / 70 = 12 inches = 13.125 x 64/70, and the English inch = 70/96 x 64/70 x 3/2 = 25/24 x 64 x 3/200.

Why should there be 54 digits in a metre? Maybe there's a link to the fact that 54 is 21/55 x 99/70 x 100, so phi squared and root 2 approximations multiplied together, and by 100?

And 39.375" / 500, which is (55/144)³ x (3024/3025)² x 99/70 inches, is also a tenth of the 0.07875" digit, or 2 millimetres. Also 2 x 54 = 108, and 108 is a really important number in various ancient traditions, notably India. It defines relations between the earth and the sun (average distance earth sun x 108 = sun diameter), and the earth and the moon (average distance earth moon x 108 = moon diameter), and many other things such as the inner angles of a pentagon in degrees.

So that's a nice way to link up metre (albeit the 39.375" one) and inch: a square with sides of (55/144)³ x (3024/3025)² inches will have a diagonal of 0.07875", which is 2 millimetres of the 39.375" metre. (multiply by 8,000/8,001 to get the modern 10,000/254 inch value)

(55/144)³ x (3024/3025)² is also 21/55 x 21/55 x 55/144, so involving the two forms of phi approximations with Fibonacci numbers, so that's maybe a better way to visualise the sides of a square, of which the diagonal is 2 mm.

And then this 2 millimetre length (or 0.07875") obtained in the square multiplied by 100 and 144/55 becomes the Egyptian Royal Cubit of 20.61818181818". This ERC multiplied by two Phi approximations, each being 55/21, gives exactly 10 x 99/70 inches. And so you could think of the inch as something that derives from the Egyptian Royal Cubit, though sometimes it seems the metre derives from the inch. The English foot can perhaps be said to derive from the metre.

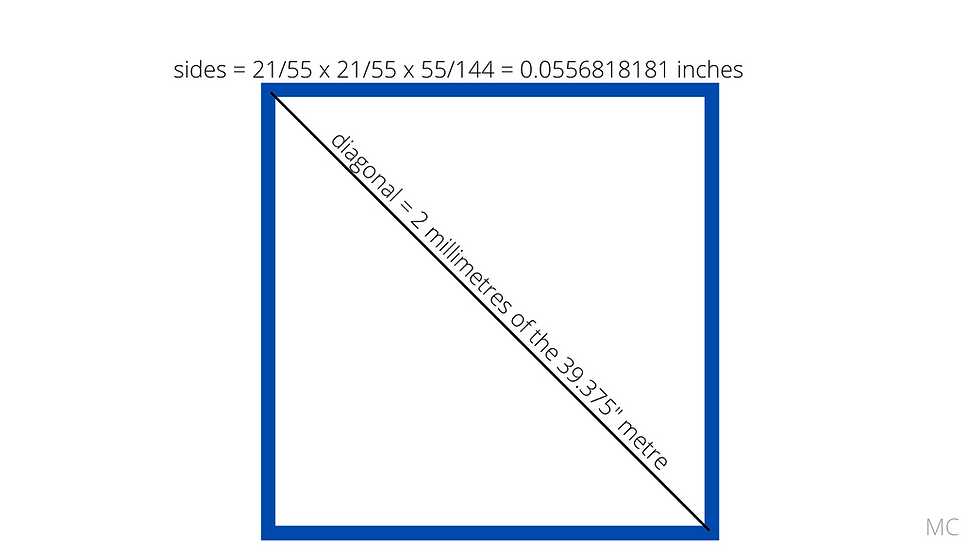
The square with sides of 21/55 x 21/55 x 55/144 = 0.0556818181 inches has a diagonal of 2 millimetres of the 39.375" metre. This diagonal is then multiplied by 100 and 144/55 to create the 20.618181818" ERC. Then this in turn is multiplied by 55/21 twice to create a length of 100 x 99/70 inches, so this length is the diagonal to a square of 100 inches.

Perhaps the incommensurability of the diagonal and sides of a square, or diameter and circumference of a circle were indeed acknowledged, but parked, and a system put in place despite this that was actually quite amazing and beautiful, where pi, Phi and √2, are deliberately approximated to become one numerical language.

Then again..., perhaps we should be using actual irrationals, not approximations, to define the relations between units. This in itself would provide an interesting way of dealing with incommensurability within say a geometric shape such as the circle or square, and allow for a high degree of precision in measurement, using one unit for one aspect of the shape, and another unit, which would be related to that first unit by an irrational number, to define the other aspects of the shape. The only approximations left then would be in the way the measure was actually marked and read... which would in the end work out the same as approximating the irrationals that link the units. But let's go with the idea for a minute. For example, to take the square from the image above, if the side of the square is 100", multiply by √2, and divide by Phi² twice, and you get an Egyptian Royal Cubit of 20.63311339", and divide again by √2, you would have a remen of 14.589814395". Taking this slightly oversized ERC again, and multiplying by 6 and dividing by pi would give a metre of 39.4063438", also a little on the big side. And though I'm using the inch as my main unit, what if the inch derived from the ERC? In which case you would have and inch of 20.63311339 x 10/2 x 1/Phi² x 6400/7 x 1/36000 = 1.00078108". It's worth looking at the possibility at any rate. Maybe it would be fun to have modern day units to measure the side of a square and another for the diagonal, or the diameter of a circle, and another for the circumference, or the side of a pentagon, and another for the length. But ultimately, we need to just accept that when we measure we approximate. Maybe Protagoras's famous phrase about Man being the measure of all things

refers to this need to approximate, because no matter what we physically measure (as opposed to theoretically), it's always going to be done in a finite way that does not allow for infinite strings of decimals or irrationals.

The square roots of three, and five, and pi in geometry and geometrical interpretations of structures also appear, such as below in this interpretation of the Great Pyramid, and of the Station Stone rectangle at Stonehenge, as well as in the construction of Phi in a Pythagorean triangle (Jim Alison coined the term "Phi-thagorean"!).

The Station Stone Rectangle is just the right height and width to fit the two spirals, as above, so that if the first side, measuring 1, is positioned along its diagonal (dark blue line), then its root 17 side (turquoise line) will meet the opposite short side at the two thirds point.