An article in a 1953 edition of the Mathematical Gazette takes the form of a dialogue between father and son. The article is authored by one "Peter Simple", obviously a pseudonym, as the name is given in inverted commas. The name is presumably an apology for stating something very easy to grasp, yet the article is enigmatic. The supposedly simple nature of the problem is further underlined by the article taking the form of a dialogue between a father and son. Yet, by making the very first words after the title a quotation by Cicero, and in Latin to boot, the author, whoever he may be, is careful not to allow the reader to dismiss his efforts as simplistic. The article deals with the ratios between three different measures, in the imperial system, invoking the geometry of the square, yet is also calls into question the terminology used in historical metrology, which can be quite confusing.
Squares
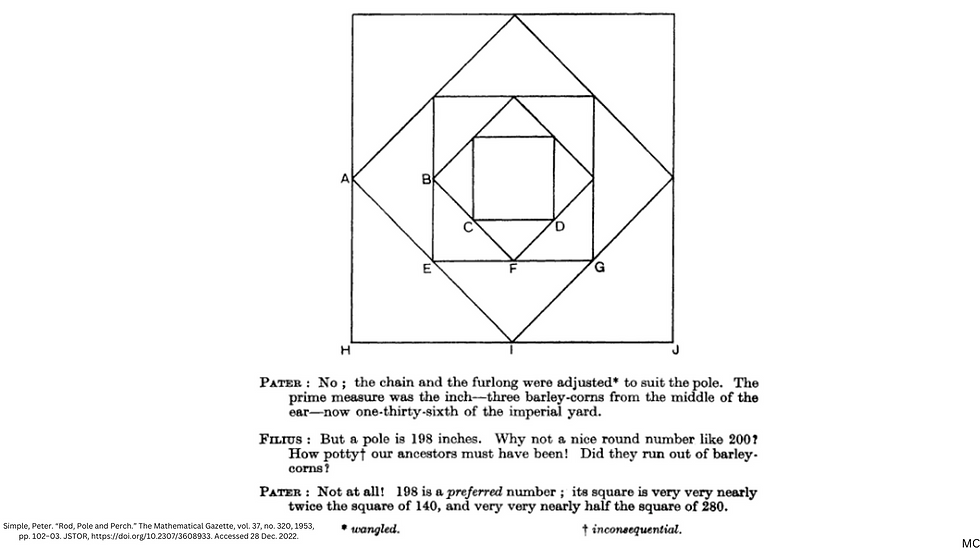
The main point the author makes in the piece is that 198 inches, which is usually given as the length of a rod, perch or pole, is not 200 inches or some other nice round number, for a good reason. It is 198 inches because this 198 the 140 x 99/70, this last fraction being a common approximation of the square root of two. The square root of two is the ratio between the side and the diagonal of a square. Furthermore, the author makes a distinction between the rod, the perch and the pole, rather than considering them as equivalents as is usually the case.
For Peter Simple, it is all about the square, and he points out that the square of 140, multiplied by 2, is 39200, and the square of 198 is 39204. So that a length of 140", which is his rod, multiplied by 99/70, gives 198", which is his pole. And the pole, multiplied by 99/70, gives (very close to) 280", which is his perch. So the pole is the diagonal of a square with sides of one rod, and the perch is the diagonal of the square with sides of one pole. Furthermore, half a rod being 70", and half a pole being 99", the very approximation of the square root of two, as 99/70, seems to be referred to.
A rod is 16.5 feet or 198 inches, or very close to 5 metres (for a perfect 5 metre - rod match, a 39.6" 'metre', i.e. 54 x 0.733333", works best). This is what is given in encyclopedias, and it is also what Peter simple gives. The author refers to a half rod as a 70" staff, "which was probably the measure of the old fathom". A fathom is usually defined as 72".
The system invoked by the author is reminiscent of the way to double the area of a square, as outlined in Plato's Meno. It is simply a matter of taking the diagonal of one square to form the side of the next biggest square.
There is another curious collection of measures connected by squares. It is Egyptian, and the square root of two ratio linking the first two was found by Flinders Petrie. It is the ratio between the remen (of 14.58" or 14.583333") and the royal cubit (of 20.61818182" or 20.62", or 20.625") The third measure in this trio linked by a square root of two was also found by Flinders Petrie, as pointed out on Jim Alison's webpage. Quoting Flinders Petrie, Jim Alison writes:
Petrie stated: “Whether the Egyptian treated the well-known plumb-line as a pendulum is not indicated by any remains, though the plumb-line was commonly in use from very early times. But the notable fact is that 29.157 inches, the diagonal of the 20.62 inch cubit, is the length which would swing 100,000 times in 24 hours, exactly true at Memphis latitude. This is so remarkable that it suggests that it may have been derived from that observed length, and the source entirely forgotten after the scientific age of the pyramid builders.” 7
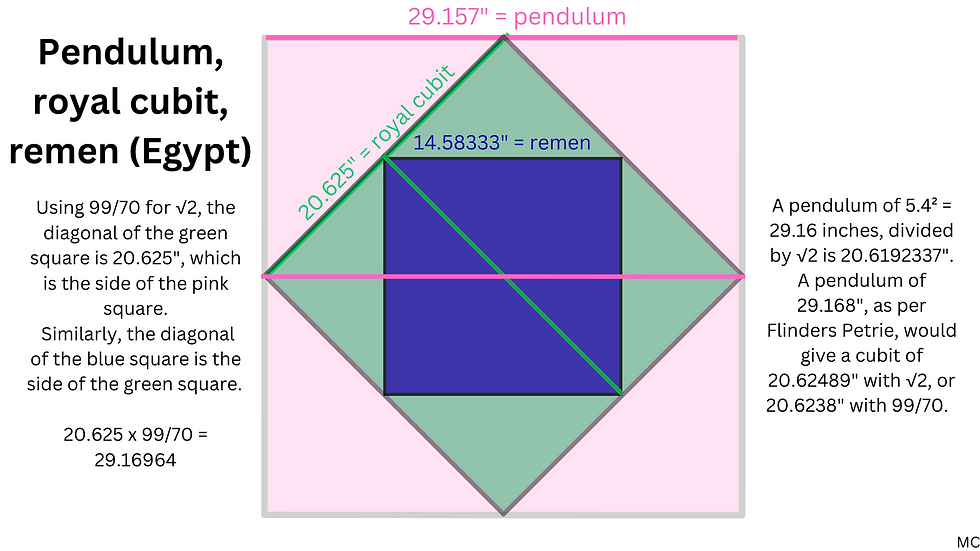
In the case of the Egyptian trio of units, plumb line, royal cubit, remen, it seems that the measure from which the others are derived is not the smallest, but the largest. This would go against the idea of a square being doubled in size and producing a series of bigger squares. this is because the largest measure corresponds to a measure of time at a precise latititude, in this case the latitude of Memphis. The other two units are the result of gradually smaller squares being produced. Mostly the rod is equated with the pole or perch, and was originally made up of 15 of the old Northern or Saxon feet, of 13.2", or 13.125". Indeed, 15 x 13.2 = 198. there are 4,800 Saxon or Northern feet in a mile.
As Frank Skinner writes:
After A.D. 410 with the departure of the Romans and the coming of the Saxons, the Northern Cubit and Foot became established in England in the Saxon kingdoms at a value of 264" for the Cubit or Ell as a cloth measure and a foot of 13'2" for building, and for land measure for their " Open Field " system of ploughlands, in which the various " holdings " were rectangular strips side by side, known as " Roods " (i acres). The sides of the Rood were always in the relation of 1 x 40, i.e. 1 Land Rod in breadth X 40 Land Rods in length, i.e. the " Furrow length " or " Furlong ". Four Roods side by side made up the " Acre ", the sides of which were thus 4 X 40 Rods. The Land Rod was 15 Saxon feet of 13-2", i.e. 16 ft. 6 ins. in our current Imperial Measure. The Furrow-length of 40 Land Rods or 600 Saxon feet was thus 660 feet or 220 yards by our current measure and is still the recognised length of the furlong, eight of which make our statute mile of 5,280 feet or 1,760 yards, later established by the Statute 35 Elizabeth, cap. 6, sect. 8 of A.D. 1593, entitled " An Act agaiste newe Buyldinge ". (1)
The article by Peter Simple alludes to an Egyptian connection. "The rod is derived from the Pharaonic qanu of 11²/³ Egyptian feet."The author gives the rod 11²/³ English feet, or 140 inches. For Peter Simple, English feet are clearly connected to, if not equated with, Egyptian feet. He goes on to write: "Whence came also the measuring reed, described by Ezekiel, of 6 great-cubits."
The author does not give a length for the reed, but assuming it is close to 140 inches, divided by 6 would give 23.3333" for the great cubit of Ezekiel. 140" / 6 = 23.3333 ". Multiplied by 6/7 to give the regular cubit, and then by 3/5 to get the foot, brings us back to 12". The reed is equated to 11²/³ Israelitic feet, just as the rod is 11²/³ English feet.
Starting with a length of 140 inches, and using the same logic, 140" / 6 = 23.3333 ". Multiplied by 6/7 this gives 20". This multiplied by 3/5 gives 12", which is an English foot. So this 11.66666 ratio for the foot and the qanu, or the Israelitic feet and the reed, is in fact simply 5/3 x 7, or 70/6, which suggests the relationship between a regular and a royal cubit, which have 6 and 7 palms respectively. So that 10 English feet multiplied by 7/6 are 140", which is Simple's rod, and 20 English feet multiplied by 7/6 are 280", Simple's Perch.
So why all these squares? It is of course to do with land measure.
In a 1955 article, Berriman writes:
Ancient linear metrology derives its primary practical significance from the special association of certain units with land measurement: for example the Egyptian setat was a land area equal to the square on 100 royal cubits and the square on this cubit was discovered by Petrie and Griffith to be twice the area of the square on a 20-digit unit called remen. Geometrically, therefore, the royal cubit can be represented by the diagonal of the square remen; mathematically its rating is 20√2 digits and the evidence suggests that this can be interpreted as 20 + (5/8) English inches on the hypothesis that √2 digit has a rational equivalent in 1 + (1/32) inch.
Thus rated the royal cubit is in (25/24) length ratio to the Sumerian cubit of 30 shusi, this sushi of 0.66 inch being the average length of division on the linear scale on Gudea’s statue in the Louvre. Hitherto it has escaped notice that this Sumerian cubit of 19.8 inches measures (1/10) English pole; similarly, the Sumerian foot of 20 shusi (=13.2 inches) is a prototype of the medieval foot that Petrie reported (on the evidence of his measurements of ancient monuments) to be the “most usual English unit” but he did not recognise it as (1/15) pole. In English metrology the pole is associated with land measurement through the 160 square pole rating of the acre but it had a simpler association with the Roman jugerum which measured 100 English sq, poles = 1 myriad Sumerian sq. cubits. The jugerum, therefore, can be represented geometrically as the square on 10 English poles = 100 Sumerian cubits.
Berriman is in agreement here with Peter Simple: an English pole is 280 inches. The setat is an area equal to the square of 100 royal cubits, which would have a diagonal length of 100 x 29.16964 inches, using 20.625 and 99/70. This is exactly 40 digits of 0.729166667" or 2 remen of 14.5833333".
The digit: √2, Phi² and the metre
Berriman mentions a hypothetical √2 digit or 1¹/³² inch unit, which multiplied by 20 gives the Egyptian royal cubit (of 20.625"), by 32 gives the 33" unit, and 16 and 12 gives 198 inches, the pole. And as the metre is related to the Egyptian royal cubit by Phi squared, this 100 of these digits of 1¹/³² inches multiplied by 55/21 for 1/Phi² is a metre (of 39.375"). And 4 such units of 1¹/³² inches multiplied by 144/55 for Phi squared give 10.8", which is the unit known as U. We can conclude that if an Egyptian royal cubit is both 20 √2 digits and 0.2 Phi² metres, and if the digit is a 54th part of a metre, then the relationship between the metre and Egyptian royal cubit is an important one.
There is a connection between the approximations 99/70 for the square root of two and 55/21 for Phi squared, in that 21/55 x 99/70 x 100 = 54, and this is the number digit in metres. And this digit multiplied by the square root of 2 and 20 gives the Egyptian royal cubit. So that is why an Egyptian royal cubit is both 0.2 Phi squared metres and 20/54 x the square root of 2 metres. I think this shows that the metre is a better base unit for the Egyptian royal cubit than the inch or foot. As the ratios between the inch and the metre have varied quite a bit over the past couple of centuries, albeit only very fractionally, I think it would be more precise to think of the digit as a unit in metres rather than inches, specifically as 55/21 x 70/99 x 1/100 metres, and then decide on a ratio to convert to inches, be it 39.375, 39.6, 39.3700787402, or other.
This is further backed up by the fact that the Egyptian royal cubit is equivalent to pi - Phi² metres.
However, Berriman clearly connects English, Roman and Sumerian units, and in particular connects the 198" pole to the Sumerian cubit. The Sumerian cubit of 19.8 inches is the Egyptian royal cubit of 20.625 inches x 24/25. We saw that an 11.66666 ratio for the foot and the qanu, or the Israelitic feet and the reed, which is in fact 70/6, and this can be used again to describe the relationship between the English foot and the Egyptian royal cubit:
20.625 x 24/25 x 70/99 x 6/7 = 12
An English foot is the Sumerian cubit of 24/25 Egyptian cubit, divided by the square root of 2, or the approximation 99/70, divided by 7 and multiplied by 6. In metres, this can be written as 20/54 x 24/25 x 6/7, or simply as 20 digits x 24/25 x 6/7.
Or 1 Roman foot x 6/7 x pi /Phi², or 1 Roman foot x 6/7 x 6/5.
Circles
Perhaps the most surprising aspect of this article is that the three main measures, the rod, the pole and the perch, are set out as different from each other. Usually, they are referred to as equivalents. In his 1911 article for Encyclopaedia Britannica, Flinders Petrie equates the three. Peter Simple supposes some sort of historical confusion as to the reason why the three may have come to mean the same thing. So, mostly the rod is equated with the pole or perch, and is thought to have originally been made up of 15 of the old Northern or Saxon feet, of 13.2", or 13.125". Indeed, 15 x 13.2 = 198. there are 4,800 Saxon or Northern feet in a mile.
This extract from an 1836 maths manual states that the rod and pole are synonyms:
As a unit of measure of area, the perch is equivalent to 1/40th of an acre. An acre is defined as 43,560 square feet. A fortieth part of this is therefore 1,089 square feet. This is the area of a square with sides 33 feet, or 396 inches, or 540 x 0.733333", or 2x198". So this does indeed seem to suggest that the perch, or at least the unit forming the side of a square, forty of which make an acre, is 2 x 198". Peter Simple names a unit of 198" a pole. However, he does not equate a perch with 396 inches, but with 280 inches. These last two measures are of course also connected by the square root of two.
another possible link is between the 198" unit, Simple's pole, and the metre, as two poles are 396 inches, which is close to ten metres. (Two perches are 540 units of 0.733333").
All in all, Peter Simple's article is a bit of an enigma. Where does he get the ratios between rod, pole and perch from, and why does Flinders Petrie not distinguish between them in his encyclopedia article?
Flinders Petrie does mention a perch different to the rod in a historical context, however:
The Gallic leuga, or league, is a different unit, being 1.59 British miles by the very concordant itinerary of the Bordeauxpilgrim. This appears to be the great Celtic measure, as opposed to the old English, or Germanic, mile. In the north-west of England and in Wales this mile lasted as 1.56 British miles till 1500; and the perch of those parts was correspondingly longer till this century (31). The " old London mile " was 5000 ft., and probably this was the mile which was modified to 5280 ft., or 8 furlongs, and so became the British statute mile.
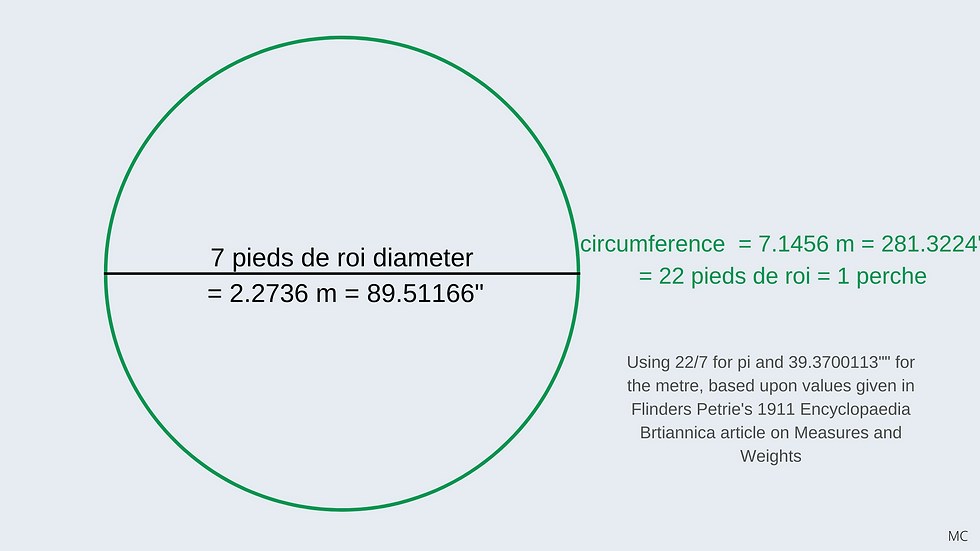
Flinders Petrie also refers to the French perche, which he gives as 22 square pieds de roi, and he gives a pied de roi 0.3248 metres (and uses a conversion rate based on a metre of 39.3700113").
This gives a French perche of (22 x 0.3248) ² = 51.059599 m², or 79,142.266 sq. in. And here we get a sense of why Peter Simple may have ascribed 280" to the English perch, as the French perche, according to these figures given by Flinders Petrie, is 281.322". Peter Simple gives the English perch 280". The use of the number 22, which is the number of pieds de roi in a French perche, suggests a pi approximation and a circle. Indeed, 22 pieds de roi divided by 7 give 40.1889 inches, and of course 280 inches, Peter Simple's preferred value for the perch, is 40 x 7 inches, and also 20 feet x 7/6. This suggests that a 280" unit is the circumference of a circle with a diameter of 89.0909 inches, using 22/7 for pi.
The French value of 281.322 inches for the perche is the circumference of a circle, of which the diameter is exactly 7 pieds de roi.
So we have moved away from the square to the circle. This would suggest that if 280" as a unit of length were derived from a circle circumference, with a diameter of 7 pieds de roi, then a unit of 40 inches would be derived from a circle with a diameter of 1 pied de roi. However, the pied de roi as given by Flinders Petrie doesn't quite match the required diameter for a 40" circumference; instead of 0.3248 m, it would need to be 0.32328 m. It does suggest a possible connection, however.
Curiously this measure of 0.32328 m is close to being 20 cm x Phi.
Using 1.6181818 for Phi and 22/7 for pi, and a 39.375" metre, 0.2 metres x pi x Phi are 40.05 inches.
This time with 144/55 for Phi squared, and a radius of 0.2 x Phi² metres, this gives a circumference of 64.8 inches, which is 1.08 x 6. A measure of 198 inches could be produced in this way: 0.2 m, multiplied by 55/21 for Phi squared, then by 22/7 for pi, then by 99/70 for the square root of two, and then by 6³/100, (with a slightly different metre of 39.37099").
Without the 99/70, the 0.2 meters multiplied by 55/21 and 22/7, and then 6³/100 give 140 inches.
20 cm multiplied by 55/21 x 22/7 x 99/70 x 39.375 x 6 x 6 / 100 gives 33.00336 inches.
Flinders Petrie gives 33 inches to the Peruvian vara. (See the 1911 article)
Using this length of 33 inches, and dividing by 11.666667, which is the number of feet in a Pharaonic qanu according to Simple, we arrive back at the square, as 33/11.666667 is close to 2 x 99/70. So the number of feet in a qanu is 33/2 x 70/99.
An Egyptian or Roman foot of 11.664 inches is also connected, as 1 metre x 144/55 x 22/7 x 36 x 39.375 / 1,000 = 11.664 inches. (Alternatively, the same but with 39.6" for the metre and 25/8 for pi, or again with 39.3752 for the metre and 55/21 for Phi squared and 3.14181818 for pi)
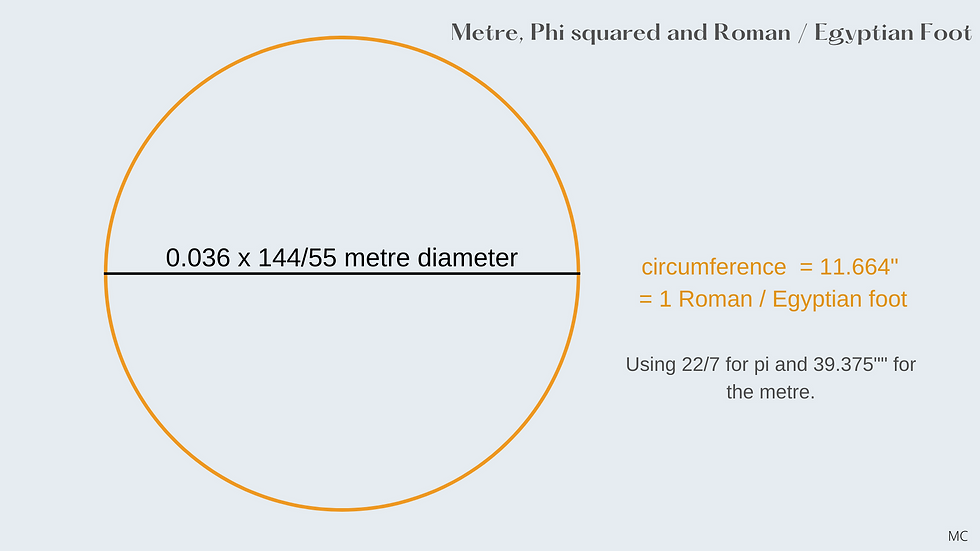
20 cm multiplied by 55/21 for Phi squared give the Egyptian royal cubit of 20.625", and if using 144/55 for Phi squared, it gives the 20.6181818" Egyptian royal cubit.
Below are some more connections centered on a circle with a diameter in metres.
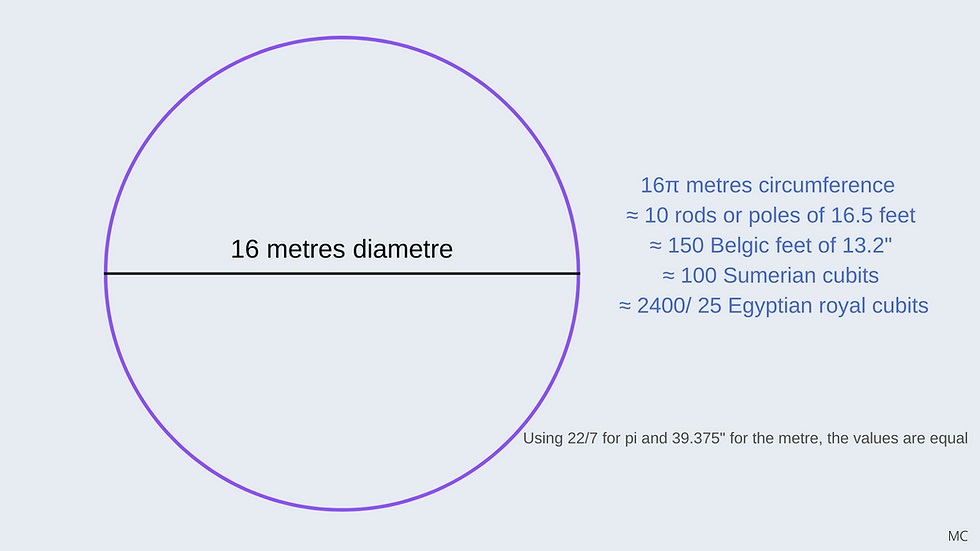
Incidentally, 280 inches is also 24 roman feet of 11.666667". Berriman says that the French pole was originally 24 Roman feet - and yes, Berriman refers to it as a pole. "which was later rated 22 pieds in the French linear scale, where 6 pieds made the toise used in the geodectic measurements that formed the basis of the metric system." So Berriman connects the 198 inch measure with 24 Roman feet and 22 pieds de roi, and indirectly all to these to the metre of 54 digits (of 0.729166667"). The discrepancy between the pied de roi as 24 Roman feet as 24 x 11.666667", and as it is recorded as 324.839mm, which is just a bit shorter and works out at 12.7889 inches with the modern metric conversion rate, is attributed to a measure of volume: " the cube of the double-pied: the actual standard muid, however, was only 286 litres which is not quite 8 times the cube of 322.5 mm."
198" is also 96/10 Egyptian royal cubits of 20.625", so there is a direct connection to the pied de roi, as there is to the pole and rod. The 70 inches alluded to by Peter Simple, described as a staff, "which was probably the measure of the old fathom" is referred to also by Berriman, who writes:
"70 inches = 96 digits = (96/54) metre = 1777.7 mm for comparison with 1778 mm = 70 x 25.4 mm."
Of course, 70/1.7777778 = 39.375, which is the other value Berriman uses.
Berriman notes is that the ratio between the French and English poles is the square root of two. So as Peter Simple suggests there is a square root of two ratio at work, but while Simple finds it between what he terms the perch, pole and rod, Berriman finds it between the English and French poles.
According to Berriman:
1 pied de roi = 324.839 mm or 24/22 Roman pedes = 323.2 mm "in terms of a 1/54 m digit" = 39.375 inches / 54 x 16 x 24/22 = 12.72727 inches, or 39.3700787402 inches / 54 x 16 x 24/22 = 12.725682. (Or alternatively again with a 0.729 inch digit)
2 pieds de roi = 1 aune
3 aunes = 1 toise = 6 pieds = 72 pouces = 864 lignes = 6 x 324.839 mm = 1949.034 or 6 x 323.2 mm = 1939.2 mm
French pole = 6 aunes = 2 toises = 12 pieds = 144 pouces = 1728 lignes
1 arpent = double-jugerum = heredium = 100 French sq poles
Approximately: One 54th part of a metre x 16 makes a Roman foot, multiplied by 24 gives 280 inches (English perch acc. to Simple) or 7.11111 m , divided by √2 gives 198 inches (English pole acc. to Simple) or 5.028 m.
Curiously, the Irish perch was slightly longer than the English, and measured not 5.5 yards but 7 yards. The Irish perch would be equal to 64 centimetres exactly, with a 39.375 inch conversion to metric.
Notes
1. F. G. Skinner. (1952). The English Yard and Pound Weight. Bulletin of the British Society for the History of Science, 1(7), 179–187. http://www.jstor.org/stable/4024847
Bibliography
Alison, Jim, Earth Measures,C:\art\R28.wpd (hiwaay.net)
BERRIMAN, A. E. “A NEW APPROACH TO THE STUDY OF ANCIENT METROLOGY.” Revue d’Assyriologie et d’archéologie Orientale, vol. 49, no. 4, 1955, pp. 193–201. JSTOR, http://www.jstor.org/stable/23295603. Accessed 8 Jan. 2023.
Flinders Petrie, Matthew, 1911, "Weights and Measures", Encyclopaedia Britannica, Weights and Measures - LoveToKnow 1911 (archive.org)
Kelly, P. 1816, METROLOGY, OR , AN EXPOSITION OF WEIGHTS AND MEASURES ,CHIEFLY THOSE OF GREAT BRITAIN AND FRANCE : COMPRISING Tables of Comparison , AND VIEWS OF VARIOUS STANDARDS ; WITH AN ACCOUNT OF LAWS AND LOCAL CUSTOMS , Parliamentary Reports ,London
Olinthus, Gregory, 1836, Mathematics for practical men: being a common-place book of principles, theorems, rules, and tables, in various departments of pure and mixed mathematics, with their application; especially to the pursuits of surveyors, architects, mechanics, and civil engineers, Philadelphia, E. L. Carey and A. Hart
Simple, Peter. “Rod, Pole and Perch.” The Mathematical Gazette, vol. 37, no. 320, 1953, pp. 102–03. JSTOR, https://doi.org/10.2307/3608933. Accessed 18 Jul. 2022.
F. G. Skinner. (1952). The English Yard and Pound Weight. Bulletin of the British Society for the History of Science, 1(7), 179–187. http://www.jstor.org/stable/4024847